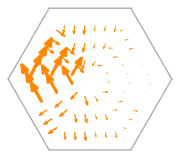
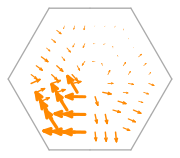
an encyclopedia of finite element definitions
Degree 1 rotated Buffa–Christiansen on a dual polygon
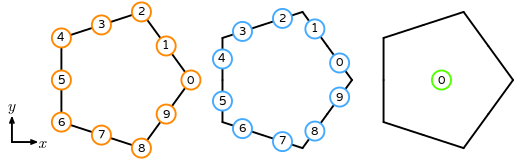
◀ Back to rotated Buffa–Christiansen definition page
- \(R\) is the reference dual polygon. The following numbering of the subentities of the reference is used:
- Basis functions:
\(\displaystyle \boldsymbol{\phi}_{0} = \begin{cases}
\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{5}{12}\\\displaystyle \tfrac{\sqrt{3} \left(- 4 x - 15\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (1, 0), (3/4, sqrt(3)/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{1}{4}\\\displaystyle \tfrac{\sqrt{3} \left(7 - 4 x\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (3/4, sqrt(3)/4), (1/2, sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{1}{6}\\\displaystyle \tfrac{\sqrt{3} \left(2 - x\right)}{9}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (1/2, sqrt(3)/2), (0, sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{1}{6}\\\displaystyle \tfrac{\sqrt{3} \left(1 - x\right)}{9}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (0, sqrt(3)/2), (-1/2, sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{1}{4}\\\displaystyle \tfrac{\sqrt{3} \left(- 4 x - 1\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (-1/2, sqrt(3)/2), (-3/4, sqrt(3)/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{1}{12}\\\displaystyle \tfrac{\sqrt{3} \left(- 4 x - 3\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (-3/4, sqrt(3)/4), (-1, 0)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{1}{12}\\\displaystyle \tfrac{\sqrt{3} \left(- 4 x - 3\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (-1, 0), (-3/4, -sqrt(3)/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{1}{4}\\\displaystyle \tfrac{\sqrt{3} \left(- 4 x - 1\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (-3/4, -sqrt(3)/4), (-1/2, -sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{1}{6}\\\displaystyle \tfrac{\sqrt{3} \left(1 - x\right)}{9}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (-1/2, -sqrt(3)/2), (0, -sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{1}{6}\\\displaystyle \tfrac{\sqrt{3} \left(2 - x\right)}{9}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (0, -sqrt(3)/2), (1/2, -sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{1}{4}\\\displaystyle \tfrac{\sqrt{3} \left(7 - 4 x\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (1/2, -sqrt(3)/2), (3/4, -sqrt(3)/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{5}{12}\\\displaystyle \tfrac{\sqrt{3} \left(- 4 x - 15\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (3/4, -sqrt(3)/4), (1, 0)))\end{cases}\)
\(\displaystyle \boldsymbol{\phi}_{1} = \begin{cases}
\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{5}{12}\\\displaystyle \tfrac{\sqrt{3} \left(- 4 x - 1\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (1, 0), (3/4, sqrt(3)/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{5}{12}\\\displaystyle \tfrac{\sqrt{3} \left(- 4 x - 15\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (3/4, sqrt(3)/4), (1/2, sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{5}{6}\\\displaystyle - \tfrac{\sqrt{3} x}{9}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (1/2, sqrt(3)/2), (0, sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{1}{6}\\\displaystyle \tfrac{\sqrt{3} \left(2 - x\right)}{9}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (0, sqrt(3)/2), (-1/2, sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{5}{12}\\\displaystyle \tfrac{\sqrt{3} \left(1 - 4 x\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (-1/2, sqrt(3)/2), (-3/4, sqrt(3)/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{1}{4}\\\displaystyle \tfrac{\sqrt{3} \left(- 4 x - 1\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (-3/4, sqrt(3)/4), (-1, 0)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{1}{12}\\\displaystyle \tfrac{\sqrt{3} \left(- 4 x - 5\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (-1, 0), (-3/4, -sqrt(3)/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{1}{12}\\\displaystyle \tfrac{\sqrt{3} \left(- 4 x - 3\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (-3/4, -sqrt(3)/4), (-1/2, -sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{1}{6}\\\displaystyle - \tfrac{\sqrt{3} x}{9}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (-1/2, -sqrt(3)/2), (0, -sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{1}{6}\\\displaystyle \tfrac{\sqrt{3} \left(1 - x\right)}{9}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (0, -sqrt(3)/2), (1/2, -sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{1}{12}\\\displaystyle \tfrac{\sqrt{3} \left(5 - 4 x\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (1/2, -sqrt(3)/2), (3/4, -sqrt(3)/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{1}{4}\\\displaystyle \tfrac{\sqrt{3} \left(7 - 4 x\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (3/4, -sqrt(3)/4), (1, 0)))\end{cases}\)
\(\displaystyle \boldsymbol{\phi}_{2} = \begin{cases}
\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{1}{4}\\\displaystyle \tfrac{\sqrt{3} \left(1 - 4 x\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (1, 0), (3/4, sqrt(3)/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{5}{12}\\\displaystyle \tfrac{\sqrt{3} \left(- 4 x - 1\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (3/4, sqrt(3)/4), (1/2, sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{1}{6}\\\displaystyle \tfrac{\sqrt{3} \left(- x - 2\right)}{9}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (1/2, sqrt(3)/2), (0, sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{5}{6}\\\displaystyle - \tfrac{\sqrt{3} x}{9}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (0, sqrt(3)/2), (-1/2, sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{5}{12}\\\displaystyle \tfrac{\sqrt{3} \left(15 - 4 x\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (-1/2, sqrt(3)/2), (-3/4, sqrt(3)/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{5}{12}\\\displaystyle \tfrac{\sqrt{3} \left(1 - 4 x\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (-3/4, sqrt(3)/4), (-1, 0)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{1}{4}\\\displaystyle \tfrac{\sqrt{3} \left(- 4 x - 7\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (-1, 0), (-3/4, -sqrt(3)/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{1}{12}\\\displaystyle \tfrac{\sqrt{3} \left(- 4 x - 5\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (-3/4, -sqrt(3)/4), (-1/2, -sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{1}{6}\\\displaystyle \tfrac{\sqrt{3} \left(- x - 1\right)}{9}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (-1/2, -sqrt(3)/2), (0, -sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{1}{6}\\\displaystyle - \tfrac{\sqrt{3} x}{9}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (0, -sqrt(3)/2), (1/2, -sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{1}{12}\\\displaystyle \tfrac{\sqrt{3} \left(3 - 4 x\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (1/2, -sqrt(3)/2), (3/4, -sqrt(3)/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{1}{12}\\\displaystyle \tfrac{\sqrt{3} \left(5 - 4 x\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (3/4, -sqrt(3)/4), (1, 0)))\end{cases}\)
\(\displaystyle \boldsymbol{\phi}_{3} = \begin{cases}
\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{1}{12}\\\displaystyle \tfrac{\sqrt{3} \left(3 - 4 x\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (1, 0), (3/4, sqrt(3)/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{1}{4}\\\displaystyle \tfrac{\sqrt{3} \left(1 - 4 x\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (3/4, sqrt(3)/4), (1/2, sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{1}{6}\\\displaystyle \tfrac{\sqrt{3} \left(- x - 1\right)}{9}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (1/2, sqrt(3)/2), (0, sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{1}{6}\\\displaystyle \tfrac{\sqrt{3} \left(- x - 2\right)}{9}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (0, sqrt(3)/2), (-1/2, sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{1}{4}\\\displaystyle \tfrac{\sqrt{3} \left(- 4 x - 7\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (-1/2, sqrt(3)/2), (-3/4, sqrt(3)/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{5}{12}\\\displaystyle \tfrac{\sqrt{3} \left(15 - 4 x\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (-3/4, sqrt(3)/4), (-1, 0)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{5}{12}\\\displaystyle \tfrac{\sqrt{3} \left(15 - 4 x\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (-1, 0), (-3/4, -sqrt(3)/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{1}{4}\\\displaystyle \tfrac{\sqrt{3} \left(- 4 x - 7\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (-3/4, -sqrt(3)/4), (-1/2, -sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{1}{6}\\\displaystyle \tfrac{\sqrt{3} \left(- x - 2\right)}{9}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (-1/2, -sqrt(3)/2), (0, -sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{1}{6}\\\displaystyle \tfrac{\sqrt{3} \left(- x - 1\right)}{9}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (0, -sqrt(3)/2), (1/2, -sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{1}{4}\\\displaystyle \tfrac{\sqrt{3} \left(1 - 4 x\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (1/2, -sqrt(3)/2), (3/4, -sqrt(3)/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{1}{12}\\\displaystyle \tfrac{\sqrt{3} \left(3 - 4 x\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (3/4, -sqrt(3)/4), (1, 0)))\end{cases}\)
\(\displaystyle \boldsymbol{\phi}_{4} = \begin{cases}
\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{1}{12}\\\displaystyle \tfrac{\sqrt{3} \left(5 - 4 x\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (1, 0), (3/4, sqrt(3)/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{1}{12}\\\displaystyle \tfrac{\sqrt{3} \left(3 - 4 x\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (3/4, sqrt(3)/4), (1/2, sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{1}{6}\\\displaystyle - \tfrac{\sqrt{3} x}{9}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (1/2, sqrt(3)/2), (0, sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{1}{6}\\\displaystyle \tfrac{\sqrt{3} \left(- x - 1\right)}{9}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (0, sqrt(3)/2), (-1/2, sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{1}{12}\\\displaystyle \tfrac{\sqrt{3} \left(- 4 x - 5\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (-1/2, sqrt(3)/2), (-3/4, sqrt(3)/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{1}{4}\\\displaystyle \tfrac{\sqrt{3} \left(- 4 x - 7\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (-3/4, sqrt(3)/4), (-1, 0)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{5}{12}\\\displaystyle \tfrac{\sqrt{3} \left(1 - 4 x\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (-1, 0), (-3/4, -sqrt(3)/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{5}{12}\\\displaystyle \tfrac{\sqrt{3} \left(15 - 4 x\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (-3/4, -sqrt(3)/4), (-1/2, -sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{5}{6}\\\displaystyle - \tfrac{\sqrt{3} x}{9}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (-1/2, -sqrt(3)/2), (0, -sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{1}{6}\\\displaystyle \tfrac{\sqrt{3} \left(- x - 2\right)}{9}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (0, -sqrt(3)/2), (1/2, -sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{5}{12}\\\displaystyle \tfrac{\sqrt{3} \left(- 4 x - 1\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (1/2, -sqrt(3)/2), (3/4, -sqrt(3)/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{1}{4}\\\displaystyle \tfrac{\sqrt{3} \left(1 - 4 x\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (3/4, -sqrt(3)/4), (1, 0)))\end{cases}\)
\(\displaystyle \boldsymbol{\phi}_{5} = \begin{cases}
\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{1}{4}\\\displaystyle \tfrac{\sqrt{3} \left(7 - 4 x\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (1, 0), (3/4, sqrt(3)/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{1}{12}\\\displaystyle \tfrac{\sqrt{3} \left(5 - 4 x\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (3/4, sqrt(3)/4), (1/2, sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{1}{6}\\\displaystyle \tfrac{\sqrt{3} \left(1 - x\right)}{9}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (1/2, sqrt(3)/2), (0, sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{1}{6}\\\displaystyle - \tfrac{\sqrt{3} x}{9}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (0, sqrt(3)/2), (-1/2, sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{1}{12}\\\displaystyle \tfrac{\sqrt{3} \left(- 4 x - 3\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (-1/2, sqrt(3)/2), (-3/4, sqrt(3)/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{1}{12}\\\displaystyle \tfrac{\sqrt{3} \left(- 4 x - 5\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (-3/4, sqrt(3)/4), (-1, 0)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{1}{4}\\\displaystyle \tfrac{\sqrt{3} \left(- 4 x - 1\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (-1, 0), (-3/4, -sqrt(3)/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{5}{12}\\\displaystyle \tfrac{\sqrt{3} \left(1 - 4 x\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (-3/4, -sqrt(3)/4), (-1/2, -sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{1}{6}\\\displaystyle \tfrac{\sqrt{3} \left(2 - x\right)}{9}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (-1/2, -sqrt(3)/2), (0, -sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{5}{6}\\\displaystyle - \tfrac{\sqrt{3} x}{9}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (0, -sqrt(3)/2), (1/2, -sqrt(3)/2)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} - \tfrac{5}{12}\\\displaystyle \tfrac{\sqrt{3} \left(- 4 x - 15\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (1/2, -sqrt(3)/2), (3/4, -sqrt(3)/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y}{9} + \tfrac{5}{12}\\\displaystyle \tfrac{\sqrt{3} \left(- 4 x - 1\right)}{36}\end{array}\right)&\text{in }\operatorname{Triangle}(((0, 0), (3/4, -sqrt(3)/4), (1, 0)))\end{cases}\)