an encyclopedia of finite element definitions
Degree 3 Tiniest tensor H(curl) on a quadrilateral
◀ Back to Tiniest tensor H(curl) definition page
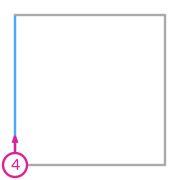
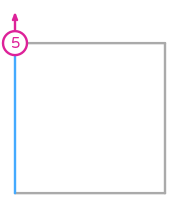
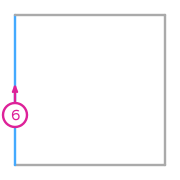
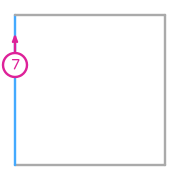
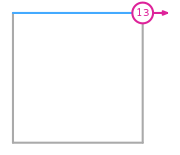
- \(R\) is the reference quadrilateral. The following numbering of the subentities of the reference cell is used:
- \(\mathcal{V}\) is spanned by: \(\left(\begin{array}{c}\displaystyle 1\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle 1\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle y\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle y\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle y^{2}\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle y^{2}\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle y^{3}\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle y^{3}\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle x\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle x\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle x y\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle x y\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle x y^{2}\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle x y^{2}\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle x y^{3}\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle x y^{3}\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle x^{2}\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle x^{2}\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle x^{2} y\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle x^{2} y\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle x^{2} y^{2}\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle x^{2} y^{2}\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle x^{2} y^{3}\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle x^{2} y^{3}\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle x^{3}\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle x^{3}\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle x^{3} y\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle x^{3} y\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle x^{3} y^{2}\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle x^{3} y^{2}\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle x^{3} y^{3}\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle x^{3} y^{3}\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle \frac{7 y \left(- 5 y^{3} + 10 y^{2} - 6 y + 1\right)}{3}\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle \frac{7 x \left(5 x^{3} - 10 x^{2} + 6 x - 1\right)}{3}\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle \frac{7 y \left(100 x^{3} y^{3} - 200 x^{3} y^{2} + 120 x^{3} y - 20 x^{3} - 150 x^{2} y^{3} + 300 x^{2} y^{2} - 180 x^{2} y + 30 x^{2} + 60 x y^{3} - 120 x y^{2} + 72 x y - 12 x - 5 y^{3} + 10 y^{2} - 6 y + 1\right)}{3}\\\displaystyle \frac{7 x \left(- 100 x^{3} y^{3} + 150 x^{3} y^{2} - 60 x^{3} y + 5 x^{3} + 200 x^{2} y^{3} - 300 x^{2} y^{2} + 120 x^{2} y - 10 x^{2} - 120 x y^{3} + 180 x y^{2} - 72 x y + 6 x + 20 y^{3} - 30 y^{2} + 12 y - 1\right)}{3}\end{array}\right)\)
- \(\mathcal{L}=\{l_0,...,l_{34}\}\)
- Functionals and basis functions:
\(\displaystyle l_{0}:\boldsymbol{v}\mapsto\displaystyle\int_{e_{0}}\boldsymbol{v}\cdot(- \frac{9 s_{0}^{3}}{2} + 9 s_{0}^{2} - \frac{11 s_{0}}{2} + 1)\hat{\boldsymbol{t}}_{0}\)
where \(e_{0}\) is the 0th edge;
\(\hat{\boldsymbol{t}}_{0}\) is the tangent to edge 0;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{0}\).
\(\displaystyle \boldsymbol{\phi}_{0} = \left(\begin{array}{c}\displaystyle - 2450 x^{3} y^{4} + 6300 x^{3} y^{3} - 5460 x^{3} y^{2} + 1750 x^{3} y - 140 x^{3} + 3675 x^{2} y^{4} - 9705 x^{2} y^{3} + \frac{112455 x^{2} y^{2}}{13} - \frac{37185 x^{2} y}{13} + 240 x^{2} - 1470 x y^{4} + \frac{53190 x y^{3}}{13} - \frac{49890 x y^{2}}{13} + \frac{17370 x y}{13} - 120 x + \frac{315 y^{4}}{2} - \frac{6055 y^{3}}{13} + \frac{5985 y^{2}}{13} - \frac{4371 y}{26} + 16\\\displaystyle \frac{5 x \left(12740 x^{3} y^{3} - 19110 x^{3} y^{2} + 7644 x^{3} y - 637 x^{3} - 32760 x^{2} y^{3} + 49374 x^{2} y^{2} - 19932 x^{2} y + 1698 x^{2} + 28392 x y^{3} - 42966 x y^{2} + 17484 x y - 1518 x - 8372 y^{3} + 12702 y^{2} - 5196 y + 457\right)}{26}\end{array}\right)\)
This DOF is associated with edge 0 of the reference cell.
where \(e_{0}\) is the 0th edge;
\(\hat{\boldsymbol{t}}_{0}\) is the tangent to edge 0;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{0}\).
\(\displaystyle \boldsymbol{\phi}_{0} = \left(\begin{array}{c}\displaystyle - 2450 x^{3} y^{4} + 6300 x^{3} y^{3} - 5460 x^{3} y^{2} + 1750 x^{3} y - 140 x^{3} + 3675 x^{2} y^{4} - 9705 x^{2} y^{3} + \frac{112455 x^{2} y^{2}}{13} - \frac{37185 x^{2} y}{13} + 240 x^{2} - 1470 x y^{4} + \frac{53190 x y^{3}}{13} - \frac{49890 x y^{2}}{13} + \frac{17370 x y}{13} - 120 x + \frac{315 y^{4}}{2} - \frac{6055 y^{3}}{13} + \frac{5985 y^{2}}{13} - \frac{4371 y}{26} + 16\\\displaystyle \frac{5 x \left(12740 x^{3} y^{3} - 19110 x^{3} y^{2} + 7644 x^{3} y - 637 x^{3} - 32760 x^{2} y^{3} + 49374 x^{2} y^{2} - 19932 x^{2} y + 1698 x^{2} + 28392 x y^{3} - 42966 x y^{2} + 17484 x y - 1518 x - 8372 y^{3} + 12702 y^{2} - 5196 y + 457\right)}{26}\end{array}\right)\)
This DOF is associated with edge 0 of the reference cell.
\(\displaystyle l_{1}:\boldsymbol{v}\mapsto\displaystyle\int_{e_{0}}\boldsymbol{v}\cdot(\frac{s_{0} \left(9 s_{0}^{2} - 9 s_{0} + 2\right)}{2})\hat{\boldsymbol{t}}_{0}\)
where \(e_{0}\) is the 0th edge;
\(\hat{\boldsymbol{t}}_{0}\) is the tangent to edge 0;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{0}\).
\(\displaystyle \boldsymbol{\phi}_{1} = \left(\begin{array}{c}\displaystyle 2450 x^{3} y^{4} - 6300 x^{3} y^{3} + 5460 x^{3} y^{2} - 1750 x^{3} y + 140 x^{3} - 3675 x^{2} y^{4} + 9195 x^{2} y^{3} - \frac{100485 x^{2} y^{2}}{13} + \frac{31065 x^{2} y}{13} - 180 x^{2} + 1470 x y^{4} - \frac{46560 x y^{3}}{13} + \frac{37920 x y^{2}}{13} - \frac{11250 x y}{13} + 60 x - \frac{175 y^{4}}{2} + \frac{2870 y^{3}}{13} - \frac{2430 y^{2}}{13} + \frac{1499 y}{26} - 4\\\displaystyle \frac{5 x \left(- 12740 x^{3} y^{3} + 19110 x^{3} y^{2} - 7644 x^{3} y + 637 x^{3} + 18200 x^{2} y^{3} - 27066 x^{2} y^{2} + 10644 x^{2} y - 850 x^{2} - 6552 x y^{3} + 9504 x y^{2} - 3552 x y + 246 x + 1092 y^{3} - 1548 y^{2} + 552 y - 33\right)}{26}\end{array}\right)\)
This DOF is associated with edge 0 of the reference cell.
where \(e_{0}\) is the 0th edge;
\(\hat{\boldsymbol{t}}_{0}\) is the tangent to edge 0;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{0}\).
\(\displaystyle \boldsymbol{\phi}_{1} = \left(\begin{array}{c}\displaystyle 2450 x^{3} y^{4} - 6300 x^{3} y^{3} + 5460 x^{3} y^{2} - 1750 x^{3} y + 140 x^{3} - 3675 x^{2} y^{4} + 9195 x^{2} y^{3} - \frac{100485 x^{2} y^{2}}{13} + \frac{31065 x^{2} y}{13} - 180 x^{2} + 1470 x y^{4} - \frac{46560 x y^{3}}{13} + \frac{37920 x y^{2}}{13} - \frac{11250 x y}{13} + 60 x - \frac{175 y^{4}}{2} + \frac{2870 y^{3}}{13} - \frac{2430 y^{2}}{13} + \frac{1499 y}{26} - 4\\\displaystyle \frac{5 x \left(- 12740 x^{3} y^{3} + 19110 x^{3} y^{2} - 7644 x^{3} y + 637 x^{3} + 18200 x^{2} y^{3} - 27066 x^{2} y^{2} + 10644 x^{2} y - 850 x^{2} - 6552 x y^{3} + 9504 x y^{2} - 3552 x y + 246 x + 1092 y^{3} - 1548 y^{2} + 552 y - 33\right)}{26}\end{array}\right)\)
This DOF is associated with edge 0 of the reference cell.
\(\displaystyle l_{2}:\boldsymbol{v}\mapsto\displaystyle\int_{e_{0}}\boldsymbol{v}\cdot(\frac{9 s_{0} \left(3 s_{0}^{2} - 5 s_{0} + 2\right)}{2})\hat{\boldsymbol{t}}_{0}\)
where \(e_{0}\) is the 0th edge;
\(\hat{\boldsymbol{t}}_{0}\) is the tangent to edge 0;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{0}\).
\(\displaystyle \boldsymbol{\phi}_{2} = \left(\begin{array}{c}\displaystyle \frac{26950 x^{3} y^{4}}{27} - \frac{7700 x^{3} y^{3}}{3} + \frac{20020 x^{3} y^{2}}{9} - \frac{19250 x^{3} y}{27} + \frac{1540 x^{3}}{27} - \frac{13475 x^{2} y^{4}}{9} + 3935 x^{2} y^{3} - \frac{136115 x^{2} y^{2}}{39} + \frac{134305 x^{2} y}{117} - \frac{860 x^{2}}{9} + \frac{5390 x y^{4}}{9} - \frac{20880 x y^{3}}{13} + \frac{56720 x y^{2}}{39} - \frac{57250 x y}{117} + \frac{380 x}{9} - \frac{805 y^{4}}{54} + \frac{690 y^{3}}{13} - \frac{7010 y^{2}}{117} + \frac{17033 y}{702} - \frac{68}{27}\\\displaystyle \frac{5 x \left(- 140140 x^{3} y^{3} + 210210 x^{3} y^{2} - 84084 x^{3} y + 7007 x^{3} + 345800 x^{2} y^{3} - 520806 x^{2} y^{2} + 209964 x^{2} y - 17830 x^{2} - 246792 x y^{3} + 373104 x y^{2} - 151488 x y + 13074 x + 41132 y^{3} - 62508 y^{2} + 25608 y - 2251\right)}{702}\end{array}\right)\)
This DOF is associated with edge 0 of the reference cell.
where \(e_{0}\) is the 0th edge;
\(\hat{\boldsymbol{t}}_{0}\) is the tangent to edge 0;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{0}\).
\(\displaystyle \boldsymbol{\phi}_{2} = \left(\begin{array}{c}\displaystyle \frac{26950 x^{3} y^{4}}{27} - \frac{7700 x^{3} y^{3}}{3} + \frac{20020 x^{3} y^{2}}{9} - \frac{19250 x^{3} y}{27} + \frac{1540 x^{3}}{27} - \frac{13475 x^{2} y^{4}}{9} + 3935 x^{2} y^{3} - \frac{136115 x^{2} y^{2}}{39} + \frac{134305 x^{2} y}{117} - \frac{860 x^{2}}{9} + \frac{5390 x y^{4}}{9} - \frac{20880 x y^{3}}{13} + \frac{56720 x y^{2}}{39} - \frac{57250 x y}{117} + \frac{380 x}{9} - \frac{805 y^{4}}{54} + \frac{690 y^{3}}{13} - \frac{7010 y^{2}}{117} + \frac{17033 y}{702} - \frac{68}{27}\\\displaystyle \frac{5 x \left(- 140140 x^{3} y^{3} + 210210 x^{3} y^{2} - 84084 x^{3} y + 7007 x^{3} + 345800 x^{2} y^{3} - 520806 x^{2} y^{2} + 209964 x^{2} y - 17830 x^{2} - 246792 x y^{3} + 373104 x y^{2} - 151488 x y + 13074 x + 41132 y^{3} - 62508 y^{2} + 25608 y - 2251\right)}{702}\end{array}\right)\)
This DOF is associated with edge 0 of the reference cell.
\(\displaystyle l_{3}:\boldsymbol{v}\mapsto\displaystyle\int_{e_{0}}\boldsymbol{v}\cdot(\frac{9 s_{0} \left(- 3 s_{0}^{2} + 4 s_{0} - 1\right)}{2})\hat{\boldsymbol{t}}_{0}\)
where \(e_{0}\) is the 0th edge;
\(\hat{\boldsymbol{t}}_{0}\) is the tangent to edge 0;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{0}\).
\(\displaystyle \boldsymbol{\phi}_{3} = \left(\begin{array}{c}\displaystyle - \frac{26950 x^{3} y^{4}}{27} + \frac{7700 x^{3} y^{3}}{3} - \frac{20020 x^{3} y^{2}}{9} + \frac{19250 x^{3} y}{27} - \frac{1540 x^{3}}{27} + \frac{13475 x^{2} y^{4}}{9} - 3765 x^{2} y^{3} + \frac{124145 x^{2} y^{2}}{39} - \frac{115945 x^{2} y}{117} + \frac{680 x^{2}}{9} - \frac{5390 x y^{4}}{9} + \frac{18670 x y^{3}}{13} - \frac{44750 x y^{2}}{39} + \frac{38890 x y}{117} - \frac{200 x}{9} + \frac{4585 y^{4}}{54} - \frac{7205 y^{3}}{39} + \frac{15065 y^{2}}{117} - \frac{21137 y}{702} + \frac{32}{27}\\\displaystyle \frac{5 x \left(140140 x^{3} y^{3} - 210210 x^{3} y^{2} + 84084 x^{3} y - 7007 x^{3} - 214760 x^{2} y^{3} + 320034 x^{2} y^{2} - 126372 x^{2} y + 10198 x^{2} + 50232 x y^{3} - 71946 x y^{2} + 26100 x y - 1626 x + 24388 y^{3} - 37878 y^{2} + 16188 y - 1565\right)}{702}\end{array}\right)\)
This DOF is associated with edge 0 of the reference cell.
where \(e_{0}\) is the 0th edge;
\(\hat{\boldsymbol{t}}_{0}\) is the tangent to edge 0;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{0}\).
\(\displaystyle \boldsymbol{\phi}_{3} = \left(\begin{array}{c}\displaystyle - \frac{26950 x^{3} y^{4}}{27} + \frac{7700 x^{3} y^{3}}{3} - \frac{20020 x^{3} y^{2}}{9} + \frac{19250 x^{3} y}{27} - \frac{1540 x^{3}}{27} + \frac{13475 x^{2} y^{4}}{9} - 3765 x^{2} y^{3} + \frac{124145 x^{2} y^{2}}{39} - \frac{115945 x^{2} y}{117} + \frac{680 x^{2}}{9} - \frac{5390 x y^{4}}{9} + \frac{18670 x y^{3}}{13} - \frac{44750 x y^{2}}{39} + \frac{38890 x y}{117} - \frac{200 x}{9} + \frac{4585 y^{4}}{54} - \frac{7205 y^{3}}{39} + \frac{15065 y^{2}}{117} - \frac{21137 y}{702} + \frac{32}{27}\\\displaystyle \frac{5 x \left(140140 x^{3} y^{3} - 210210 x^{3} y^{2} + 84084 x^{3} y - 7007 x^{3} - 214760 x^{2} y^{3} + 320034 x^{2} y^{2} - 126372 x^{2} y + 10198 x^{2} + 50232 x y^{3} - 71946 x y^{2} + 26100 x y - 1626 x + 24388 y^{3} - 37878 y^{2} + 16188 y - 1565\right)}{702}\end{array}\right)\)
This DOF is associated with edge 0 of the reference cell.
\(\displaystyle l_{4}:\boldsymbol{v}\mapsto\displaystyle\int_{e_{1}}\boldsymbol{v}\cdot(- \frac{9 s_{0}^{3}}{2} + 9 s_{0}^{2} - \frac{11 s_{0}}{2} + 1)\hat{\boldsymbol{t}}_{1}\)
where \(e_{1}\) is the 1st edge;
\(\hat{\boldsymbol{t}}_{1}\) is the tangent to edge 1;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{1}\).
\(\displaystyle \boldsymbol{\phi}_{4} = \left(\begin{array}{c}\displaystyle \frac{5 y \left(12740 x^{3} y^{3} - 32760 x^{3} y^{2} + 28392 x^{3} y - 8372 x^{3} - 19110 x^{2} y^{3} + 49374 x^{2} y^{2} - 42966 x^{2} y + 12702 x^{2} + 7644 x y^{3} - 19932 x y^{2} + 17484 x y - 5196 x - 637 y^{3} + 1698 y^{2} - 1518 y + 457\right)}{26}\\\displaystyle - 2450 x^{4} y^{3} + 3675 x^{4} y^{2} - 1470 x^{4} y + \frac{315 x^{4}}{2} + 6300 x^{3} y^{3} - 9705 x^{3} y^{2} + \frac{53190 x^{3} y}{13} - \frac{6055 x^{3}}{13} - 5460 x^{2} y^{3} + \frac{112455 x^{2} y^{2}}{13} - \frac{49890 x^{2} y}{13} + \frac{5985 x^{2}}{13} + 1750 x y^{3} - \frac{37185 x y^{2}}{13} + \frac{17370 x y}{13} - \frac{4371 x}{26} - 140 y^{3} + 240 y^{2} - 120 y + 16\end{array}\right)\)
This DOF is associated with edge 1 of the reference cell.
where \(e_{1}\) is the 1st edge;
\(\hat{\boldsymbol{t}}_{1}\) is the tangent to edge 1;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{1}\).
\(\displaystyle \boldsymbol{\phi}_{4} = \left(\begin{array}{c}\displaystyle \frac{5 y \left(12740 x^{3} y^{3} - 32760 x^{3} y^{2} + 28392 x^{3} y - 8372 x^{3} - 19110 x^{2} y^{3} + 49374 x^{2} y^{2} - 42966 x^{2} y + 12702 x^{2} + 7644 x y^{3} - 19932 x y^{2} + 17484 x y - 5196 x - 637 y^{3} + 1698 y^{2} - 1518 y + 457\right)}{26}\\\displaystyle - 2450 x^{4} y^{3} + 3675 x^{4} y^{2} - 1470 x^{4} y + \frac{315 x^{4}}{2} + 6300 x^{3} y^{3} - 9705 x^{3} y^{2} + \frac{53190 x^{3} y}{13} - \frac{6055 x^{3}}{13} - 5460 x^{2} y^{3} + \frac{112455 x^{2} y^{2}}{13} - \frac{49890 x^{2} y}{13} + \frac{5985 x^{2}}{13} + 1750 x y^{3} - \frac{37185 x y^{2}}{13} + \frac{17370 x y}{13} - \frac{4371 x}{26} - 140 y^{3} + 240 y^{2} - 120 y + 16\end{array}\right)\)
This DOF is associated with edge 1 of the reference cell.
\(\displaystyle l_{5}:\boldsymbol{v}\mapsto\displaystyle\int_{e_{1}}\boldsymbol{v}\cdot(\frac{s_{0} \left(9 s_{0}^{2} - 9 s_{0} + 2\right)}{2})\hat{\boldsymbol{t}}_{1}\)
where \(e_{1}\) is the 1st edge;
\(\hat{\boldsymbol{t}}_{1}\) is the tangent to edge 1;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{1}\).
\(\displaystyle \boldsymbol{\phi}_{5} = \left(\begin{array}{c}\displaystyle \frac{5 y \left(- 12740 x^{3} y^{3} + 18200 x^{3} y^{2} - 6552 x^{3} y + 1092 x^{3} + 19110 x^{2} y^{3} - 27066 x^{2} y^{2} + 9504 x^{2} y - 1548 x^{2} - 7644 x y^{3} + 10644 x y^{2} - 3552 x y + 552 x + 637 y^{3} - 850 y^{2} + 246 y - 33\right)}{26}\\\displaystyle 2450 x^{4} y^{3} - 3675 x^{4} y^{2} + 1470 x^{4} y - \frac{175 x^{4}}{2} - 6300 x^{3} y^{3} + 9195 x^{3} y^{2} - \frac{46560 x^{3} y}{13} + \frac{2870 x^{3}}{13} + 5460 x^{2} y^{3} - \frac{100485 x^{2} y^{2}}{13} + \frac{37920 x^{2} y}{13} - \frac{2430 x^{2}}{13} - 1750 x y^{3} + \frac{31065 x y^{2}}{13} - \frac{11250 x y}{13} + \frac{1499 x}{26} + 140 y^{3} - 180 y^{2} + 60 y - 4\end{array}\right)\)
This DOF is associated with edge 1 of the reference cell.
where \(e_{1}\) is the 1st edge;
\(\hat{\boldsymbol{t}}_{1}\) is the tangent to edge 1;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{1}\).
\(\displaystyle \boldsymbol{\phi}_{5} = \left(\begin{array}{c}\displaystyle \frac{5 y \left(- 12740 x^{3} y^{3} + 18200 x^{3} y^{2} - 6552 x^{3} y + 1092 x^{3} + 19110 x^{2} y^{3} - 27066 x^{2} y^{2} + 9504 x^{2} y - 1548 x^{2} - 7644 x y^{3} + 10644 x y^{2} - 3552 x y + 552 x + 637 y^{3} - 850 y^{2} + 246 y - 33\right)}{26}\\\displaystyle 2450 x^{4} y^{3} - 3675 x^{4} y^{2} + 1470 x^{4} y - \frac{175 x^{4}}{2} - 6300 x^{3} y^{3} + 9195 x^{3} y^{2} - \frac{46560 x^{3} y}{13} + \frac{2870 x^{3}}{13} + 5460 x^{2} y^{3} - \frac{100485 x^{2} y^{2}}{13} + \frac{37920 x^{2} y}{13} - \frac{2430 x^{2}}{13} - 1750 x y^{3} + \frac{31065 x y^{2}}{13} - \frac{11250 x y}{13} + \frac{1499 x}{26} + 140 y^{3} - 180 y^{2} + 60 y - 4\end{array}\right)\)
This DOF is associated with edge 1 of the reference cell.
\(\displaystyle l_{6}:\boldsymbol{v}\mapsto\displaystyle\int_{e_{1}}\boldsymbol{v}\cdot(\frac{9 s_{0} \left(3 s_{0}^{2} - 5 s_{0} + 2\right)}{2})\hat{\boldsymbol{t}}_{1}\)
where \(e_{1}\) is the 1st edge;
\(\hat{\boldsymbol{t}}_{1}\) is the tangent to edge 1;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{1}\).
\(\displaystyle \boldsymbol{\phi}_{6} = \left(\begin{array}{c}\displaystyle \frac{5 y \left(- 140140 x^{3} y^{3} + 345800 x^{3} y^{2} - 246792 x^{3} y + 41132 x^{3} + 210210 x^{2} y^{3} - 520806 x^{2} y^{2} + 373104 x^{2} y - 62508 x^{2} - 84084 x y^{3} + 209964 x y^{2} - 151488 x y + 25608 x + 7007 y^{3} - 17830 y^{2} + 13074 y - 2251\right)}{702}\\\displaystyle \frac{26950 x^{4} y^{3}}{27} - \frac{13475 x^{4} y^{2}}{9} + \frac{5390 x^{4} y}{9} - \frac{805 x^{4}}{54} - \frac{7700 x^{3} y^{3}}{3} + 3935 x^{3} y^{2} - \frac{20880 x^{3} y}{13} + \frac{690 x^{3}}{13} + \frac{20020 x^{2} y^{3}}{9} - \frac{136115 x^{2} y^{2}}{39} + \frac{56720 x^{2} y}{39} - \frac{7010 x^{2}}{117} - \frac{19250 x y^{3}}{27} + \frac{134305 x y^{2}}{117} - \frac{57250 x y}{117} + \frac{17033 x}{702} + \frac{1540 y^{3}}{27} - \frac{860 y^{2}}{9} + \frac{380 y}{9} - \frac{68}{27}\end{array}\right)\)
This DOF is associated with edge 1 of the reference cell.
where \(e_{1}\) is the 1st edge;
\(\hat{\boldsymbol{t}}_{1}\) is the tangent to edge 1;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{1}\).
\(\displaystyle \boldsymbol{\phi}_{6} = \left(\begin{array}{c}\displaystyle \frac{5 y \left(- 140140 x^{3} y^{3} + 345800 x^{3} y^{2} - 246792 x^{3} y + 41132 x^{3} + 210210 x^{2} y^{3} - 520806 x^{2} y^{2} + 373104 x^{2} y - 62508 x^{2} - 84084 x y^{3} + 209964 x y^{2} - 151488 x y + 25608 x + 7007 y^{3} - 17830 y^{2} + 13074 y - 2251\right)}{702}\\\displaystyle \frac{26950 x^{4} y^{3}}{27} - \frac{13475 x^{4} y^{2}}{9} + \frac{5390 x^{4} y}{9} - \frac{805 x^{4}}{54} - \frac{7700 x^{3} y^{3}}{3} + 3935 x^{3} y^{2} - \frac{20880 x^{3} y}{13} + \frac{690 x^{3}}{13} + \frac{20020 x^{2} y^{3}}{9} - \frac{136115 x^{2} y^{2}}{39} + \frac{56720 x^{2} y}{39} - \frac{7010 x^{2}}{117} - \frac{19250 x y^{3}}{27} + \frac{134305 x y^{2}}{117} - \frac{57250 x y}{117} + \frac{17033 x}{702} + \frac{1540 y^{3}}{27} - \frac{860 y^{2}}{9} + \frac{380 y}{9} - \frac{68}{27}\end{array}\right)\)
This DOF is associated with edge 1 of the reference cell.
\(\displaystyle l_{7}:\boldsymbol{v}\mapsto\displaystyle\int_{e_{1}}\boldsymbol{v}\cdot(\frac{9 s_{0} \left(- 3 s_{0}^{2} + 4 s_{0} - 1\right)}{2})\hat{\boldsymbol{t}}_{1}\)
where \(e_{1}\) is the 1st edge;
\(\hat{\boldsymbol{t}}_{1}\) is the tangent to edge 1;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{1}\).
\(\displaystyle \boldsymbol{\phi}_{7} = \left(\begin{array}{c}\displaystyle \frac{5 y \left(140140 x^{3} y^{3} - 214760 x^{3} y^{2} + 50232 x^{3} y + 24388 x^{3} - 210210 x^{2} y^{3} + 320034 x^{2} y^{2} - 71946 x^{2} y - 37878 x^{2} + 84084 x y^{3} - 126372 x y^{2} + 26100 x y + 16188 x - 7007 y^{3} + 10198 y^{2} - 1626 y - 1565\right)}{702}\\\displaystyle - \frac{26950 x^{4} y^{3}}{27} + \frac{13475 x^{4} y^{2}}{9} - \frac{5390 x^{4} y}{9} + \frac{4585 x^{4}}{54} + \frac{7700 x^{3} y^{3}}{3} - 3765 x^{3} y^{2} + \frac{18670 x^{3} y}{13} - \frac{7205 x^{3}}{39} - \frac{20020 x^{2} y^{3}}{9} + \frac{124145 x^{2} y^{2}}{39} - \frac{44750 x^{2} y}{39} + \frac{15065 x^{2}}{117} + \frac{19250 x y^{3}}{27} - \frac{115945 x y^{2}}{117} + \frac{38890 x y}{117} - \frac{21137 x}{702} - \frac{1540 y^{3}}{27} + \frac{680 y^{2}}{9} - \frac{200 y}{9} + \frac{32}{27}\end{array}\right)\)
This DOF is associated with edge 1 of the reference cell.
where \(e_{1}\) is the 1st edge;
\(\hat{\boldsymbol{t}}_{1}\) is the tangent to edge 1;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{1}\).
\(\displaystyle \boldsymbol{\phi}_{7} = \left(\begin{array}{c}\displaystyle \frac{5 y \left(140140 x^{3} y^{3} - 214760 x^{3} y^{2} + 50232 x^{3} y + 24388 x^{3} - 210210 x^{2} y^{3} + 320034 x^{2} y^{2} - 71946 x^{2} y - 37878 x^{2} + 84084 x y^{3} - 126372 x y^{2} + 26100 x y + 16188 x - 7007 y^{3} + 10198 y^{2} - 1626 y - 1565\right)}{702}\\\displaystyle - \frac{26950 x^{4} y^{3}}{27} + \frac{13475 x^{4} y^{2}}{9} - \frac{5390 x^{4} y}{9} + \frac{4585 x^{4}}{54} + \frac{7700 x^{3} y^{3}}{3} - 3765 x^{3} y^{2} + \frac{18670 x^{3} y}{13} - \frac{7205 x^{3}}{39} - \frac{20020 x^{2} y^{3}}{9} + \frac{124145 x^{2} y^{2}}{39} - \frac{44750 x^{2} y}{39} + \frac{15065 x^{2}}{117} + \frac{19250 x y^{3}}{27} - \frac{115945 x y^{2}}{117} + \frac{38890 x y}{117} - \frac{21137 x}{702} - \frac{1540 y^{3}}{27} + \frac{680 y^{2}}{9} - \frac{200 y}{9} + \frac{32}{27}\end{array}\right)\)
This DOF is associated with edge 1 of the reference cell.
\(\displaystyle l_{8}:\boldsymbol{v}\mapsto\displaystyle\int_{e_{2}}\boldsymbol{v}\cdot(- \frac{9 s_{0}^{3}}{2} + 9 s_{0}^{2} - \frac{11 s_{0}}{2} + 1)\hat{\boldsymbol{t}}_{2}\)
where \(e_{2}\) is the 2nd edge;
\(\hat{\boldsymbol{t}}_{2}\) is the tangent to edge 2;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{2}\).
\(\displaystyle \boldsymbol{\phi}_{8} = \left(\begin{array}{c}\displaystyle \frac{5 y \left(12740 x^{3} y^{3} - 32760 x^{3} y^{2} + 28392 x^{3} y - 8372 x^{3} - 19110 x^{2} y^{3} + 48906 x^{2} y^{2} - 42210 x^{2} y + 12414 x^{2} + 7644 x y^{3} - 19464 x y^{2} + 16728 x y - 4908 x - 637 y^{3} + 1620 y^{2} - 1392 y + 409\right)}{26}\\\displaystyle \frac{x \left(- 63700 x^{3} y^{3} + 95550 x^{3} y^{2} - 38220 x^{3} y + 4095 x^{3} + 91000 x^{2} y^{3} - 129870 x^{2} y^{2} + 46500 x^{2} y - 4270 x^{2} - 32760 x y^{3} + 41220 x y^{2} - 9960 x y + 210 x + 1820 y^{3} - 660 y^{2} - 1440 y + 381\right)}{26}\end{array}\right)\)
This DOF is associated with edge 2 of the reference cell.
where \(e_{2}\) is the 2nd edge;
\(\hat{\boldsymbol{t}}_{2}\) is the tangent to edge 2;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{2}\).
\(\displaystyle \boldsymbol{\phi}_{8} = \left(\begin{array}{c}\displaystyle \frac{5 y \left(12740 x^{3} y^{3} - 32760 x^{3} y^{2} + 28392 x^{3} y - 8372 x^{3} - 19110 x^{2} y^{3} + 48906 x^{2} y^{2} - 42210 x^{2} y + 12414 x^{2} + 7644 x y^{3} - 19464 x y^{2} + 16728 x y - 4908 x - 637 y^{3} + 1620 y^{2} - 1392 y + 409\right)}{26}\\\displaystyle \frac{x \left(- 63700 x^{3} y^{3} + 95550 x^{3} y^{2} - 38220 x^{3} y + 4095 x^{3} + 91000 x^{2} y^{3} - 129870 x^{2} y^{2} + 46500 x^{2} y - 4270 x^{2} - 32760 x y^{3} + 41220 x y^{2} - 9960 x y + 210 x + 1820 y^{3} - 660 y^{2} - 1440 y + 381\right)}{26}\end{array}\right)\)
This DOF is associated with edge 2 of the reference cell.
\(\displaystyle l_{9}:\boldsymbol{v}\mapsto\displaystyle\int_{e_{2}}\boldsymbol{v}\cdot(\frac{s_{0} \left(9 s_{0}^{2} - 9 s_{0} + 2\right)}{2})\hat{\boldsymbol{t}}_{2}\)
where \(e_{2}\) is the 2nd edge;
\(\hat{\boldsymbol{t}}_{2}\) is the tangent to edge 2;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{2}\).
\(\displaystyle \boldsymbol{\phi}_{9} = \left(\begin{array}{c}\displaystyle \frac{5 y \left(- 12740 x^{3} y^{3} + 18200 x^{3} y^{2} - 6552 x^{3} y + 1092 x^{3} + 19110 x^{2} y^{3} - 27534 x^{2} y^{2} + 10152 x^{2} y - 1728 x^{2} - 7644 x y^{3} + 11112 x y^{2} - 4200 x y + 732 x + 637 y^{3} - 928 y^{2} + 354 y - 63\right)}{26}\\\displaystyle \frac{x \left(63700 x^{3} y^{3} - 95550 x^{3} y^{2} + 38220 x^{3} y - 2275 x^{3} - 91000 x^{2} y^{3} + 143130 x^{2} y^{2} - 59760 x^{2} y + 3360 x^{2} + 32760 x y^{3} - 57060 x y^{2} + 25800 x y - 1290 x - 1820 y^{3} + 4800 y^{2} - 2700 y + 101\right)}{26}\end{array}\right)\)
This DOF is associated with edge 2 of the reference cell.
where \(e_{2}\) is the 2nd edge;
\(\hat{\boldsymbol{t}}_{2}\) is the tangent to edge 2;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{2}\).
\(\displaystyle \boldsymbol{\phi}_{9} = \left(\begin{array}{c}\displaystyle \frac{5 y \left(- 12740 x^{3} y^{3} + 18200 x^{3} y^{2} - 6552 x^{3} y + 1092 x^{3} + 19110 x^{2} y^{3} - 27534 x^{2} y^{2} + 10152 x^{2} y - 1728 x^{2} - 7644 x y^{3} + 11112 x y^{2} - 4200 x y + 732 x + 637 y^{3} - 928 y^{2} + 354 y - 63\right)}{26}\\\displaystyle \frac{x \left(63700 x^{3} y^{3} - 95550 x^{3} y^{2} + 38220 x^{3} y - 2275 x^{3} - 91000 x^{2} y^{3} + 143130 x^{2} y^{2} - 59760 x^{2} y + 3360 x^{2} + 32760 x y^{3} - 57060 x y^{2} + 25800 x y - 1290 x - 1820 y^{3} + 4800 y^{2} - 2700 y + 101\right)}{26}\end{array}\right)\)
This DOF is associated with edge 2 of the reference cell.
\(\displaystyle l_{10}:\boldsymbol{v}\mapsto\displaystyle\int_{e_{2}}\boldsymbol{v}\cdot(\frac{9 s_{0} \left(3 s_{0}^{2} - 5 s_{0} + 2\right)}{2})\hat{\boldsymbol{t}}_{2}\)
where \(e_{2}\) is the 2nd edge;
\(\hat{\boldsymbol{t}}_{2}\) is the tangent to edge 2;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{2}\).
\(\displaystyle \boldsymbol{\phi}_{10} = \left(\begin{array}{c}\displaystyle \frac{5 y \left(- 140140 x^{3} y^{3} + 345800 x^{3} y^{2} - 246792 x^{3} y + 41132 x^{3} + 210210 x^{2} y^{3} - 516594 x^{2} y^{2} + 367272 x^{2} y - 60888 x^{2} - 84084 x y^{3} + 205752 x y^{2} - 145656 x y + 23988 x + 7007 y^{3} - 17128 y^{2} + 12102 y - 1981\right)}{702}\\\displaystyle \frac{x \left(700700 x^{3} y^{3} - 1051050 x^{3} y^{2} + 420420 x^{3} y - 10465 x^{3} - 1001000 x^{2} y^{3} + 1441830 x^{2} y^{2} - 554160 x^{2} y + 4600 x^{2} + 360360 x y^{3} - 469260 x y^{2} + 160920 x y + 6930 x - 20020 y^{3} + 11400 y^{2} + 2460 y - 2833\right)}{702}\end{array}\right)\)
This DOF is associated with edge 2 of the reference cell.
where \(e_{2}\) is the 2nd edge;
\(\hat{\boldsymbol{t}}_{2}\) is the tangent to edge 2;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{2}\).
\(\displaystyle \boldsymbol{\phi}_{10} = \left(\begin{array}{c}\displaystyle \frac{5 y \left(- 140140 x^{3} y^{3} + 345800 x^{3} y^{2} - 246792 x^{3} y + 41132 x^{3} + 210210 x^{2} y^{3} - 516594 x^{2} y^{2} + 367272 x^{2} y - 60888 x^{2} - 84084 x y^{3} + 205752 x y^{2} - 145656 x y + 23988 x + 7007 y^{3} - 17128 y^{2} + 12102 y - 1981\right)}{702}\\\displaystyle \frac{x \left(700700 x^{3} y^{3} - 1051050 x^{3} y^{2} + 420420 x^{3} y - 10465 x^{3} - 1001000 x^{2} y^{3} + 1441830 x^{2} y^{2} - 554160 x^{2} y + 4600 x^{2} + 360360 x y^{3} - 469260 x y^{2} + 160920 x y + 6930 x - 20020 y^{3} + 11400 y^{2} + 2460 y - 2833\right)}{702}\end{array}\right)\)
This DOF is associated with edge 2 of the reference cell.
\(\displaystyle l_{11}:\boldsymbol{v}\mapsto\displaystyle\int_{e_{2}}\boldsymbol{v}\cdot(\frac{9 s_{0} \left(- 3 s_{0}^{2} + 4 s_{0} - 1\right)}{2})\hat{\boldsymbol{t}}_{2}\)
where \(e_{2}\) is the 2nd edge;
\(\hat{\boldsymbol{t}}_{2}\) is the tangent to edge 2;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{2}\).
\(\displaystyle \boldsymbol{\phi}_{11} = \left(\begin{array}{c}\displaystyle \frac{5 y \left(140140 x^{3} y^{3} - 214760 x^{3} y^{2} + 50232 x^{3} y + 24388 x^{3} - 210210 x^{2} y^{3} + 324246 x^{2} y^{2} - 78750 x^{2} y - 35286 x^{2} + 84084 x y^{3} - 130584 x y^{2} + 32904 x y + 13596 x - 7007 y^{3} + 10900 y^{2} - 2760 y - 1133\right)}{702}\\\displaystyle \frac{x \left(- 700700 x^{3} y^{3} + 1051050 x^{3} y^{2} - 420420 x^{3} y + 59605 x^{3} + 1001000 x^{2} y^{3} - 1561170 x^{2} y^{2} + 673500 x^{2} y - 108730 x^{2} - 360360 x y^{3} + 611820 x y^{2} - 303480 x y + 58950 x + 20020 y^{3} - 48660 y^{2} + 34800 y - 8993\right)}{702}\end{array}\right)\)
This DOF is associated with edge 2 of the reference cell.
where \(e_{2}\) is the 2nd edge;
\(\hat{\boldsymbol{t}}_{2}\) is the tangent to edge 2;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{2}\).
\(\displaystyle \boldsymbol{\phi}_{11} = \left(\begin{array}{c}\displaystyle \frac{5 y \left(140140 x^{3} y^{3} - 214760 x^{3} y^{2} + 50232 x^{3} y + 24388 x^{3} - 210210 x^{2} y^{3} + 324246 x^{2} y^{2} - 78750 x^{2} y - 35286 x^{2} + 84084 x y^{3} - 130584 x y^{2} + 32904 x y + 13596 x - 7007 y^{3} + 10900 y^{2} - 2760 y - 1133\right)}{702}\\\displaystyle \frac{x \left(- 700700 x^{3} y^{3} + 1051050 x^{3} y^{2} - 420420 x^{3} y + 59605 x^{3} + 1001000 x^{2} y^{3} - 1561170 x^{2} y^{2} + 673500 x^{2} y - 108730 x^{2} - 360360 x y^{3} + 611820 x y^{2} - 303480 x y + 58950 x + 20020 y^{3} - 48660 y^{2} + 34800 y - 8993\right)}{702}\end{array}\right)\)
This DOF is associated with edge 2 of the reference cell.
\(\displaystyle l_{12}:\boldsymbol{v}\mapsto\displaystyle\int_{e_{3}}\boldsymbol{v}\cdot(- \frac{9 s_{0}^{3}}{2} + 9 s_{0}^{2} - \frac{11 s_{0}}{2} + 1)\hat{\boldsymbol{t}}_{3}\)
where \(e_{3}\) is the 3rd edge;
\(\hat{\boldsymbol{t}}_{3}\) is the tangent to edge 3;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{3}\).
\(\displaystyle \boldsymbol{\phi}_{12} = \left(\begin{array}{c}\displaystyle \frac{y \left(- 63700 x^{3} y^{3} + 91000 x^{3} y^{2} - 32760 x^{3} y + 1820 x^{3} + 95550 x^{2} y^{3} - 129870 x^{2} y^{2} + 41220 x^{2} y - 660 x^{2} - 38220 x y^{3} + 46500 x y^{2} - 9960 x y - 1440 x + 4095 y^{3} - 4270 y^{2} + 210 y + 381\right)}{26}\\\displaystyle \frac{5 x \left(12740 x^{3} y^{3} - 19110 x^{3} y^{2} + 7644 x^{3} y - 637 x^{3} - 32760 x^{2} y^{3} + 48906 x^{2} y^{2} - 19464 x^{2} y + 1620 x^{2} + 28392 x y^{3} - 42210 x y^{2} + 16728 x y - 1392 x - 8372 y^{3} + 12414 y^{2} - 4908 y + 409\right)}{26}\end{array}\right)\)
This DOF is associated with edge 3 of the reference cell.
where \(e_{3}\) is the 3rd edge;
\(\hat{\boldsymbol{t}}_{3}\) is the tangent to edge 3;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{3}\).
\(\displaystyle \boldsymbol{\phi}_{12} = \left(\begin{array}{c}\displaystyle \frac{y \left(- 63700 x^{3} y^{3} + 91000 x^{3} y^{2} - 32760 x^{3} y + 1820 x^{3} + 95550 x^{2} y^{3} - 129870 x^{2} y^{2} + 41220 x^{2} y - 660 x^{2} - 38220 x y^{3} + 46500 x y^{2} - 9960 x y - 1440 x + 4095 y^{3} - 4270 y^{2} + 210 y + 381\right)}{26}\\\displaystyle \frac{5 x \left(12740 x^{3} y^{3} - 19110 x^{3} y^{2} + 7644 x^{3} y - 637 x^{3} - 32760 x^{2} y^{3} + 48906 x^{2} y^{2} - 19464 x^{2} y + 1620 x^{2} + 28392 x y^{3} - 42210 x y^{2} + 16728 x y - 1392 x - 8372 y^{3} + 12414 y^{2} - 4908 y + 409\right)}{26}\end{array}\right)\)
This DOF is associated with edge 3 of the reference cell.
\(\displaystyle l_{13}:\boldsymbol{v}\mapsto\displaystyle\int_{e_{3}}\boldsymbol{v}\cdot(\frac{s_{0} \left(9 s_{0}^{2} - 9 s_{0} + 2\right)}{2})\hat{\boldsymbol{t}}_{3}\)
where \(e_{3}\) is the 3rd edge;
\(\hat{\boldsymbol{t}}_{3}\) is the tangent to edge 3;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{3}\).
\(\displaystyle \boldsymbol{\phi}_{13} = \left(\begin{array}{c}\displaystyle \frac{y \left(63700 x^{3} y^{3} - 91000 x^{3} y^{2} + 32760 x^{3} y - 1820 x^{3} - 95550 x^{2} y^{3} + 143130 x^{2} y^{2} - 57060 x^{2} y + 4800 x^{2} + 38220 x y^{3} - 59760 x y^{2} + 25800 x y - 2700 x - 2275 y^{3} + 3360 y^{2} - 1290 y + 101\right)}{26}\\\displaystyle \frac{5 x \left(- 12740 x^{3} y^{3} + 19110 x^{3} y^{2} - 7644 x^{3} y + 637 x^{3} + 18200 x^{2} y^{3} - 27534 x^{2} y^{2} + 11112 x^{2} y - 928 x^{2} - 6552 x y^{3} + 10152 x y^{2} - 4200 x y + 354 x + 1092 y^{3} - 1728 y^{2} + 732 y - 63\right)}{26}\end{array}\right)\)
This DOF is associated with edge 3 of the reference cell.
where \(e_{3}\) is the 3rd edge;
\(\hat{\boldsymbol{t}}_{3}\) is the tangent to edge 3;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{3}\).
\(\displaystyle \boldsymbol{\phi}_{13} = \left(\begin{array}{c}\displaystyle \frac{y \left(63700 x^{3} y^{3} - 91000 x^{3} y^{2} + 32760 x^{3} y - 1820 x^{3} - 95550 x^{2} y^{3} + 143130 x^{2} y^{2} - 57060 x^{2} y + 4800 x^{2} + 38220 x y^{3} - 59760 x y^{2} + 25800 x y - 2700 x - 2275 y^{3} + 3360 y^{2} - 1290 y + 101\right)}{26}\\\displaystyle \frac{5 x \left(- 12740 x^{3} y^{3} + 19110 x^{3} y^{2} - 7644 x^{3} y + 637 x^{3} + 18200 x^{2} y^{3} - 27534 x^{2} y^{2} + 11112 x^{2} y - 928 x^{2} - 6552 x y^{3} + 10152 x y^{2} - 4200 x y + 354 x + 1092 y^{3} - 1728 y^{2} + 732 y - 63\right)}{26}\end{array}\right)\)
This DOF is associated with edge 3 of the reference cell.
\(\displaystyle l_{14}:\boldsymbol{v}\mapsto\displaystyle\int_{e_{3}}\boldsymbol{v}\cdot(\frac{9 s_{0} \left(3 s_{0}^{2} - 5 s_{0} + 2\right)}{2})\hat{\boldsymbol{t}}_{3}\)
where \(e_{3}\) is the 3rd edge;
\(\hat{\boldsymbol{t}}_{3}\) is the tangent to edge 3;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{3}\).
\(\displaystyle \boldsymbol{\phi}_{14} = \left(\begin{array}{c}\displaystyle \frac{y \left(700700 x^{3} y^{3} - 1001000 x^{3} y^{2} + 360360 x^{3} y - 20020 x^{3} - 1051050 x^{2} y^{3} + 1441830 x^{2} y^{2} - 469260 x^{2} y + 11400 x^{2} + 420420 x y^{3} - 554160 x y^{2} + 160920 x y + 2460 x - 10465 y^{3} + 4600 y^{2} + 6930 y - 2833\right)}{702}\\\displaystyle \frac{5 x \left(- 140140 x^{3} y^{3} + 210210 x^{3} y^{2} - 84084 x^{3} y + 7007 x^{3} + 345800 x^{2} y^{3} - 516594 x^{2} y^{2} + 205752 x^{2} y - 17128 x^{2} - 246792 x y^{3} + 367272 x y^{2} - 145656 x y + 12102 x + 41132 y^{3} - 60888 y^{2} + 23988 y - 1981\right)}{702}\end{array}\right)\)
This DOF is associated with edge 3 of the reference cell.
where \(e_{3}\) is the 3rd edge;
\(\hat{\boldsymbol{t}}_{3}\) is the tangent to edge 3;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{3}\).
\(\displaystyle \boldsymbol{\phi}_{14} = \left(\begin{array}{c}\displaystyle \frac{y \left(700700 x^{3} y^{3} - 1001000 x^{3} y^{2} + 360360 x^{3} y - 20020 x^{3} - 1051050 x^{2} y^{3} + 1441830 x^{2} y^{2} - 469260 x^{2} y + 11400 x^{2} + 420420 x y^{3} - 554160 x y^{2} + 160920 x y + 2460 x - 10465 y^{3} + 4600 y^{2} + 6930 y - 2833\right)}{702}\\\displaystyle \frac{5 x \left(- 140140 x^{3} y^{3} + 210210 x^{3} y^{2} - 84084 x^{3} y + 7007 x^{3} + 345800 x^{2} y^{3} - 516594 x^{2} y^{2} + 205752 x^{2} y - 17128 x^{2} - 246792 x y^{3} + 367272 x y^{2} - 145656 x y + 12102 x + 41132 y^{3} - 60888 y^{2} + 23988 y - 1981\right)}{702}\end{array}\right)\)
This DOF is associated with edge 3 of the reference cell.
\(\displaystyle l_{15}:\boldsymbol{v}\mapsto\displaystyle\int_{e_{3}}\boldsymbol{v}\cdot(\frac{9 s_{0} \left(- 3 s_{0}^{2} + 4 s_{0} - 1\right)}{2})\hat{\boldsymbol{t}}_{3}\)
where \(e_{3}\) is the 3rd edge;
\(\hat{\boldsymbol{t}}_{3}\) is the tangent to edge 3;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{3}\).
\(\displaystyle \boldsymbol{\phi}_{15} = \left(\begin{array}{c}\displaystyle \frac{y \left(- 700700 x^{3} y^{3} + 1001000 x^{3} y^{2} - 360360 x^{3} y + 20020 x^{3} + 1051050 x^{2} y^{3} - 1561170 x^{2} y^{2} + 611820 x^{2} y - 48660 x^{2} - 420420 x y^{3} + 673500 x y^{2} - 303480 x y + 34800 x + 59605 y^{3} - 108730 y^{2} + 58950 y - 8993\right)}{702}\\\displaystyle \frac{5 x \left(140140 x^{3} y^{3} - 210210 x^{3} y^{2} + 84084 x^{3} y - 7007 x^{3} - 214760 x^{2} y^{3} + 324246 x^{2} y^{2} - 130584 x^{2} y + 10900 x^{2} + 50232 x y^{3} - 78750 x y^{2} + 32904 x y - 2760 x + 24388 y^{3} - 35286 y^{2} + 13596 y - 1133\right)}{702}\end{array}\right)\)
This DOF is associated with edge 3 of the reference cell.
where \(e_{3}\) is the 3rd edge;
\(\hat{\boldsymbol{t}}_{3}\) is the tangent to edge 3;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{3}\).
\(\displaystyle \boldsymbol{\phi}_{15} = \left(\begin{array}{c}\displaystyle \frac{y \left(- 700700 x^{3} y^{3} + 1001000 x^{3} y^{2} - 360360 x^{3} y + 20020 x^{3} + 1051050 x^{2} y^{3} - 1561170 x^{2} y^{2} + 611820 x^{2} y - 48660 x^{2} - 420420 x y^{3} + 673500 x y^{2} - 303480 x y + 34800 x + 59605 y^{3} - 108730 y^{2} + 58950 y - 8993\right)}{702}\\\displaystyle \frac{5 x \left(140140 x^{3} y^{3} - 210210 x^{3} y^{2} + 84084 x^{3} y - 7007 x^{3} - 214760 x^{2} y^{3} + 324246 x^{2} y^{2} - 130584 x^{2} y + 10900 x^{2} + 50232 x y^{3} - 78750 x y^{2} + 32904 x y - 2760 x + 24388 y^{3} - 35286 y^{2} + 13596 y - 1133\right)}{702}\end{array}\right)\)
This DOF is associated with edge 3 of the reference cell.
\(\displaystyle l_{16}:\mathbf{v}\mapsto\displaystyle\int_{R}\left(\begin{array}{c}\displaystyle 1\\\displaystyle 0\end{array}\right)\cdot\mathbf{v}\)
where \(R\) is the reference element.
\(\displaystyle \boldsymbol{\phi}_{16} = \left(\begin{array}{c}\displaystyle \frac{30 y \left(12740 x^{3} y^{3} - 29120 x^{3} y^{2} + 21112 x^{3} y - 4732 x^{3} - 19110 x^{2} y^{3} + 44070 x^{2} y^{2} - 32268 x^{2} y + 7308 x^{2} + 7644 x y^{3} - 17988 x y^{2} + 13464 x y - 3120 x - 819 y^{3} + 1974 y^{2} - 1514 y + 359\right)}{13}\\\displaystyle \frac{30 x \left(- 12740 x^{3} y^{3} + 19110 x^{3} y^{2} - 7644 x^{3} y + 637 x^{3} + 32760 x^{2} y^{3} - 49530 x^{2} y^{2} + 20088 x^{2} y - 1724 x^{2} - 28392 x y^{3} + 43218 x y^{2} - 17736 x y + 1560 x + 8372 y^{3} - 12798 y^{2} + 5292 y - 473\right)}{13}\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
where \(R\) is the reference element.
\(\displaystyle \boldsymbol{\phi}_{16} = \left(\begin{array}{c}\displaystyle \frac{30 y \left(12740 x^{3} y^{3} - 29120 x^{3} y^{2} + 21112 x^{3} y - 4732 x^{3} - 19110 x^{2} y^{3} + 44070 x^{2} y^{2} - 32268 x^{2} y + 7308 x^{2} + 7644 x y^{3} - 17988 x y^{2} + 13464 x y - 3120 x - 819 y^{3} + 1974 y^{2} - 1514 y + 359\right)}{13}\\\displaystyle \frac{30 x \left(- 12740 x^{3} y^{3} + 19110 x^{3} y^{2} - 7644 x^{3} y + 637 x^{3} + 32760 x^{2} y^{3} - 49530 x^{2} y^{2} + 20088 x^{2} y - 1724 x^{2} - 28392 x y^{3} + 43218 x y^{2} - 17736 x y + 1560 x + 8372 y^{3} - 12798 y^{2} + 5292 y - 473\right)}{13}\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
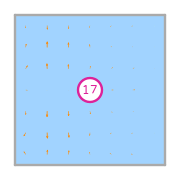
\(\displaystyle l_{17}:\mathbf{v}\mapsto\displaystyle\int_{R}\left(\begin{array}{c}\displaystyle 2 s_{1}\\\displaystyle 0\end{array}\right)\cdot\mathbf{v}\)
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{17} = \left(\begin{array}{c}\displaystyle \frac{15 y \left(- 63700 x^{3} y^{3} + 134680 x^{3} y^{2} - 87360 x^{3} y + 16380 x^{3} + 95550 x^{2} y^{3} - 202800 x^{2} y^{2} + 132210 x^{2} y - 24960 x^{2} - 38220 x y^{3} + 81840 x y^{2} - 53964 x y + 10344 x + 4095 y^{3} - 8862 y^{2} + 5922 y - 1155\right)}{13}\\\displaystyle \frac{15 x \left(63700 x^{3} y^{3} - 95550 x^{3} y^{2} + 38220 x^{3} y - 3185 x^{3} - 163800 x^{2} y^{3} + 246480 x^{2} y^{2} - 99060 x^{2} y + 8320 x^{2} + 141960 x y^{3} - 214200 x y^{2} + 86436 x y - 7308 x - 41860 y^{3} + 63270 y^{2} - 25596 y + 2173\right)}{13}\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{17} = \left(\begin{array}{c}\displaystyle \frac{15 y \left(- 63700 x^{3} y^{3} + 134680 x^{3} y^{2} - 87360 x^{3} y + 16380 x^{3} + 95550 x^{2} y^{3} - 202800 x^{2} y^{2} + 132210 x^{2} y - 24960 x^{2} - 38220 x y^{3} + 81840 x y^{2} - 53964 x y + 10344 x + 4095 y^{3} - 8862 y^{2} + 5922 y - 1155\right)}{13}\\\displaystyle \frac{15 x \left(63700 x^{3} y^{3} - 95550 x^{3} y^{2} + 38220 x^{3} y - 3185 x^{3} - 163800 x^{2} y^{3} + 246480 x^{2} y^{2} - 99060 x^{2} y + 8320 x^{2} + 141960 x y^{3} - 214200 x y^{2} + 86436 x y - 7308 x - 41860 y^{3} + 63270 y^{2} - 25596 y + 2173\right)}{13}\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
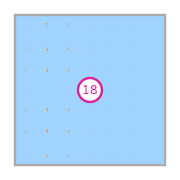
\(\displaystyle l_{18}:\mathbf{v}\mapsto\displaystyle\int_{R}\left(\begin{array}{c}\displaystyle 3 s_{1}^{2}\\\displaystyle 0\end{array}\right)\cdot\mathbf{v}\)
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{18} = \left(\begin{array}{c}\displaystyle 70 y \left(700 x^{3} y^{3} - 1400 x^{3} y^{2} + 840 x^{3} y - 140 x^{3} - 1050 x^{2} y^{3} + 2100 x^{2} y^{2} - 1260 x^{2} y + 210 x^{2} + 420 x y^{3} - 840 x y^{2} + 504 x y - 84 x - 45 y^{3} + 90 y^{2} - 54 y + 9\right)\\\displaystyle 70 x \left(- 700 x^{3} y^{3} + 1050 x^{3} y^{2} - 420 x^{3} y + 35 x^{3} + 1800 x^{2} y^{3} - 2700 x^{2} y^{2} + 1080 x^{2} y - 90 x^{2} - 1560 x y^{3} + 2340 x y^{2} - 936 x y + 78 x + 460 y^{3} - 690 y^{2} + 276 y - 23\right)\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{18} = \left(\begin{array}{c}\displaystyle 70 y \left(700 x^{3} y^{3} - 1400 x^{3} y^{2} + 840 x^{3} y - 140 x^{3} - 1050 x^{2} y^{3} + 2100 x^{2} y^{2} - 1260 x^{2} y + 210 x^{2} + 420 x y^{3} - 840 x y^{2} + 504 x y - 84 x - 45 y^{3} + 90 y^{2} - 54 y + 9\right)\\\displaystyle 70 x \left(- 700 x^{3} y^{3} + 1050 x^{3} y^{2} - 420 x^{3} y + 35 x^{3} + 1800 x^{2} y^{3} - 2700 x^{2} y^{2} + 1080 x^{2} y - 90 x^{2} - 1560 x y^{3} + 2340 x y^{2} - 936 x y + 78 x + 460 y^{3} - 690 y^{2} + 276 y - 23\right)\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
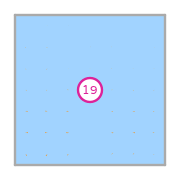
\(\displaystyle l_{19}:\mathbf{v}\mapsto\displaystyle\int_{R}\left(\begin{array}{c}\displaystyle 0\\\displaystyle -1\end{array}\right)\cdot\mathbf{v}\)
where \(R\) is the reference element.
\(\displaystyle \boldsymbol{\phi}_{19} = \left(\begin{array}{c}\displaystyle \frac{30 y \left(12740 x^{3} y^{3} - 32760 x^{3} y^{2} + 28392 x^{3} y - 8372 x^{3} - 19110 x^{2} y^{3} + 49530 x^{2} y^{2} - 43218 x^{2} y + 12798 x^{2} + 7644 x y^{3} - 20088 x y^{2} + 17736 x y - 5292 x - 637 y^{3} + 1724 y^{2} - 1560 y + 473\right)}{13}\\\displaystyle \frac{30 x \left(- 12740 x^{3} y^{3} + 19110 x^{3} y^{2} - 7644 x^{3} y + 819 x^{3} + 29120 x^{2} y^{3} - 44070 x^{2} y^{2} + 17988 x^{2} y - 1974 x^{2} - 21112 x y^{3} + 32268 x y^{2} - 13464 x y + 1514 x + 4732 y^{3} - 7308 y^{2} + 3120 y - 359\right)}{13}\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
where \(R\) is the reference element.
\(\displaystyle \boldsymbol{\phi}_{19} = \left(\begin{array}{c}\displaystyle \frac{30 y \left(12740 x^{3} y^{3} - 32760 x^{3} y^{2} + 28392 x^{3} y - 8372 x^{3} - 19110 x^{2} y^{3} + 49530 x^{2} y^{2} - 43218 x^{2} y + 12798 x^{2} + 7644 x y^{3} - 20088 x y^{2} + 17736 x y - 5292 x - 637 y^{3} + 1724 y^{2} - 1560 y + 473\right)}{13}\\\displaystyle \frac{30 x \left(- 12740 x^{3} y^{3} + 19110 x^{3} y^{2} - 7644 x^{3} y + 819 x^{3} + 29120 x^{2} y^{3} - 44070 x^{2} y^{2} + 17988 x^{2} y - 1974 x^{2} - 21112 x y^{3} + 32268 x y^{2} - 13464 x y + 1514 x + 4732 y^{3} - 7308 y^{2} + 3120 y - 359\right)}{13}\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
\(\displaystyle l_{20}:\mathbf{v}\mapsto\displaystyle\int_{R}\left(\begin{array}{c}\displaystyle s_{0}\\\displaystyle - s_{1}\end{array}\right)\cdot\mathbf{v}\)
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{20} = \left(\begin{array}{c}\displaystyle \frac{360 y \left(- 12740 x^{3} y^{3} + 29120 x^{3} y^{2} - 21112 x^{3} y + 4732 x^{3} + 19110 x^{2} y^{3} - 43875 x^{2} y^{2} + 31968 x^{2} y - 7203 x^{2} - 7644 x y^{3} + 17688 x y^{2} - 13000 x y + 2956 x + 637 y^{3} - 1499 y^{2} + 1122 y - 260\right)}{13}\\\displaystyle \frac{360 x \left(12740 x^{3} y^{3} - 19110 x^{3} y^{2} + 7644 x^{3} y - 637 x^{3} - 29120 x^{2} y^{3} + 43875 x^{2} y^{2} - 17688 x^{2} y + 1499 x^{2} + 21112 x y^{3} - 31968 x y^{2} + 13000 x y - 1122 x - 4732 y^{3} + 7203 y^{2} - 2956 y + 260\right)}{13}\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{20} = \left(\begin{array}{c}\displaystyle \frac{360 y \left(- 12740 x^{3} y^{3} + 29120 x^{3} y^{2} - 21112 x^{3} y + 4732 x^{3} + 19110 x^{2} y^{3} - 43875 x^{2} y^{2} + 31968 x^{2} y - 7203 x^{2} - 7644 x y^{3} + 17688 x y^{2} - 13000 x y + 2956 x + 637 y^{3} - 1499 y^{2} + 1122 y - 260\right)}{13}\\\displaystyle \frac{360 x \left(12740 x^{3} y^{3} - 19110 x^{3} y^{2} + 7644 x^{3} y - 637 x^{3} - 29120 x^{2} y^{3} + 43875 x^{2} y^{2} - 17688 x^{2} y + 1499 x^{2} + 21112 x y^{3} - 31968 x y^{2} + 13000 x y - 1122 x - 4732 y^{3} + 7203 y^{2} - 2956 y + 260\right)}{13}\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
\(\displaystyle l_{21}:\mathbf{v}\mapsto\displaystyle\int_{R}\left(\begin{array}{c}\displaystyle 2 s_{0} s_{1}\\\displaystyle - s_{1}^{2}\end{array}\right)\cdot\mathbf{v}\)
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{21} = \left(\begin{array}{c}\displaystyle \frac{180 y \left(63700 x^{3} y^{3} - 134680 x^{3} y^{2} + 87360 x^{3} y - 16380 x^{3} - 95550 x^{2} y^{3} + 202410 x^{2} y^{2} - 131625 x^{2} y + 24765 x^{2} + 38220 x y^{3} - 81240 x y^{2} + 53064 x y - 10044 x - 3185 y^{3} + 6820 y^{2} - 4497 y + 862\right)}{13}\\\displaystyle \frac{180 x \left(- 63700 x^{3} y^{3} + 95550 x^{3} y^{2} - 38220 x^{3} y + 3185 x^{3} + 145600 x^{2} y^{3} - 218790 x^{2} y^{2} + 87750 x^{2} y - 7345 x^{2} - 105560 x y^{3} + 158940 x y^{2} - 63936 x y + 5378 x + 23660 y^{3} - 35700 y^{2} + 14406 y - 1218\right)}{13}\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{21} = \left(\begin{array}{c}\displaystyle \frac{180 y \left(63700 x^{3} y^{3} - 134680 x^{3} y^{2} + 87360 x^{3} y - 16380 x^{3} - 95550 x^{2} y^{3} + 202410 x^{2} y^{2} - 131625 x^{2} y + 24765 x^{2} + 38220 x y^{3} - 81240 x y^{2} + 53064 x y - 10044 x - 3185 y^{3} + 6820 y^{2} - 4497 y + 862\right)}{13}\\\displaystyle \frac{180 x \left(- 63700 x^{3} y^{3} + 95550 x^{3} y^{2} - 38220 x^{3} y + 3185 x^{3} + 145600 x^{2} y^{3} - 218790 x^{2} y^{2} + 87750 x^{2} y - 7345 x^{2} - 105560 x y^{3} + 158940 x y^{2} - 63936 x y + 5378 x + 23660 y^{3} - 35700 y^{2} + 14406 y - 1218\right)}{13}\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
\(\displaystyle l_{22}:\mathbf{v}\mapsto\displaystyle\int_{R}\left(\begin{array}{c}\displaystyle 3 s_{0} s_{1}^{2}\\\displaystyle - s_{1}^{3}\end{array}\right)\cdot\mathbf{v}\)
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{22} = \left(\begin{array}{c}\displaystyle 5880 y \left(- 100 x^{3} y^{3} + 200 x^{3} y^{2} - 120 x^{3} y + 20 x^{3} + 150 x^{2} y^{3} - 300 x^{2} y^{2} + 180 x^{2} y - 30 x^{2} - 60 x y^{3} + 120 x y^{2} - 72 x y + 12 x + 5 y^{3} - 10 y^{2} + 6 y - 1\right)\\\displaystyle 840 x \left(700 x^{3} y^{3} - 1050 x^{3} y^{2} + 420 x^{3} y - 35 x^{3} - 1600 x^{2} y^{3} + 2400 x^{2} y^{2} - 960 x^{2} y + 80 x^{2} + 1160 x y^{3} - 1740 x y^{2} + 696 x y - 58 x - 260 y^{3} + 390 y^{2} - 156 y + 13\right)\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{22} = \left(\begin{array}{c}\displaystyle 5880 y \left(- 100 x^{3} y^{3} + 200 x^{3} y^{2} - 120 x^{3} y + 20 x^{3} + 150 x^{2} y^{3} - 300 x^{2} y^{2} + 180 x^{2} y - 30 x^{2} - 60 x y^{3} + 120 x y^{2} - 72 x y + 12 x + 5 y^{3} - 10 y^{2} + 6 y - 1\right)\\\displaystyle 840 x \left(700 x^{3} y^{3} - 1050 x^{3} y^{2} + 420 x^{3} y - 35 x^{3} - 1600 x^{2} y^{3} + 2400 x^{2} y^{2} - 960 x^{2} y + 80 x^{2} + 1160 x y^{3} - 1740 x y^{2} + 696 x y - 58 x - 260 y^{3} + 390 y^{2} - 156 y + 13\right)\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
\(\displaystyle l_{23}:\mathbf{v}\mapsto\displaystyle\int_{R}\left(\begin{array}{c}\displaystyle 0\\\displaystyle - 2 s_{0}\end{array}\right)\cdot\mathbf{v}\)
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{23} = \left(\begin{array}{c}\displaystyle \frac{15 y \left(- 63700 x^{3} y^{3} + 163800 x^{3} y^{2} - 141960 x^{3} y + 41860 x^{3} + 95550 x^{2} y^{3} - 246480 x^{2} y^{2} + 214200 x^{2} y - 63270 x^{2} - 38220 x y^{3} + 99060 x y^{2} - 86436 x y + 25596 x + 3185 y^{3} - 8320 y^{2} + 7308 y - 2173\right)}{13}\\\displaystyle \frac{15 x \left(63700 x^{3} y^{3} - 95550 x^{3} y^{2} + 38220 x^{3} y - 4095 x^{3} - 134680 x^{2} y^{3} + 202800 x^{2} y^{2} - 81840 x^{2} y + 8862 x^{2} + 87360 x y^{3} - 132210 x y^{2} + 53964 x y - 5922 x - 16380 y^{3} + 24960 y^{2} - 10344 y + 1155\right)}{13}\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{23} = \left(\begin{array}{c}\displaystyle \frac{15 y \left(- 63700 x^{3} y^{3} + 163800 x^{3} y^{2} - 141960 x^{3} y + 41860 x^{3} + 95550 x^{2} y^{3} - 246480 x^{2} y^{2} + 214200 x^{2} y - 63270 x^{2} - 38220 x y^{3} + 99060 x y^{2} - 86436 x y + 25596 x + 3185 y^{3} - 8320 y^{2} + 7308 y - 2173\right)}{13}\\\displaystyle \frac{15 x \left(63700 x^{3} y^{3} - 95550 x^{3} y^{2} + 38220 x^{3} y - 4095 x^{3} - 134680 x^{2} y^{3} + 202800 x^{2} y^{2} - 81840 x^{2} y + 8862 x^{2} + 87360 x y^{3} - 132210 x y^{2} + 53964 x y - 5922 x - 16380 y^{3} + 24960 y^{2} - 10344 y + 1155\right)}{13}\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
\(\displaystyle l_{24}:\mathbf{v}\mapsto\displaystyle\int_{R}\left(\begin{array}{c}\displaystyle s_{0}^{2}\\\displaystyle - 2 s_{0} s_{1}\end{array}\right)\cdot\mathbf{v}\)
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{24} = \left(\begin{array}{c}\displaystyle \frac{180 y \left(63700 x^{3} y^{3} - 145600 x^{3} y^{2} + 105560 x^{3} y - 23660 x^{3} - 95550 x^{2} y^{3} + 218790 x^{2} y^{2} - 158940 x^{2} y + 35700 x^{2} + 38220 x y^{3} - 87750 x y^{2} + 63936 x y - 14406 x - 3185 y^{3} + 7345 y^{2} - 5378 y + 1218\right)}{13}\\\displaystyle \frac{180 x \left(- 63700 x^{3} y^{3} + 95550 x^{3} y^{2} - 38220 x^{3} y + 3185 x^{3} + 134680 x^{2} y^{3} - 202410 x^{2} y^{2} + 81240 x^{2} y - 6820 x^{2} - 87360 x y^{3} + 131625 x y^{2} - 53064 x y + 4497 x + 16380 y^{3} - 24765 y^{2} + 10044 y - 862\right)}{13}\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{24} = \left(\begin{array}{c}\displaystyle \frac{180 y \left(63700 x^{3} y^{3} - 145600 x^{3} y^{2} + 105560 x^{3} y - 23660 x^{3} - 95550 x^{2} y^{3} + 218790 x^{2} y^{2} - 158940 x^{2} y + 35700 x^{2} + 38220 x y^{3} - 87750 x y^{2} + 63936 x y - 14406 x - 3185 y^{3} + 7345 y^{2} - 5378 y + 1218\right)}{13}\\\displaystyle \frac{180 x \left(- 63700 x^{3} y^{3} + 95550 x^{3} y^{2} - 38220 x^{3} y + 3185 x^{3} + 134680 x^{2} y^{3} - 202410 x^{2} y^{2} + 81240 x^{2} y - 6820 x^{2} - 87360 x y^{3} + 131625 x y^{2} - 53064 x y + 4497 x + 16380 y^{3} - 24765 y^{2} + 10044 y - 862\right)}{13}\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
\(\displaystyle l_{25}:\mathbf{v}\mapsto\displaystyle\int_{R}\left(\begin{array}{c}\displaystyle 2 s_{0}^{2} s_{1}\\\displaystyle - 2 s_{0} s_{1}^{2}\end{array}\right)\cdot\mathbf{v}\)
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{25} = \left(\begin{array}{c}\displaystyle 450 y \left(- 4900 x^{3} y^{3} + 10360 x^{3} y^{2} - 6720 x^{3} y + 1260 x^{3} + 7350 x^{2} y^{3} - 15552 x^{2} y^{2} + 10098 x^{2} y - 1896 x^{2} - 2940 x y^{3} + 6228 x y^{2} - 4050 x y + 762 x + 245 y^{3} - 520 y^{2} + 339 y - 64\right)\\\displaystyle 450 x \left(4900 x^{3} y^{3} - 7350 x^{3} y^{2} + 2940 x^{3} y - 245 x^{3} - 10360 x^{2} y^{3} + 15552 x^{2} y^{2} - 6228 x^{2} y + 520 x^{2} + 6720 x y^{3} - 10098 x y^{2} + 4050 x y - 339 x - 1260 y^{3} + 1896 y^{2} - 762 y + 64\right)\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{25} = \left(\begin{array}{c}\displaystyle 450 y \left(- 4900 x^{3} y^{3} + 10360 x^{3} y^{2} - 6720 x^{3} y + 1260 x^{3} + 7350 x^{2} y^{3} - 15552 x^{2} y^{2} + 10098 x^{2} y - 1896 x^{2} - 2940 x y^{3} + 6228 x y^{2} - 4050 x y + 762 x + 245 y^{3} - 520 y^{2} + 339 y - 64\right)\\\displaystyle 450 x \left(4900 x^{3} y^{3} - 7350 x^{3} y^{2} + 2940 x^{3} y - 245 x^{3} - 10360 x^{2} y^{3} + 15552 x^{2} y^{2} - 6228 x^{2} y + 520 x^{2} + 6720 x y^{3} - 10098 x y^{2} + 4050 x y - 339 x - 1260 y^{3} + 1896 y^{2} - 762 y + 64\right)\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
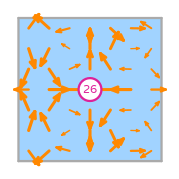
\(\displaystyle l_{26}:\mathbf{v}\mapsto\displaystyle\int_{R}\left(\begin{array}{c}\displaystyle 3 s_{0}^{2} s_{1}^{2}\\\displaystyle - 2 s_{0} s_{1}^{3}\end{array}\right)\cdot\mathbf{v}\)
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{26} = \left(\begin{array}{c}\displaystyle 14700 y \left(100 x^{3} y^{3} - 200 x^{3} y^{2} + 120 x^{3} y - 20 x^{3} - 150 x^{2} y^{3} + 300 x^{2} y^{2} - 180 x^{2} y + 30 x^{2} + 60 x y^{3} - 120 x y^{2} + 72 x y - 12 x - 5 y^{3} + 10 y^{2} - 6 y + 1\right)\\\displaystyle 2100 x \left(- 700 x^{3} y^{3} + 1050 x^{3} y^{2} - 420 x^{3} y + 35 x^{3} + 1480 x^{2} y^{3} - 2220 x^{2} y^{2} + 888 x^{2} y - 74 x^{2} - 960 x y^{3} + 1440 x y^{2} - 576 x y + 48 x + 180 y^{3} - 270 y^{2} + 108 y - 9\right)\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{26} = \left(\begin{array}{c}\displaystyle 14700 y \left(100 x^{3} y^{3} - 200 x^{3} y^{2} + 120 x^{3} y - 20 x^{3} - 150 x^{2} y^{3} + 300 x^{2} y^{2} - 180 x^{2} y + 30 x^{2} + 60 x y^{3} - 120 x y^{2} + 72 x y - 12 x - 5 y^{3} + 10 y^{2} - 6 y + 1\right)\\\displaystyle 2100 x \left(- 700 x^{3} y^{3} + 1050 x^{3} y^{2} - 420 x^{3} y + 35 x^{3} + 1480 x^{2} y^{3} - 2220 x^{2} y^{2} + 888 x^{2} y - 74 x^{2} - 960 x y^{3} + 1440 x y^{2} - 576 x y + 48 x + 180 y^{3} - 270 y^{2} + 108 y - 9\right)\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
\(\displaystyle l_{27}:\mathbf{v}\mapsto\displaystyle\int_{R}\left(\begin{array}{c}\displaystyle 0\\\displaystyle - 3 s_{0}^{2}\end{array}\right)\cdot\mathbf{v}\)
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{27} = \left(\begin{array}{c}\displaystyle 70 y \left(700 x^{3} y^{3} - 1800 x^{3} y^{2} + 1560 x^{3} y - 460 x^{3} - 1050 x^{2} y^{3} + 2700 x^{2} y^{2} - 2340 x^{2} y + 690 x^{2} + 420 x y^{3} - 1080 x y^{2} + 936 x y - 276 x - 35 y^{3} + 90 y^{2} - 78 y + 23\right)\\\displaystyle 70 x \left(- 700 x^{3} y^{3} + 1050 x^{3} y^{2} - 420 x^{3} y + 45 x^{3} + 1400 x^{2} y^{3} - 2100 x^{2} y^{2} + 840 x^{2} y - 90 x^{2} - 840 x y^{3} + 1260 x y^{2} - 504 x y + 54 x + 140 y^{3} - 210 y^{2} + 84 y - 9\right)\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{27} = \left(\begin{array}{c}\displaystyle 70 y \left(700 x^{3} y^{3} - 1800 x^{3} y^{2} + 1560 x^{3} y - 460 x^{3} - 1050 x^{2} y^{3} + 2700 x^{2} y^{2} - 2340 x^{2} y + 690 x^{2} + 420 x y^{3} - 1080 x y^{2} + 936 x y - 276 x - 35 y^{3} + 90 y^{2} - 78 y + 23\right)\\\displaystyle 70 x \left(- 700 x^{3} y^{3} + 1050 x^{3} y^{2} - 420 x^{3} y + 45 x^{3} + 1400 x^{2} y^{3} - 2100 x^{2} y^{2} + 840 x^{2} y - 90 x^{2} - 840 x y^{3} + 1260 x y^{2} - 504 x y + 54 x + 140 y^{3} - 210 y^{2} + 84 y - 9\right)\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
\(\displaystyle l_{28}:\mathbf{v}\mapsto\displaystyle\int_{R}\left(\begin{array}{c}\displaystyle s_{0}^{3}\\\displaystyle - 3 s_{0}^{2} s_{1}\end{array}\right)\cdot\mathbf{v}\)
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{28} = \left(\begin{array}{c}\displaystyle 840 y \left(- 700 x^{3} y^{3} + 1600 x^{3} y^{2} - 1160 x^{3} y + 260 x^{3} + 1050 x^{2} y^{3} - 2400 x^{2} y^{2} + 1740 x^{2} y - 390 x^{2} - 420 x y^{3} + 960 x y^{2} - 696 x y + 156 x + 35 y^{3} - 80 y^{2} + 58 y - 13\right)\\\displaystyle 5880 x \left(100 x^{3} y^{3} - 150 x^{3} y^{2} + 60 x^{3} y - 5 x^{3} - 200 x^{2} y^{3} + 300 x^{2} y^{2} - 120 x^{2} y + 10 x^{2} + 120 x y^{3} - 180 x y^{2} + 72 x y - 6 x - 20 y^{3} + 30 y^{2} - 12 y + 1\right)\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{28} = \left(\begin{array}{c}\displaystyle 840 y \left(- 700 x^{3} y^{3} + 1600 x^{3} y^{2} - 1160 x^{3} y + 260 x^{3} + 1050 x^{2} y^{3} - 2400 x^{2} y^{2} + 1740 x^{2} y - 390 x^{2} - 420 x y^{3} + 960 x y^{2} - 696 x y + 156 x + 35 y^{3} - 80 y^{2} + 58 y - 13\right)\\\displaystyle 5880 x \left(100 x^{3} y^{3} - 150 x^{3} y^{2} + 60 x^{3} y - 5 x^{3} - 200 x^{2} y^{3} + 300 x^{2} y^{2} - 120 x^{2} y + 10 x^{2} + 120 x y^{3} - 180 x y^{2} + 72 x y - 6 x - 20 y^{3} + 30 y^{2} - 12 y + 1\right)\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
\(\displaystyle l_{29}:\mathbf{v}\mapsto\displaystyle\int_{R}\left(\begin{array}{c}\displaystyle 2 s_{0}^{3} s_{1}\\\displaystyle - 3 s_{0}^{2} s_{1}^{2}\end{array}\right)\cdot\mathbf{v}\)
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{29} = \left(\begin{array}{c}\displaystyle 2100 y \left(700 x^{3} y^{3} - 1480 x^{3} y^{2} + 960 x^{3} y - 180 x^{3} - 1050 x^{2} y^{3} + 2220 x^{2} y^{2} - 1440 x^{2} y + 270 x^{2} + 420 x y^{3} - 888 x y^{2} + 576 x y - 108 x - 35 y^{3} + 74 y^{2} - 48 y + 9\right)\\\displaystyle 14700 x \left(- 100 x^{3} y^{3} + 150 x^{3} y^{2} - 60 x^{3} y + 5 x^{3} + 200 x^{2} y^{3} - 300 x^{2} y^{2} + 120 x^{2} y - 10 x^{2} - 120 x y^{3} + 180 x y^{2} - 72 x y + 6 x + 20 y^{3} - 30 y^{2} + 12 y - 1\right)\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{29} = \left(\begin{array}{c}\displaystyle 2100 y \left(700 x^{3} y^{3} - 1480 x^{3} y^{2} + 960 x^{3} y - 180 x^{3} - 1050 x^{2} y^{3} + 2220 x^{2} y^{2} - 1440 x^{2} y + 270 x^{2} + 420 x y^{3} - 888 x y^{2} + 576 x y - 108 x - 35 y^{3} + 74 y^{2} - 48 y + 9\right)\\\displaystyle 14700 x \left(- 100 x^{3} y^{3} + 150 x^{3} y^{2} - 60 x^{3} y + 5 x^{3} + 200 x^{2} y^{3} - 300 x^{2} y^{2} + 120 x^{2} y - 10 x^{2} - 120 x y^{3} + 180 x y^{2} - 72 x y + 6 x + 20 y^{3} - 30 y^{2} + 12 y - 1\right)\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
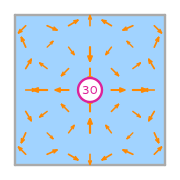
\(\displaystyle l_{30}:\mathbf{v}\mapsto\displaystyle\int_{R}\left(\begin{array}{c}\displaystyle 3 s_{0}^{3} s_{1}^{2}\\\displaystyle - 3 s_{0}^{2} s_{1}^{3}\end{array}\right)\cdot\mathbf{v}\)
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{30} = \left(\begin{array}{c}\displaystyle 9800 y \left(- 100 x^{3} y^{3} + 200 x^{3} y^{2} - 120 x^{3} y + 20 x^{3} + 150 x^{2} y^{3} - 300 x^{2} y^{2} + 180 x^{2} y - 30 x^{2} - 60 x y^{3} + 120 x y^{2} - 72 x y + 12 x + 5 y^{3} - 10 y^{2} + 6 y - 1\right)\\\displaystyle 9800 x \left(100 x^{3} y^{3} - 150 x^{3} y^{2} + 60 x^{3} y - 5 x^{3} - 200 x^{2} y^{3} + 300 x^{2} y^{2} - 120 x^{2} y + 10 x^{2} + 120 x y^{3} - 180 x y^{2} + 72 x y - 6 x - 20 y^{3} + 30 y^{2} - 12 y + 1\right)\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{30} = \left(\begin{array}{c}\displaystyle 9800 y \left(- 100 x^{3} y^{3} + 200 x^{3} y^{2} - 120 x^{3} y + 20 x^{3} + 150 x^{2} y^{3} - 300 x^{2} y^{2} + 180 x^{2} y - 30 x^{2} - 60 x y^{3} + 120 x y^{2} - 72 x y + 12 x + 5 y^{3} - 10 y^{2} + 6 y - 1\right)\\\displaystyle 9800 x \left(100 x^{3} y^{3} - 150 x^{3} y^{2} + 60 x^{3} y - 5 x^{3} - 200 x^{2} y^{3} + 300 x^{2} y^{2} - 120 x^{2} y + 10 x^{2} + 120 x y^{3} - 180 x y^{2} + 72 x y - 6 x - 20 y^{3} + 30 y^{2} - 12 y + 1\right)\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
\(\displaystyle l_{31}:\mathbf{v}\mapsto\displaystyle\int_{R}\left(\begin{array}{c}\displaystyle s_{1} \left(- 2 s_{0} s_{1} + 2 s_{0} + s_{1} - 1\right)\\\displaystyle s_{0} \left(- 2 s_{0} s_{1} + s_{0} + 2 s_{1} - 1\right)\end{array}\right)\cdot\mathbf{v}\)
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{31} = \left(\begin{array}{c}\displaystyle \frac{60 y \left(- 1365 x^{2} y^{2} + 2205 x^{2} y - 840 x^{2} + 1470 x y^{2} - 2382 x y + 912 x - 280 y^{2} + 456 y - 176\right)}{13}\\\displaystyle \frac{60 x \left(- 1365 x^{2} y^{2} + 1470 x^{2} y - 280 x^{2} + 2205 x y^{2} - 2382 x y + 456 x - 840 y^{2} + 912 y - 176\right)}{13}\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{31} = \left(\begin{array}{c}\displaystyle \frac{60 y \left(- 1365 x^{2} y^{2} + 2205 x^{2} y - 840 x^{2} + 1470 x y^{2} - 2382 x y + 912 x - 280 y^{2} + 456 y - 176\right)}{13}\\\displaystyle \frac{60 x \left(- 1365 x^{2} y^{2} + 1470 x^{2} y - 280 x^{2} + 2205 x y^{2} - 2382 x y + 456 x - 840 y^{2} + 912 y - 176\right)}{13}\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
\(\displaystyle l_{32}:\mathbf{v}\mapsto\displaystyle\int_{R}\left(\begin{array}{c}\displaystyle s_{1}^{2} \left(- 2 s_{0} s_{1} + 2 s_{0} + s_{1} - 1\right)\\\displaystyle s_{0} s_{1} \left(- 3 s_{0} s_{1} + 2 s_{0} + 3 s_{1} - 2\right)\end{array}\right)\cdot\mathbf{v}\)
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{32} = \left(\begin{array}{c}\displaystyle \frac{2100 y \left(78 x^{2} y^{2} - 117 x^{2} y + 39 x^{2} - 84 x y^{2} + 126 x y - 42 x + 16 y^{2} - 24 y + 8\right)}{13}\\\displaystyle \frac{2100 x \left(78 x^{2} y^{2} - 78 x^{2} y + 13 x^{2} - 126 x y^{2} + 126 x y - 21 x + 48 y^{2} - 48 y + 8\right)}{13}\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{32} = \left(\begin{array}{c}\displaystyle \frac{2100 y \left(78 x^{2} y^{2} - 117 x^{2} y + 39 x^{2} - 84 x y^{2} + 126 x y - 42 x + 16 y^{2} - 24 y + 8\right)}{13}\\\displaystyle \frac{2100 x \left(78 x^{2} y^{2} - 78 x^{2} y + 13 x^{2} - 126 x y^{2} + 126 x y - 21 x + 48 y^{2} - 48 y + 8\right)}{13}\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
\(\displaystyle l_{33}:\mathbf{v}\mapsto\displaystyle\int_{R}\left(\begin{array}{c}\displaystyle s_{0} s_{1} \left(- 3 s_{0} s_{1} + 3 s_{0} + 2 s_{1} - 2\right)\\\displaystyle s_{0}^{2} \left(- 2 s_{0} s_{1} + s_{0} + 2 s_{1} - 1\right)\end{array}\right)\cdot\mathbf{v}\)
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{33} = \left(\begin{array}{c}\displaystyle \frac{2100 y \left(78 x^{2} y^{2} - 126 x^{2} y + 48 x^{2} - 78 x y^{2} + 126 x y - 48 x + 13 y^{2} - 21 y + 8\right)}{13}\\\displaystyle \frac{2100 x \left(78 x^{2} y^{2} - 84 x^{2} y + 16 x^{2} - 117 x y^{2} + 126 x y - 24 x + 39 y^{2} - 42 y + 8\right)}{13}\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{33} = \left(\begin{array}{c}\displaystyle \frac{2100 y \left(78 x^{2} y^{2} - 126 x^{2} y + 48 x^{2} - 78 x y^{2} + 126 x y - 48 x + 13 y^{2} - 21 y + 8\right)}{13}\\\displaystyle \frac{2100 x \left(78 x^{2} y^{2} - 84 x^{2} y + 16 x^{2} - 117 x y^{2} + 126 x y - 24 x + 39 y^{2} - 42 y + 8\right)}{13}\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
\(\displaystyle l_{34}:\mathbf{v}\mapsto\displaystyle\int_{R}\left(\begin{array}{c}\displaystyle s_{0} s_{1}^{2} \left(- 3 s_{0} s_{1} + 3 s_{0} + 2 s_{1} - 2\right)\\\displaystyle s_{0}^{2} s_{1} \left(- 3 s_{0} s_{1} + 2 s_{0} + 3 s_{1} - 2\right)\end{array}\right)\cdot\mathbf{v}\)
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{34} = \left(\begin{array}{c}\displaystyle 2100 y \left(- 12 x^{2} y^{2} + 18 x^{2} y - 6 x^{2} + 12 x y^{2} - 18 x y + 6 x - 2 y^{2} + 3 y - 1\right)\\\displaystyle 2100 x \left(- 12 x^{2} y^{2} + 12 x^{2} y - 2 x^{2} + 18 x y^{2} - 18 x y + 3 x - 6 y^{2} + 6 y - 1\right)\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{34} = \left(\begin{array}{c}\displaystyle 2100 y \left(- 12 x^{2} y^{2} + 18 x^{2} y - 6 x^{2} + 12 x y^{2} - 18 x y + 6 x - 2 y^{2} + 3 y - 1\right)\\\displaystyle 2100 x \left(- 12 x^{2} y^{2} + 12 x^{2} y - 2 x^{2} + 18 x y^{2} - 18 x y + 3 x - 6 y^{2} + 6 y - 1\right)\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.