Tiniest tensor

Click here to read what the information on this page means.

Alternative names	TNT
De Rham complex families	\(\left[S_{3,k}^\square\right]_{0}\)
Degrees	\(1\leqslant k\) where \(k\) is the polynomial subdegree
Polynomial subdegree	\(k\)
Polynomial superdegree	\(\max(dk,d+k)\)
Lagrange subdegree	\(k\)
Lagrange superdegree	\(k+1\)
Reference cells	quadrilateral, hexahedron
Finite dimensional space	\(\mathcal{Q}_{k} \oplus \mathcal{Z}^{(30)}_{k}\) (quadrilateral) \(\mathcal{Q}_{k} \oplus \mathcal{Z}^{(31)}_{k} \oplus \mathcal{Z}^{(32)}_{k} \oplus \mathcal{Z}^{(33)}_{k}\) (hexahedron) ↓ Show set definitions ↓↑ Hide set definitions ↑ \(\mathcal{Q}_k=\operatorname{span}\left\{\prod_{i=1}^dx_i^{p_i}\middle\|\max_i(p_i)\leqslant k\right\}\) \(\mathcal{Z}^{(30)}_k=\left\{x\tilde{B}_{k+1}(y),(1-x)\tilde{B}_{k+1}(y),y\tilde{B}_{k+1}(x),(1-y)\tilde{B}_{k+1}(x)\right\} \) where \(\tilde{P}_k\) is the degree \(k\) Legendre polynomial on \([0,1]\) \(\tilde{B}_k\) is \((\tilde{P}_{k+2}-\tilde{P}_k)/(4k+6)\) \(\mathcal{Z}^{(31)}_k=\left\{xy\tilde{B}_{k+1}(z),(1-x)y\tilde{B}_{k+1}(z),(x(1-y)\tilde{B}_{k+1}(z),(1-x)(1-y)\tilde{B}_{k+1}(z)\right\} \) where \(\tilde{P}_k\) is the degree \(k\) Legendre polynomial on \([0,1]\) \(\tilde{B}_k\) is \((\tilde{P}_{k+2}-\tilde{P}_k)/(4k+6)\) \(\mathcal{Z}^{(32)}_k=\left\{xz\tilde{B}_{k+1}(y),(1-x)z\tilde{B}_{k+1}(y),(x(1-z)\tilde{B}_{k+1}(y),(1-x)(1-z)\tilde{B}_{k+1}(y)\right\}\) \(\mathcal{Z}^{(33)}_k=\left\{yz\tilde{B}_{k+1}(x),(1-y)z\tilde{B}_{k+1}(x),(y(1-z)\tilde{B}_{k+1}(x),(1-y)(1-z)\tilde{B}_{k+1}(x)\right\}\)
DOFs	On each vertex: point evaluations On each edge: integral moments with \(\frac{\partial}{\partial x}f\) for each \(f\) in a degree \(k\) Lagrange space On each face: integral moments with \(\Delta f\) for each \(f\) in a degree \(k\) Lagrange space such that the trace of \(f\) on the edges of the face is 0 On each volume: integral moments of gradient with \(\nabla f\) for each \(f\) in a degree \(k\) Lagrange space such that the trace of \(f\) on the faces of the volume is 0
Number of DOFs	quadrilateral: \(k^2 + 4\) hexahedron: \(k^3 + 12\)
Mapping	identity
continuity	Function values are continuous.
Categories	Scalar-valued elements

Implementations

This element is implemented in Basix.UFL and Symfem .↓ Show implementation detail ↓

↑ Hide implementation detail ↑

Basix.UFL

Uses custom element code
↓ Show Basix.UFL examples ↓

Before running this example, you must install Basix.UFL:

pip3 install git+https://github.com/FEniCS/basix fenics-ufl

This element can then be created with the following lines of Python:

import basix
import basix.ufl
import numpy as np

# Create Tiniest tensor degree 2 on a quadrilateral
e = basix.ufl.custom_element(
    basix.CellType.quadrilateral,
    (),
    np.array([[0.9999999999999996, 0.0, 0.0, -2.7755575615628914e-17, 0.0, 0.0, 0.0, 0.0, -8.326672684688674e-17], [0.4999999999999998, 0.28867513459481275, 0.0, 0.0, 0.0, 0.0, 1.3877787807814457e-17, -4.163336342344337e-17, -4.163336342344337e-17], [0.49999999999999983, 0.0, -1.3877787807814457e-17, 0.28867513459481275, 0.0, -2.7755575615628914e-17, 0.0, 1.3877787807814457e-17, -4.163336342344337e-17], [0.2499999999999999, 0.14433756729740635, 0.0, 0.14433756729740632, 0.08333333333333329, 0.0, 6.938893903907228e-18, -2.7755575615628914e-17, -1.3877787807814457e-17], [-0.12499999999999996, 0.07216878364870317, 1.734723475976807e-18, -6.505213034913027e-19, -1.734723475976807e-18, 0.0, 0.05590169943749474, -0.03227486121839513, 6.5052130349130266e-18], [-0.12499999999999994, -0.07216878364870319, 5.204170427930421e-18, 0.0, 0.0, 0.0, 0.05590169943749475, 0.03227486121839513, 6.938893903907228e-18], [-0.12499999999999996, 6.505213034913027e-19, 0.05590169943749475, 0.07216878364870319, 0.0, -0.03227486121839514, 1.0842021724855044e-18, -6.505213034913027e-19, 6.071532165918825e-18], [-0.12499999999999994, 0.0, 0.055901699437494734, -0.07216878364870319, 0.0, 0.03227486121839514, 5.204170427930421e-18, 0.0, 6.938893903907228e-18]], dtype=np.float64),
    [[np.array([[0.0, 0.0]], dtype=np.float64), np.array([[1.0, 0.0]], dtype=np.float64), np.array([[0.0, 1.0]], dtype=np.float64), np.array([[1.0, 1.0]], dtype=np.float64)], [np.array([[0.21132486540518713, 0.0], [0.7886751345948129, 0.0]], dtype=np.float64), np.array([[0.0, 0.21132486540518713], [0.0, 0.7886751345948129]], dtype=np.float64), np.array([[1.0, 0.21132486540518713], [1.0, 0.7886751345948129]], dtype=np.float64), np.array([[0.21132486540518713, 1.0], [0.7886751345948129, 1.0]], dtype=np.float64)], [np.empty((0, 2), dtype=np.float64)], []],
    [[np.array([[[[1.0]]]], dtype=np.float64), np.array([[[[1.0]]]], dtype=np.float64), np.array([[[[1.0]]]], dtype=np.float64), np.array([[[[1.0]]]], dtype=np.float64)], [np.array([[[[0.5], [0.5]]]], dtype=np.float64), np.array([[[[0.5], [0.5]]]], dtype=np.float64), np.array([[[[0.5], [0.5]]]], dtype=np.float64), np.array([[[[0.5], [0.5]]]], dtype=np.float64)], [np.empty((0, 1, 0, 1), dtype=np.float64)], []],
    0,
    basix.MapType.identity,
    basix.SobolevSpace.H1,
    False,
    -1,
    2,
    basix.PolysetType.standard, dtype=np.float64
)

# Create Tiniest tensor degree 3 on a quadrilateral
e = basix.ufl.custom_element(
    basix.CellType.quadrilateral,
    (),
    np.array([[0.9999999999999996, -8.326672684688674e-17, -3.608224830031759e-16, -1.942890293094024e-16, -9.71445146547012e-17, 0.0, 6.938893903907228e-18, 2.0816681711721685e-17, -3.885780586188048e-16, 0.0, 6.938893903907228e-18, -4.163336342344337e-17, -2.220446049250313e-16, 8.326672684688674e-17, -4.163336342344337e-17, 0.0], [0.4999999999999997, 0.28867513459481264, -2.706168622523819e-16, -3.3306690738754696e-16, -4.163336342344337e-17, -2.7755575615628914e-17, -8.673617379884035e-19, 7.806255641895632e-18, -1.95590071916385e-16, -9.627715291671279e-17, 2.6020852139652106e-18, -3.2959746043559335e-17, -8.326672684688674e-17, -2.7755575615628914e-17, -3.8163916471489756e-17, -4.163336342344337e-17], [0.33333333333333315, 0.28867513459481264, 0.07453559924999274, -3.434752482434078e-16, -2.7755575615628914e-17, -3.122502256758253e-17, 1.3877787807814457e-17, 8.673617379884035e-19, -1.2354483755472323e-16, -1.1053441148489718e-16, -2.7321894746634712e-17, -1.0272815584300155e-17, -5.551115123125783e-17, -2.7755575615628914e-17, -3.469446951953614e-17, -4.163336342344337e-17], [0.49999999999999967, -2.7755575615628914e-17, -1.942890293094024e-16, -9.020562075079397e-17, 0.28867513459481264, -2.7755575615628914e-17, -1.1796119636642288e-16, -6.591949208711867e-17, -2.7755575615628914e-16, 0.0, -1.3877787807814457e-17, -2.42861286636753e-17, -3.3306690738754696e-16, 1.3877787807814457e-17, -4.85722573273506e-17, -6.938893903907228e-18], [0.24999999999999983, 0.14433756729740632, -1.3704315460216776e-16, -1.5265566588595902e-16, 0.1443375672974063, 0.0833333333333332, -7.45931094670027e-17, -7.112366251504909e-17, -1.249000902703301e-16, -6.245004513516506e-17, 1.734723475976807e-18, -1.734723475976807e-18, -1.6653345369377348e-16, -5.551115123125783e-17, -2.42861286636753e-17, -2.42861286636753e-17], [0.16666666666666655, 0.14433756729740632, 0.037267799624996364, -1.6653345369377348e-16, 0.09622504486493753, 0.08333333333333322, 0.021516574145596677, -9.194034422677078e-17, -1.0408340855860843e-16, -6.245004513516506e-17, -2.6020852139652106e-17, -1.8214596497756474e-17, -1.249000902703301e-16, -6.938893903907228e-17, -4.163336342344337e-17, -6.938893903907228e-18], [0.33333333333333315, -2.7755575615628914e-17, -1.1796119636642288e-16, -7.632783294297951e-17, 0.28867513459481264, -2.7755575615628914e-17, -1.1449174941446927e-16, -6.245004513516506e-17, 0.07453559924999274, 1.3877787807814457e-17, -5.204170427930421e-17, -6.938893903907228e-18, -3.3306690738754696e-16, 1.3877787807814457e-17, -1.3877787807814457e-17, -1.3877787807814457e-17], [0.16666666666666657, 0.09622504486493755, -1.0061396160665481e-16, -1.1796119636642288e-16, 0.14433756729740632, 0.08333333333333322, -7.892991815694472e-17, -9.367506770274758e-17, 0.03726779962499635, 0.02151657414559669, -2.7755575615628914e-17, -4.163336342344337e-17, -1.5265566588595902e-16, -6.938893903907228e-17, -3.642919299551295e-17, -1.3877787807814457e-17], [0.11111111111111105, 0.09622504486493755, 0.02484519974999757, -1.1362438767648086e-16, 0.09622504486493755, 0.08333333333333323, 0.02151657414559667, -8.890457814381136e-17, 0.024845199749997576, 0.021516574145596677, 0.00555555555555552, -2.688821387764051e-17, -1.1796119636642288e-16, -7.632783294297951e-17, -2.949029909160572e-17, -1.3010426069826053e-17], [-6.938893903907228e-18, 1.734723475976807e-18, -1.734723475976807e-18, -8.673617379884035e-19, -0.057735026918962574, 0.033333333333333326, 1.431146867680866e-17, -1.474514954580286e-17, -1.3877787807814457e-17, 1.0408340855860843e-17, 2.6020852139652106e-18, -3.469446951953614e-18, 0.03779644730092273, -0.02182178902359924, -1.3444106938820255e-17, 5.204170427930421e-18], [-6.938893903907228e-18, -3.469446951953614e-18, -8.673617379884035e-19, -4.336808689942018e-19, -0.05773502691896257, -0.03333333333333331, 3.0357660829594124e-17, 3.469446951953614e-17, -1.734723475976807e-17, -1.0408340855860843e-17, 3.469446951953614e-18, 1.734723475976807e-18, 0.03779644730092273, 0.021821789023599228, -2.0816681711721685e-17, -1.9081958235744878e-17], [-6.938893903907228e-18, -0.057735026918962554, -1.734723475976807e-18, 0.03779644730092274, 7.806255641895632e-18, 0.03333333333333331, -8.673617379884035e-19, -0.021821789023599242, 1.734723475976807e-18, 1.734723475976807e-17, 5.204170427930421e-18, -1.9081958235744878e-17, -6.938893903907228e-18, -1.3877787807814457e-17, -8.673617379884035e-19, 2.0816681711721685e-17], [-1.0408340855860843e-17, -0.057735026918962554, -5.204170427930421e-18, 0.03779644730092274, -8.673617379884035e-18, -0.0333333333333333, 3.469446951953614e-18, 0.02182178902359924, -1.734723475976807e-18, 2.7755575615628914e-17, 6.938893903907228e-18, -1.214306433183765e-17, 3.469446951953614e-18, 2.7755575615628914e-17, 1.734723475976807e-18, -6.938893903907228e-18]], dtype=np.float64),
    [[np.array([[0.0, 0.0]], dtype=np.float64), np.array([[1.0, 0.0]], dtype=np.float64), np.array([[0.0, 1.0]], dtype=np.float64), np.array([[1.0, 1.0]], dtype=np.float64)], [np.array([[0.21132486540518713, 0.0], [0.7886751345948129, 0.0], [0.1127016653792583, 0.0], [0.5, 0.0], [0.8872983346207417, 0.0]], dtype=np.float64), np.array([[0.0, 0.21132486540518713], [0.0, 0.7886751345948129], [0.0, 0.1127016653792583], [0.0, 0.5], [0.0, 0.8872983346207417]], dtype=np.float64), np.array([[1.0, 0.21132486540518713], [1.0, 0.7886751345948129], [1.0, 0.1127016653792583], [1.0, 0.5], [1.0, 0.8872983346207417]], dtype=np.float64), np.array([[0.21132486540518713, 1.0], [0.7886751345948129, 1.0], [0.1127016653792583, 1.0], [0.5, 1.0], [0.8872983346207417, 1.0]], dtype=np.float64)], [np.array([[0.1127016653792583, 0.1127016653792583], [0.1127016653792583, 0.5], [0.1127016653792583, 0.8872983346207417], [0.5, 0.1127016653792583], [0.5, 0.5], [0.5, 0.8872983346207417], [0.8872983346207417, 0.1127016653792583], [0.8872983346207417, 0.5], [0.8872983346207417, 0.8872983346207417]], dtype=np.float64)], []],
    [[np.array([[[[1.0]]]], dtype=np.float64), np.array([[[[1.0]]]], dtype=np.float64), np.array([[[[1.0]]]], dtype=np.float64), np.array([[[[1.0]]]], dtype=np.float64)], [np.array([[[[0.5], [0.5], [0.0], [0.0], [0.0]]], [[[0.0], [0.0], [0.06261203632181014], [0.4444444444444444], [0.49294351923374524]]]], dtype=np.float64), np.array([[[[0.5], [0.5], [0.0], [0.0], [0.0]]], [[[0.0], [0.0], [0.06261203632181014], [0.4444444444444444], [0.49294351923374524]]]], dtype=np.float64), np.array([[[[0.5], [0.5], [0.0], [0.0], [0.0]]], [[[0.0], [0.0], [0.06261203632181014], [0.4444444444444444], [0.49294351923374524]]]], dtype=np.float64), np.array([[[[0.5], [0.5], [0.0], [0.0], [0.0]]], [[[0.0], [0.0], [0.06261203632181014], [0.4444444444444444], [0.49294351923374524]]]], dtype=np.float64)], [np.array([[[[-0.030864197530864168], [-0.08641975308641972], [-0.030864197530864168], [-0.08641975308641972], [-0.19753086419753085], [-0.08641975308641972], [-0.030864197530864168], [-0.08641975308641972], [-0.030864197530864168]]]], dtype=np.float64)], []],
    0,
    basix.MapType.identity,
    basix.SobolevSpace.H1,
    False,
    -1,
    3,
    basix.PolysetType.standard, dtype=np.float64
)

# Create Tiniest tensor degree 4 on a quadrilateral
e = basix.ufl.custom_element(
    basix.CellType.quadrilateral,
    (),
    np.array([[1.0000000000000002, -8.673617379884035e-17, -1.3530843112619095e-16, -1.734723475976807e-16, -6.696032617270475e-16, -8.673617379884035e-17, 0.0, 6.938893903907228e-18, 1.734723475976807e-17, -1.734723475976807e-17, -1.3183898417423734e-16, -2.7755575615628914e-17, 6.938893903907228e-17, 2.0816681711721685e-17, -4.163336342344337e-17, -1.457167719820518e-16, 2.7755575615628914e-17, 1.3877787807814457e-17, 0.0, -3.8163916471489756e-17, -6.83481049534862e-16, 1.3877787807814457e-17, -6.245004513516506e-17, -2.2551405187698492e-17, 6.938893903907228e-18], [0.5000000000000001, 0.2886751345948128, -1.9081958235744878e-16, -2.671474153004283e-16, -3.7643499428696714e-16, -3.8163916471489756e-17, -2.0816681711721685e-17, 6.938893903907228e-18, -6.938893903907228e-18, -3.469446951953614e-18, -6.938893903907228e-17, -2.0816681711721685e-17, 3.469446951953614e-17, 3.469446951953614e-18, -1.3877787807814457e-17, -5.898059818321144e-17, -4.163336342344337e-17, 0.0, -1.0408340855860843e-17, -8.673617379884035e-18, -3.903127820947816e-16, -1.682681771697503e-16, -1.734723475976807e-17, -1.3877787807814457e-17, 2.42861286636753e-17], [0.3333333333333334, 0.2886751345948129, 0.07453559924999284, -2.7755575615628914e-16, -2.654126918244515e-16, -2.0816681711721685e-17, -6.938893903907228e-18, 0.0, -6.938893903907228e-18, -1.734723475976807e-17, -2.7755575615628914e-17, -3.469446951953614e-17, 2.0816681711721685e-17, 1.734723475976807e-17, -6.938893903907228e-18, -5.898059818321144e-17, -2.7755575615628914e-17, 1.3877787807814457e-17, 2.0816681711721685e-17, -1.734723475976807e-18, -2.237793284010081e-16, -1.5265566588595902e-16, -6.245004513516506e-17, -1.214306433183765e-17, 2.7755575615628914e-17], [0.25000000000000006, 0.2598076211353316, 0.11180339887498934, 0.018898223650461104, -2.6194324487249787e-16, -1.734723475976807e-17, -1.734723475976807e-17, 0.0, -3.469446951953614e-18, -1.734723475976807e-18, -3.8163916471489756e-17, -3.122502256758253e-17, 6.938893903907228e-18, 0.0, -6.938893903907228e-18, -4.5102810375396984e-17, -4.5102810375396984e-17, 3.469446951953614e-18, -6.938893903907228e-18, -1.734723475976807e-18, -1.43982048506075e-16, -1.5439038936193583e-16, -8.326672684688674e-17, -2.2551405187698492e-17, 2.2551405187698492e-17], [0.5000000000000001, -3.8163916471489756e-17, -7.28583859910259e-17, -6.591949208711867e-17, -3.4867941867133823e-16, 0.28867513459481287, -2.0816681711721685e-17, -3.469446951953614e-17, -6.245004513516506e-17, -2.0469737016526324e-16, -1.8388068845354155e-16, 6.938893903907228e-18, 4.163336342344337e-17, 1.3877787807814457e-17, -2.7755575615628914e-17, -2.671474153004283e-16, 1.3877787807814457e-17, 0.0, -3.469446951953614e-18, -3.2959746043559335e-17, -3.625572064791527e-16, 2.6020852139652106e-17, -4.5102810375396984e-17, -2.42861286636753e-17, -3.469446951953614e-18], [0.25000000000000006, 0.14433756729740643, -8.673617379884035e-17, -1.3183898417423734e-16, -1.8041124150158794e-16, 0.14433756729740643, 0.08333333333333331, -3.469446951953614e-17, -7.979727989493313e-17, -1.0755285551056204e-16, -9.020562075079397e-17, -3.469446951953614e-17, 2.7755575615628914e-17, 3.469446951953614e-18, -1.3877787807814457e-17, -1.4224732503009818e-16, -6.245004513516506e-17, 2.0816681711721685e-17, -3.469446951953614e-18, -1.734723475976807e-17, -1.960237527853792e-16, -8.326672684688674e-17, -1.3877787807814457e-17, -1.9081958235744878e-17, 1.0408340855860843e-17], [0.1666666666666667, 0.14433756729740643, 0.03726779962499642, -1.249000902703301e-16, -1.4051260155412137e-16, 0.09622504486493763, 0.08333333333333333, 0.02151657414559672, -7.632783294297951e-17, -8.673617379884035e-17, -5.898059818321144e-17, -3.8163916471489756e-17, 0.0, 1.0408340855860843e-17, 0.0, -1.0408340855860843e-16, -8.673617379884035e-17, -6.938893903907228e-18, 3.469446951953614e-18, -5.204170427930421e-18, -1.3010426069826053e-16, -1.1275702593849246e-16, -3.469446951953614e-17, -1.214306433183765e-17, 1.5612511283791264e-17], [0.125, 0.12990381056766578, 0.055901699437494665, 0.009449111825230554, -1.3530843112619095e-16, 0.07216878364870322, 0.07499999999999998, 0.032274861218395096, 0.005455447255899727, -8.153200337090993e-17, -4.163336342344337e-17, -2.7755575615628914e-17, -3.469446951953614e-18, 0.0, 1.734723475976807e-18, -7.112366251504909e-17, -6.938893903907228e-17, -1.734723475976807e-17, 3.469446951953614e-18, 5.204170427930421e-18, -7.979727989493313e-17, -9.367506770274758e-17, -4.85722573273506e-17, -1.3877787807814457e-17, 9.540979117872439e-18], [0.33333333333333337, -2.42861286636753e-17, -3.469446951953614e-17, -5.204170427930421e-17, -2.2724877535296173e-16, 0.28867513459481287, 0.0, -3.469446951953614e-17, -6.245004513516506e-17, -2.0122792321330962e-16, 0.07453559924999284, -6.938893903907228e-18, 1.3877787807814457e-17, -3.469446951953614e-18, -7.28583859910259e-17, -2.8102520310824275e-16, 2.42861286636753e-17, 6.938893903907228e-18, 0.0, -4.163336342344337e-17, -2.931682674400804e-16, 2.7755575615628914e-17, -3.469446951953614e-17, -2.0816681711721685e-17, -6.938893903907228e-18], [0.16666666666666669, 0.09622504486493762, -5.551115123125783e-17, -1.0061396160665481e-16, -1.2836953722228372e-16, 0.14433756729740643, 0.08333333333333333, -4.5102810375396984e-17, -9.020562075079397e-17, -1.2836953722228372e-16, 0.03726779962499642, 0.021516574145596726, 6.938893903907228e-18, -1.0408340855860843e-17, -4.163336342344337e-17, -1.3877787807814457e-16, -6.591949208711867e-17, 1.0408340855860843e-17, 3.469446951953614e-18, -6.938893903907228e-18, -1.43982048506075e-16, -7.112366251504909e-17, 3.469446951953614e-18, -5.204170427930421e-18, 5.204170427930421e-18], [0.11111111111111113, 0.09622504486493762, 0.02484519974999761, -8.500145032286355e-17, -9.194034422677078e-17, 0.0962250448649376, 0.08333333333333331, 0.021516574145596712, -8.326672684688674e-17, -8.153200337090993e-17, 0.02484519974999761, 0.02151657414559672, 0.005555555555555543, -2.0816681711721685e-17, -2.2551405187698492e-17, -8.153200337090993e-17, -6.938893903907228e-17, -3.469446951953614e-18, 0.0, -3.469446951953614e-18, -8.673617379884035e-17, -6.591949208711867e-17, -1.734723475976807e-17, -1.0408340855860843e-17, 1.0408340855860843e-17], [0.08333333333333334, 0.08660254037844385, 0.03726779962499645, 0.0062994078834870335, -8.847089727481716e-17, 0.07216878364870322, 0.07499999999999998, 0.0322748612183951, 0.005455447255899734, -7.806255641895632e-17, 0.01863389981249821, 0.019364916731037046, 0.008333333333333314, 0.00140859042454752, -2.2551405187698492e-17, -6.938893903907228e-17, -5.898059818321144e-17, -1.734723475976807e-17, 0.0, -8.673617379884035e-18, -6.938893903907228e-17, -7.28583859910259e-17, -3.469446951953614e-17, -1.9081958235744878e-17, 9.540979117872439e-18], [0.25000000000000006, -2.42861286636753e-17, -3.122502256758253e-17, -4.163336342344337e-17, -1.6132928326584306e-16, 0.25980762113533157, -1.734723475976807e-17, -2.7755575615628914e-17, -4.85722573273506e-17, -1.8908485888147197e-16, 0.11180339887498934, -1.3877787807814457e-17, -6.938893903907228e-18, -1.3877787807814457e-17, -9.71445146547012e-17, 0.018898223650461107, 1.3877787807814457e-17, -2.0816681711721685e-17, -6.938893903907228e-18, -4.336808689942018e-17, -2.688821387764051e-16, 1.9081958235744878e-17, -3.122502256758253e-17, -1.0408340855860843e-17, -1.5612511283791264e-17], [0.125, 0.07216878364870322, -3.8163916471489756e-17, -6.765421556309548e-17, -9.020562075079397e-17, 0.12990381056766578, 0.07499999999999998, -3.122502256758253e-17, -7.632783294297951e-17, -9.8879238130678e-17, 0.055901699437494665, 0.0322748612183951, -6.938893903907228e-18, -2.42861286636753e-17, -5.551115123125783e-17, 0.009449111825230542, 0.0054554472558997445, 1.0408340855860843e-17, 3.469446951953614e-18, -1.5612511283791264e-17, -1.2836953722228372e-16, -5.551115123125783e-17, 3.469446951953614e-18, -1.0408340855860843e-17, 8.673617379884035e-18], [0.08333333333333334, 0.07216878364870322, 0.01863389981249821, -6.765421556309548e-17, -6.938893903907228e-17, 0.08660254037844385, 0.07499999999999998, 0.019364916731037046, -7.632783294297951e-17, -8.153200337090993e-17, 0.03726779962499645, 0.0322748612183951, 0.008333333333333314, -3.122502256758253e-17, -3.9898639947466563e-17, 0.0062994078834870335, 0.005455447255899758, 0.00140859042454752, 0.0, -1.214306433183765e-17, -9.020562075079397e-17, -6.938893903907228e-17, -1.9081958235744878e-17, -1.214306433183765e-17, 1.1275702593849246e-17], [0.0625, 0.06495190528383289, 0.027950849718747332, 0.004724555912615277, -6.852157730108388e-17, 0.06495190528383289, 0.06749999999999998, 0.029047375096555587, 0.004909902530309759, -6.765421556309548e-17, 0.027950849718747332, 0.02904737509655559, 0.012499999999999976, 0.0021128856368212642, -3.122502256758253e-17, 0.004724555912615273, 0.004909902530309769, 0.0021128856368212677, 0.000357142857142851, -6.938893903907228e-18, -7.112366251504909e-17, -6.245004513516506e-17, -2.7755575615628914e-17, -1.5612511283791264e-17, 6.938893903907228e-18], [-3.2526065174565133e-18, 1.179069862577986e-18, -5.55653613398821e-19, 0.0, -4.404571325722362e-19, 2.913793338554793e-18, -2.1955093992831465e-18, 1.8973538018496328e-19, -1.7618285302889447e-19, 9.486769009248164e-19, -0.037267799624996496, 0.021516574145596764, 2.981555974335137e-19, -1.0760706561918632e-17, 2.59801945581839e-17, -5.204170427930421e-18, 2.371692252312041e-18, 3.1170812458958252e-18, 1.2739375526704677e-18, 1.599198204416119e-18, 0.027777777777777794, -0.016037507477489617, 2.5614276324970042e-18, 7.623296525288703e-18, -1.5341460740669888e-17], [-5.637851296924623e-18, -1.951563910473908e-18, -1.0842021724855044e-18, 4.336808689942018e-19, 6.505213034913027e-19, 2.8189256484623115e-18, 1.3010426069826053e-18, 0.0, 1.3010426069826053e-18, 0.0, -0.03726779962499649, -0.021516574145596754, 1.1275702593849246e-17, 2.1250362580715887e-17, 2.5804011705155006e-17, -4.7704895589362195e-18, -3.0357660829594124e-18, -2.168404344971009e-18, 0.0, 1.0842021724855044e-18, 0.027777777777777794, 0.016037507477489606, -1.2793585635328952e-17, -1.3227266504323154e-17, -2.3418766925686896e-17], [-3.2526065174565133e-18, 3.564314642046096e-18, -0.03726779962499649, -4.336808689942018e-18, 0.027777777777777797, 2.0464316005663896e-18, -3.279711571768651e-18, 0.021516574145596757, 1.9922214919421144e-18, -0.016037507477489617, -1.4230153513872246e-18, 4.0657581468206416e-19, -4.038653092508504e-18, 9.486769009248164e-19, -9.0801931945661e-19, 2.6020852139652106e-18, 6.369687763352339e-19, -8.158621347953421e-18, 1.7076184216646695e-18, 4.960224939121183e-18, -2.236166980751353e-19, 6.776263578034403e-20, 2.337810934421869e-17, -2.5682038960750386e-18, -1.1438332919722072e-17], [-2.6020852139652106e-18, 4.119968255444917e-18, -0.03726779962499649, -3.686287386450715e-18, 0.027777777777777794, -3.2526065174565133e-18, 3.0357660829594124e-18, -0.021516574145596757, -1.734723475976807e-18, 0.016037507477489606, -1.5178830414797062e-18, 8.673617379884035e-19, 8.239936510889834e-18, -8.673617379884035e-19, -1.4094628242311558e-17, -2.8189256484623115e-18, -2.6020852139652106e-18, 1.951563910473908e-17, 4.336808689942018e-19, -2.2551405187698492e-17, -1.8431436932253575e-18, -1.951563910473908e-18, 2.710505431213761e-17, -4.336808689942018e-19, -2.873135757086587e-17]], dtype=np.float64),
    [[np.array([[0.0, 0.0]], dtype=np.float64), np.array([[1.0, 0.0]], dtype=np.float64), np.array([[0.0, 1.0]], dtype=np.float64), np.array([[1.0, 1.0]], dtype=np.float64)], [np.array([[0.1127016653792583, 0.0], [0.5, 0.0], [0.8872983346207417, 0.0], [0.06943184420297371, 0.0], [0.33000947820757187, 0.0], [0.6699905217924281, 0.0], [0.9305681557970262, 0.0]], dtype=np.float64), np.array([[0.0, 0.1127016653792583], [0.0, 0.5], [0.0, 0.8872983346207417], [0.0, 0.06943184420297371], [0.0, 0.33000947820757187], [0.0, 0.6699905217924281], [0.0, 0.9305681557970262]], dtype=np.float64), np.array([[1.0, 0.1127016653792583], [1.0, 0.5], [1.0, 0.8872983346207417], [1.0, 0.06943184420297371], [1.0, 0.33000947820757187], [1.0, 0.6699905217924281], [1.0, 0.9305681557970262]], dtype=np.float64), np.array([[0.1127016653792583, 1.0], [0.5, 1.0], [0.8872983346207417, 1.0], [0.06943184420297371, 1.0], [0.33000947820757187, 1.0], [0.6699905217924281, 1.0], [0.9305681557970262, 1.0]], dtype=np.float64)], [np.array([[0.06943184420297371, 0.06943184420297371], [0.06943184420297371, 0.33000947820757187], [0.06943184420297371, 0.6699905217924281], [0.06943184420297371, 0.9305681557970262], [0.33000947820757187, 0.06943184420297371], [0.33000947820757187, 0.33000947820757187], [0.33000947820757187, 0.6699905217924281], [0.33000947820757187, 0.9305681557970262], [0.6699905217924281, 0.06943184420297371], [0.6699905217924281, 0.33000947820757187], [0.6699905217924281, 0.6699905217924281], [0.6699905217924281, 0.9305681557970262], [0.9305681557970262, 0.06943184420297371], [0.9305681557970262, 0.33000947820757187], [0.9305681557970262, 0.6699905217924281], [0.9305681557970262, 0.9305681557970262]], dtype=np.float64)], []],
    [[np.array([[[[1.0]]]], dtype=np.float64), np.array([[[[1.0]]]], dtype=np.float64), np.array([[[[1.0]]]], dtype=np.float64), np.array([[[[1.0]]]], dtype=np.float64)], [np.array([[[[0.2777777777777777], [0.4444444444444444], [0.2777777777777777], [0.0], [0.0], [0.0], [0.0]]], [[[0.06261203632181014], [0.4444444444444444], [0.49294351923374524], [0.0], [0.0], [0.0], [0.0]]], [[[0.0], [0.0], [0.0], [0.0025153980367775505], [0.10653403049014981], [0.4391095159686139], [0.45184105550445836]]]], dtype=np.float64), np.array([[[[0.2777777777777777], [0.4444444444444444], [0.2777777777777777], [0.0], [0.0], [0.0], [0.0]]], [[[0.06261203632181014], [0.4444444444444444], [0.49294351923374524], [0.0], [0.0], [0.0], [0.0]]], [[[0.0], [0.0], [0.0], [0.0025153980367775505], [0.10653403049014981], [0.4391095159686139], [0.45184105550445836]]]], dtype=np.float64), np.array([[[[0.2777777777777777], [0.4444444444444444], [0.2777777777777777], [0.0], [0.0], [0.0], [0.0]]], [[[0.06261203632181014], [0.4444444444444444], [0.49294351923374524], [0.0], [0.0], [0.0], [0.0]]], [[[0.0], [0.0], [0.0], [0.0025153980367775505], [0.10653403049014981], [0.4391095159686139], [0.45184105550445836]]]], dtype=np.float64), np.array([[[[0.2777777777777777], [0.4444444444444444], [0.2777777777777777], [0.0], [0.0], [0.0], [0.0]]], [[[0.06261203632181014], [0.4444444444444444], [0.49294351923374524], [0.0], [0.0], [0.0], [0.0]]], [[[0.0], [0.0], [0.0], [0.0025153980367775505], [0.10653403049014981], [0.4391095159686139], [0.45184105550445836]]]], dtype=np.float64)], [np.array([[[[-0.007818132048204512], [-0.03240740740740739], [-0.032407407407407385], [-0.007818132048204512], [-0.0324074074074074], [-0.09403371980364733], [-0.09403371980364732], [-0.032407407407407406], [-0.032407407407407385], [-0.09403371980364732], [-0.09403371980364728], [-0.03240740740740737], [-0.007818132048204512], [-0.03240740740740739], [-0.032407407407407385], [-0.007818132048204512]]], [[[0.002823411371443833], [-0.008203176844169028], [-0.024204230563238362], [-0.01064154341964835], [0.019346191766075816], [-0.015047177710813684], [-0.07898654209283365], [-0.051753599173483225], [0.019346191766075813], [-0.015047177710813674], [-0.0789865420928336], [-0.051753599173483184], [0.002823411371443832], [-0.008203176844169036], [-0.02420423056323838], [-0.010641543419648363]]], [[[0.002823411371443833], [0.019346191766075816], [0.019346191766075816], [0.002823411371443833], [-0.00820317684416903], [-0.015047177710813684], [-0.01504717771081368], [-0.00820317684416903], [-0.024204230563238362], [-0.07898654209283365], [-0.07898654209283362], [-0.024204230563238366], [-0.01064154341964835], [-0.051753599173483225], [-0.05175359917348321], [-0.010641543419648344]]], [[[0.00042975881927679167], [0.006557421280888858], [0.012788770485186958], [0.002393652552167052], [0.006557421280888859], [0.00030943780431633134], [-0.015356615515129996], [-0.01476059812505788], [0.012788770485186961], [-0.015356615515130003], [-0.06362992657770361], [-0.03699300104842533], [0.002393652552167046], [-0.01476059812505789], [-0.03699300104842534], [-0.013035195971815416]]]], dtype=np.float64)], []],
    0,
    basix.MapType.identity,
    basix.SobolevSpace.H1,
    False,
    -1,
    4,
    basix.PolysetType.standard, dtype=np.float64
)

# Create Tiniest tensor degree 2 on a hexahedron
e = basix.ufl.custom_element(
    basix.CellType.hexahedron,
    (),
    np.array([[0.9999999999999991, 0.0, -3.469446951953614e-17, -1.3877787807814457e-17, -2.7755575615628914e-17, 0.0, 3.122502256758253e-17, -1.3877787807814457e-17, -8.326672684688674e-17, 0.0, -6.938893903907228e-18, 0.0, -6.938893903907228e-18, 0.0, 0.0, -1.3877787807814457e-17, -2.7755575615628914e-17, 0.0, -5.204170427930421e-17, 0.0, -7.632783294297951e-17, -1.0408340855860843e-17, 0.0, -2.7755575615628914e-17, -9.367506770274758e-17, 0.0, -1.3877787807814457e-17], [0.49999999999999967, 0.28867513459481264, -6.938893903907228e-18, 1.3877787807814457e-17, 6.938893903907228e-18, 6.938893903907228e-18, -4.163336342344337e-17, 1.0408340855860843e-17, -4.5102810375396984e-17, 0.0, 6.938893903907228e-18, 0.0, -6.938893903907228e-18, 0.0, 0.0, -3.469446951953614e-18, -1.3877787807814457e-17, 0.0, -1.3877787807814457e-17, 1.0408340855860843e-17, -5.204170427930421e-17, 6.938893903907228e-18, 6.938893903907228e-18, -3.469446951953614e-18, -4.5102810375396984e-17, 2.42861286636753e-17, -3.642919299551295e-17], [0.49999999999999967, 0.0, -2.0816681711721685e-17, 0.28867513459481264, 0.0, -3.469446951953614e-18, -6.938893903907228e-18, 0.0, -3.469446951953614e-17, 1.3877787807814457e-17, -6.938893903907228e-18, 0.0, 0.0, 0.0, -3.469446951953614e-18, 1.0408340855860843e-17, -1.3877787807814457e-17, 0.0, -6.938893903907228e-18, -6.938893903907228e-18, -5.551115123125783e-17, -3.469446951953614e-18, 3.469446951953614e-18, 2.0816681711721685e-17, -3.8163916471489756e-17, 3.469446951953614e-18, -3.122502256758253e-17], [0.24999999999999983, 0.14433756729740632, -1.0408340855860843e-17, 0.14433756729740635, 0.08333333333333327, 6.938893903907228e-18, -5.204170427930421e-18, -3.469446951953614e-18, -1.3877787807814457e-17, 3.469446951953614e-18, 3.469446951953614e-18, -3.469446951953614e-18, -3.469446951953614e-18, 0.0, 0.0, -1.0408340855860843e-17, -6.938893903907228e-18, 0.0, -1.214306433183765e-17, 0.0, -2.2551405187698492e-17, -6.938893903907228e-18, 3.469446951953614e-18, 1.3877787807814457e-17, -2.2551405187698492e-17, 1.0408340855860843e-17, -1.5612511283791264e-17], [0.49999999999999967, 0.0, -1.3877787807814457e-17, -1.3877787807814457e-17, 0.0, 3.469446951953614e-18, -6.938893903907228e-18, 6.938893903907228e-18, -4.85722573273506e-17, 0.28867513459481264, 0.0, 6.938893903907228e-18, 0.0, 0.0, 3.469446951953614e-18, 1.0408340855860843e-17, -6.938893903907228e-18, 1.3877787807814457e-17, -6.938893903907228e-18, 6.938893903907228e-18, -4.85722573273506e-17, -3.469446951953614e-18, 0.0, 6.938893903907228e-18, -3.8163916471489756e-17, 0.0, -2.0816681711721685e-17], [0.24999999999999983, 0.14433756729740632, -8.673617379884035e-18, 3.469446951953614e-18, -3.469446951953614e-18, 0.0, -5.204170427930421e-18, 3.469446951953614e-18, -2.2551405187698492e-17, 0.14433756729740632, 0.08333333333333326, 6.938893903907228e-18, -3.469446951953614e-18, 0.0, -3.469446951953614e-18, 3.469446951953614e-18, -3.469446951953614e-18, 0.0, -8.673617379884035e-18, -6.938893903907228e-18, -1.9081958235744878e-17, 0.0, 3.469446951953614e-18, 3.469446951953614e-18, -2.6020852139652106e-17, 6.938893903907228e-18, -6.938893903907228e-18], [0.24999999999999983, 0.0, -8.673617379884035e-18, 0.14433756729740632, -6.938893903907228e-18, -6.938893903907228e-18, -1.734723475976807e-18, -3.469446951953614e-18, -2.2551405187698492e-17, 0.14433756729740632, 0.0, 6.938893903907228e-18, 0.08333333333333327, 0.0, 0.0, 3.469446951953614e-18, -3.469446951953614e-18, 1.0408340855860843e-17, -8.673617379884035e-18, -3.469446951953614e-18, -1.734723475976807e-17, -6.938893903907228e-18, 6.938893903907228e-18, 1.0408340855860843e-17, -6.938893903907228e-18, 0.0, -5.204170427930421e-18], [0.12499999999999992, 0.07216878364870316, -6.938893903907228e-18, 0.07216878364870316, 0.04166666666666664, 0.0, -5.204170427930421e-18, -3.469446951953614e-18, -1.0408340855860843e-17, 0.07216878364870316, 0.04166666666666664, 3.469446951953614e-18, 0.04166666666666664, 0.02405626121623439, 3.469446951953614e-18, 3.469446951953614e-18, -3.469446951953614e-18, -3.469446951953614e-18, -5.204170427930421e-18, -3.469446951953614e-18, -1.214306433183765e-17, -3.469446951953614e-18, 3.469446951953614e-18, 0.0, -1.0408340855860843e-17, 0.0, -3.469446951953614e-18], [-0.062499999999999965, 0.03608439182435158, 3.9302328752599536e-18, 0.036084391824351594, -0.02083333333333332, 4.404571325722362e-19, 7.318364664277155e-19, -3.889575293791747e-18, 4.424900116456465e-18, 4.946672411965114e-19, 2.168404344971009e-19, 4.404571325722362e-19, -5.421010862427522e-19, 7.318364664277155e-19, -2.303929616531697e-19, 1.0367683274392636e-18, -1.5178830414797062e-18, 4.404571325722362e-19, 0.027950849718747364, -0.01613743060919757, -1.6466320494623599e-18, -0.016137430609197565, 0.009316949906249124, -3.950561665994057e-18, -2.913793338554793e-19, -6.6542908336297835e-18, -7.284483346386983e-19], [-0.06249999999999996, -0.03608439182435158, 2.8731357570865868e-18, 0.036084391824351594, 0.02083333333333332, 4.336808689942018e-19, 4.174178364069192e-18, 3.848917712323541e-18, 2.710505431213761e-18, -3.7947076036992655e-19, -2.168404344971009e-19, 0.0, 0.0, -4.336808689942018e-19, -1.0842021724855044e-19, 1.951563910473908e-18, -2.168404344971009e-19, 0.0, 0.02795084971874736, 0.01613743060919757, -7.589415207398531e-19, -0.01613743060919756, -0.009316949906249124, -1.3552527156068805e-18, 3.144186300207963e-18, 1.4094628242311558e-18, -1.1655173354219173e-18], [-0.062499999999999965, 0.03608439182435159, 3.3610267347050637e-18, -0.03608439182435158, 0.02083333333333332, 2.710505431213761e-19, 1.1926223897340549e-18, -2.656295322589486e-18, 3.74049749507499e-18, 5.421010862427522e-20, -3.2526065174565133e-19, 2.710505431213761e-19, -1.1926223897340549e-18, 5.421010862427522e-19, -1.0842021724855044e-19, -1.6263032587282567e-19, 1.0842021724855044e-19, 5.421010862427522e-20, 0.027950849718747364, -0.016137430609197565, -1.463672932855431e-18, 0.01613743060919757, -0.009316949906249124, 3.0899761915836876e-18, 2.222614453595284e-18, -2.8731357570865868e-18, -2.7376104855258987e-18], [-0.06249999999999995, -0.03608439182435159, 3.469446951953614e-18, -0.03608439182435158, -0.020833333333333315, 0.0, 8.239936510889834e-18, 6.5052130349130266e-18, 3.469446951953614e-18, 0.0, 0.0, -4.336808689942018e-19, -8.673617379884035e-19, 8.673617379884035e-19, 8.673617379884035e-19, 1.3010426069826053e-18, 0.0, -4.336808689942018e-19, 0.02795084971874737, 0.01613743060919757, 1.3010426069826053e-18, 0.01613743060919757, 0.00931694990624912, 3.0357660829594124e-18, -3.0357660829594124e-18, 3.0357660829594124e-18, -8.673617379884035e-19], [-0.062499999999999965, 0.03608439182435158, 3.496552006265752e-18, -3.7269449679189215e-19, -6.505213034913027e-19, 6.776263578034403e-21, 0.027950849718747364, -0.01613743060919757, 1.8228149024912543e-18, 0.036084391824351594, -0.02083333333333332, 1.7414997395548415e-18, -9.75781955236954e-19, -4.607859233063394e-19, -1.2197274440461925e-19, -0.01613743060919756, 0.009316949906249124, -1.7279472123987727e-18, 2.4665599424045226e-18, -4.2012834183813297e-19, 7.026985330421676e-18, -5.827586677109586e-19, 2.0328790734103208e-19, -4.811147140404426e-19, -2.913793338554793e-19, -3.1848438816761693e-18, -2.4631718106155054e-18], [-0.062499999999999965, -0.03608439182435158, 2.222614453595284e-18, 4.87890977618477e-19, 1.0842021724855044e-18, 4.336808689942018e-19, 0.027950849718747364, 0.01613743060919757, 1.1384122811097797e-18, 0.036084391824351594, 0.02083333333333332, -1.734723475976807e-18, 0.0, -4.336808689942018e-19, -1.0842021724855044e-19, -0.01613743060919756, -0.009316949906249124, -4.553649124439119e-18, 1.5720931501039814e-18, 6.0173220572945496e-18, 4.445228907190568e-18, 1.1926223897340549e-18, 1.0842021724855044e-19, -5.421010862427522e-20, 1.4094628242311558e-18, 2.710505431213761e-18, -1.599198204416119e-18], [-0.062499999999999965, 0.03608439182435159, 2.927345865710862e-18, -8.131516293641283e-19, 5.421010862427522e-19, -5.963111948670274e-19, 0.02795084971874737, -0.016137430609197565, 2.0057740190981832e-18, -0.03608439182435159, 0.020833333333333322, 1.5720931501039814e-18, -1.1926223897340549e-18, -1.1926223897340549e-18, -1.0842021724855044e-19, 0.01613743060919757, -0.009316949906249122, 2.656295322589486e-18, 2.0599841277224584e-18, -4.391018798566293e-18, 5.908901840045999e-18, -5.421010862427522e-20, -1.0842021724855044e-19, 5.421010862427522e-20, 1.3552527156068805e-18, -5.041540102057596e-18, 2.981555974335137e-19], [-0.06249999999999996, -0.03608439182435159, 1.734723475976807e-18, 8.673617379884035e-19, 0.0, 0.0, 0.02795084971874737, 0.01613743060919756, 2.168404344971009e-18, -0.03608439182435159, -0.02083333333333332, -8.673617379884035e-19, -8.673617379884035e-19, -8.673617379884035e-19, 0.0, 0.01613743060919756, 0.009316949906249122, 3.0357660829594124e-18, 1.3010426069826053e-18, 3.903127820947816e-18, 4.7704895589362195e-18, -4.336808689942018e-19, 0.0, -8.673617379884035e-19, 2.168404344971009e-18, 2.168404344971009e-18, -2.6020852139652106e-18], [-0.062499999999999965, 4.336808689942018e-19, 0.027950849718747364, 0.03608439182435159, 6.505213034913027e-19, -0.016137430609197558, -2.270048298641525e-18, -4.336808689942018e-19, -4.0657581468206416e-20, 0.03608439182435159, -4.336808689942018e-19, -0.01613743060919756, -0.02083333333333332, 0.0, 0.009316949906249124, 2.0464316005663896e-18, -8.673617379884035e-19, -4.4858864886587746e-18, 3.1509625637859973e-18, 0.0, -4.0657581468206416e-20, -2.6291902682773483e-18, -3.2526065174565133e-19, 3.2932640989247197e-18, 4.512991542970912e-18, -4.336808689942018e-19, 3.5914196963582334e-19], [-0.06249999999999996, -4.336808689942018e-19, 0.02795084971874736, -0.03608439182435158, 0.0, 0.016137430609197565, 1.0842021724855044e-18, 0.0, 3.3610267347050637e-18, 0.036084391824351594, 0.0, -0.01613743060919756, 0.020833333333333322, 8.673617379884035e-19, -0.00931694990624912, 4.065758146820642e-18, 0.0, -3.0899761915836876e-18, 3.686287386450715e-18, -8.673617379884035e-19, 1.6263032587282567e-18, 1.734723475976807e-18, -2.168404344971009e-19, -1.3552527156068805e-18, 4.228388472693467e-18, 0.0, -6.2341624917916505e-19], [-0.06249999999999996, 0.0, 0.02795084971874736, 0.036084391824351594, 0.0, -0.016137430609197558, 6.288372600415926e-18, 1.734723475976807e-18, 1.6263032587282567e-18, -0.03608439182435158, 0.0, 0.016137430609197565, 0.02083333333333332, -8.673617379884035e-19, -0.00931694990624912, -2.0057740190981832e-18, 0.0, 2.5478751053409354e-18, 2.8189256484623115e-18, 0.0, 7.589415207398531e-19, -2.6020852139652106e-18, 0.0, -1.7889335846010823e-18, 5.095750210681871e-18, 4.336808689942018e-19, -1.4907779871675686e-18], [-0.06249999999999996, 0.0, 0.027950849718747367, -0.03608439182435159, 0.0, 0.01613743060919756, 5.637851296924623e-18, 4.336808689942018e-19, 4.336808689942018e-19, -0.03608439182435159, 0.0, 0.01613743060919756, -0.020833333333333322, 0.0, 0.009316949906249122, -3.0357660829594124e-18, -8.673617379884035e-19, 4.7704895589362195e-18, 2.168404344971009e-18, 0.0, 4.336808689942018e-19, 3.0357660829594124e-18, 0.0, 1.3010426069826053e-18, 7.37257477290143e-18, 0.0, -1.5178830414797062e-18]], dtype=np.float64),
    [[np.array([[0.0, 0.0, 0.0]], dtype=np.float64), np.array([[1.0, 0.0, 0.0]], dtype=np.float64), np.array([[0.0, 1.0, 0.0]], dtype=np.float64), np.array([[1.0, 1.0, 0.0]], dtype=np.float64), np.array([[0.0, 0.0, 1.0]], dtype=np.float64), np.array([[1.0, 0.0, 1.0]], dtype=np.float64), np.array([[0.0, 1.0, 1.0]], dtype=np.float64), np.array([[1.0, 1.0, 1.0]], dtype=np.float64)], [np.array([[0.21132486540518713, 0.0, 0.0], [0.7886751345948129, 0.0, 0.0]], dtype=np.float64), np.array([[0.0, 0.21132486540518713, 0.0], [0.0, 0.7886751345948129, 0.0]], dtype=np.float64), np.array([[0.0, 0.0, 0.21132486540518713], [0.0, 0.0, 0.7886751345948129]], dtype=np.float64), np.array([[1.0, 0.21132486540518713, 0.0], [1.0, 0.7886751345948129, 0.0]], dtype=np.float64), np.array([[1.0, 0.0, 0.21132486540518713], [1.0, 0.0, 0.7886751345948129]], dtype=np.float64), np.array([[0.21132486540518713, 1.0, 0.0], [0.7886751345948129, 1.0, 0.0]], dtype=np.float64), np.array([[0.0, 1.0, 0.21132486540518713], [0.0, 1.0, 0.7886751345948129]], dtype=np.float64), np.array([[1.0, 1.0, 0.21132486540518713], [1.0, 1.0, 0.7886751345948129]], dtype=np.float64), np.array([[0.21132486540518713, 0.0, 1.0], [0.7886751345948129, 0.0, 1.0]], dtype=np.float64), np.array([[0.0, 0.21132486540518713, 1.0], [0.0, 0.7886751345948129, 1.0]], dtype=np.float64), np.array([[1.0, 0.21132486540518713, 1.0], [1.0, 0.7886751345948129, 1.0]], dtype=np.float64), np.array([[0.21132486540518713, 1.0, 1.0], [0.7886751345948129, 1.0, 1.0]], dtype=np.float64)], [np.empty((0, 3), dtype=np.float64), np.empty((0, 3), dtype=np.float64), np.empty((0, 3), dtype=np.float64), np.empty((0, 3), dtype=np.float64), np.empty((0, 3), dtype=np.float64), np.empty((0, 3), dtype=np.float64)], [np.empty((0, 3), dtype=np.float64)]],
    [[np.array([[[[1.0]]]], dtype=np.float64), np.array([[[[1.0]]]], dtype=np.float64), np.array([[[[1.0]]]], dtype=np.float64), np.array([[[[1.0]]]], dtype=np.float64), np.array([[[[1.0]]]], dtype=np.float64), np.array([[[[1.0]]]], dtype=np.float64), np.array([[[[1.0]]]], dtype=np.float64), np.array([[[[1.0]]]], dtype=np.float64)], [np.array([[[[0.5], [0.5]]]], dtype=np.float64), np.array([[[[0.5], [0.5]]]], dtype=np.float64), np.array([[[[0.5], [0.5]]]], dtype=np.float64), np.array([[[[0.5], [0.5]]]], dtype=np.float64), np.array([[[[0.5], [0.5]]]], dtype=np.float64), np.array([[[[0.5], [0.5]]]], dtype=np.float64), np.array([[[[0.5], [0.5]]]], dtype=np.float64), np.array([[[[0.5], [0.5]]]], dtype=np.float64), np.array([[[[0.5], [0.5]]]], dtype=np.float64), np.array([[[[0.5], [0.5]]]], dtype=np.float64), np.array([[[[0.5], [0.5]]]], dtype=np.float64), np.array([[[[0.5], [0.5]]]], dtype=np.float64)], [np.empty((0, 1, 0, 1), dtype=np.float64), np.empty((0, 1, 0, 1), dtype=np.float64), np.empty((0, 1, 0, 1), dtype=np.float64), np.empty((0, 1, 0, 1), dtype=np.float64), np.empty((0, 1, 0, 1), dtype=np.float64), np.empty((0, 1, 0, 1), dtype=np.float64)], [np.empty((0, 1, 0, 1), dtype=np.float64)]],
    0,
    basix.MapType.identity,
    basix.SobolevSpace.H1,
    False,
    -1,
    2,
    basix.PolysetType.standard, dtype=np.float64
)

Symfem

"TNT"
↓ Show Symfem examples ↓ This implementation is used to compute the examples below and verify other implementations.

Examples

quadrilateral degree 2	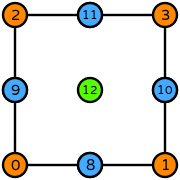(click to view basis functions)
quadrilateral degree 3	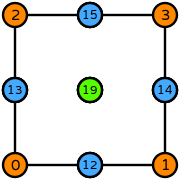(click to view basis functions)
quadrilateral degree 4	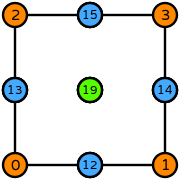(click to view basis functions)
hexahedron degree 2	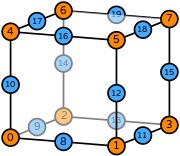(click to view basis functions)

References

[1] Cockburn, Bernardo and Qiu, Weifeng. Commuting diagrams for the TNT elements on cubes, Mathematics of Computation 83, 603–633, 2014. [DOI: 10.1090/S0025-5718-2013-02729-9] [BibTeX]
[2] Cockburn, Bernardo and Fu, Guosheng. A systematic construction of finite element commuting exact sequences, SIAM journal on numerical analysis 55, 1650–1688, 2017. [DOI: 10.1137/16M1073352] [BibTeX]

DefElement stats

Element added	24 October 2021
Element last updated	04 June 2025