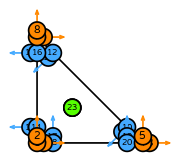
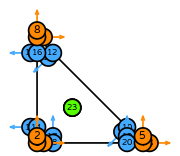
Click here to read what the information on this page means.
| Alternative names | conforming Arnold–Winther |
| Degrees | \(2\leqslant k\)
where \(k\) is the Polynomial subdegree |
| Polynomial subdegree | \(k\) |
| Polynomial superdegree | \(k+1\) |
| Reference elements | triangle |
| Polynomial set | \(\mathcal{Z}^{(2)}_{k} \oplus \mathcal{Z}^{(3)}_{k+1}\)
↓ Show polynomial set definitions ↓↑ Hide polynomial set definitions ↑\(\mathcal{Z}^{(2)}_k=\left\{\mathbf{M}\in\mathcal{P}_{k}^{d\times d}\middle|\mathbf{M}^t=\mathbf{M}\right\}\)
\(\mathcal{P}_k=\operatorname{span}\left\{\prod_{i=1}^dx_i^{p_i}\middle|\sum_{i=1}^dp_i\leqslant k\right\}\)
\(\mathcal{Z}^{(3)}_k=\left\{\mathbf{M}\in\mathcal{P}_{k}^{d\times d}\middle|\mathbf{M}^t=\mathbf{M}\text{ and }\operatorname{div}\mathbf{M}=0\right\}\) |
| DOFs | On each vertex: point evaluations of three components
On each edge: integral moments of normal-normal and normal-tangent inner products with a degree \(k-1\) Lagrange space
On each face: integral moments of three components with a degree \(k-2\) Lagrange space, and integral moments of tensor dot product with \(\frac{\partial}{\partial(x, y)}x^2y^2(1-x-y)^2f\) for each degree \(k-3\) polynomial \(f\) in a degree \(k-3\) Lagrange space |
| Number of DOFs | triangle: \((3k^2+11k+14)/2\) |
| Mapping | double contravariant Piola |
| continuity | Inner products with normals to facets are continuous |
| Categories | Matrix-valued elements |
This element is implemented in
FIAT ,
Symfem , and
(legacy) UFL.
↓ Show implementation detail ↓↑ Hide implementation detail ↑| FIAT | FIAT.ArnoldWinther
↓ Show FIAT examples ↓↑ Hide FIAT examples ↑Before running this example, you must install FIAT: pip3 install git+https://github.com/firedrakeproject/fiat.git This element can then be created with the following lines of Python: import FIAT
# Create Arnold-Winther degree 2
element = FIAT.ArnoldWinther(FIAT.ufc_cell("triangle"), 3) Note: This implementation includes additional DOFs that are used then filtered out when mapping the element, as described in Kirby (2018). |
| Symfem | "AW"
↓ Show Symfem examples ↓↑ Hide Symfem examples ↑Before running this example, you must install Symfem: pip3 install symfem This element can then be created with the following lines of Python: import symfem
# Create Arnold-Winther degree 2 on a triangle
element = symfem.create_element("triangle", "AW", 2)
# Create Arnold-Winther degree 3 on a triangle
element = symfem.create_element("triangle", "AW", 3) |
| (legacy) UFL | "AWc"
↓ Show (legacy) UFL examples ↓↑ Hide (legacy) UFL examples ↑Before running this example, you must install (legacy) UFL: pip3 install setuptools
pip3 install fenics-ufl-legacy This element can then be created with the following lines of Python: import ufl_legacy
# Create Arnold-Winther degree 2 on a triangle
element = ufl_legacy.FiniteElement("AWc", "triangle", 3)
# Create Arnold-Winther degree 3 on a triangle
element = ufl_legacy.FiniteElement("AWc", "triangle", 4) |
- Arnold, Douglas N. and Winther, Ragnar. Mixed finite elements for elasticity, Numerische Mathematik 92(3), 401–419, 2002. [DOI: 10.1007/s002110100348] [BibTeX]
- Kirby, Robert C. A general approach to transforming finite elements, SMAI Journal of Computational Mathematics 4, 197–224, 2018. [DOI: 10.5802/smai-jcm.33] [BibTeX]
| Element added | 10 February 2021 |
| Element last updated | 31 March 2025 |
