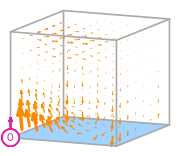
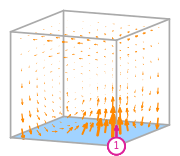
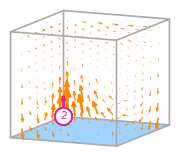
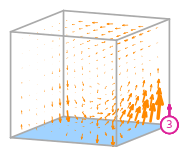
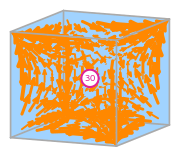
an encyclopedia of finite element definitions
Degree 1 Tiniest tensor H(div) on a hexahedron
◀ Back to Tiniest tensor H(div) definition page
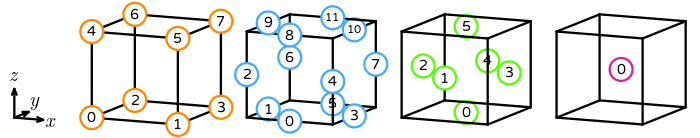
- \(R\) is the reference hexahedron. The following numbering of the subentities of the reference cell is used:
- \(\mathcal{V}\) is spanned by: \(\left(\begin{array}{c}\displaystyle 1\\\displaystyle 0\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle 1\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 1\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle z\\\displaystyle 0\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle z\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle z\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle y\\\displaystyle 0\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle y\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle y\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle y z\\\displaystyle 0\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle y z\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle y z\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle x\\\displaystyle 0\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle x\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle x\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle x z\\\displaystyle 0\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle x z\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle x z\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle x y\\\displaystyle 0\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle x y\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle x y\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle x y z\\\displaystyle 0\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle x y z\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle x y z\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle \frac{3 z \left(z - 1\right)}{2}\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle \frac{3 y \left(y - 1\right)}{2}\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle \frac{3 y \left(- 2 y z + y + 2 z - 1\right)}{2}\\\displaystyle \frac{3 z \left(- 2 y z + 2 y + z - 1\right)}{2}\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle \frac{3 x \left(x - 1\right)}{2}\\\displaystyle 0\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle \frac{3 x \left(- 2 x z + x + 2 z - 1\right)}{2}\\\displaystyle 0\\\displaystyle \frac{3 z \left(- 2 x z + 2 x + z - 1\right)}{2}\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle \frac{3 x \left(- 2 x y + x + 2 y - 1\right)}{2}\\\displaystyle \frac{3 y \left(- 2 x y + 2 x + y - 1\right)}{2}\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle \frac{3 x \left(4 x y z - 2 x y - 2 x z + x - 4 y z + 2 y + 2 z - 1\right)}{2}\\\displaystyle \frac{3 y \left(4 x y z - 2 x y - 4 x z + 2 x - 2 y z + y + 2 z - 1\right)}{2}\\\displaystyle \frac{3 z \left(4 x y z - 4 x y - 2 x z + 2 x - 2 y z + 2 y + z - 1\right)}{2}\end{array}\right)\)
- \(\mathcal{L}=\{l_0,...,l_{30}\}\)
- Functionals and basis functions:
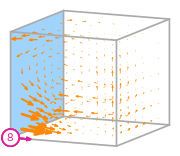
\(\displaystyle l_{0}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{0}}\boldsymbol{v}\cdot(s_{0} s_{1} - s_{0} - s_{1} + 1)\hat{\boldsymbol{n}}_{0}\)
where \(f_{0}\) is the 0th face;
\(\hat{\boldsymbol{n}}_{0}\) is the normal to facet 0;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{0}\).
\(\displaystyle \boldsymbol{\phi}_{0} = \left(\begin{array}{c}\displaystyle \frac{9 x \left(8 x y z - 4 x y - 6 x z + 3 x - 8 y z + 4 y + 6 z - 3\right)}{2}\\\displaystyle \frac{9 y \left(8 x y z - 4 x y - 8 x z + 4 x - 6 y z + 3 y + 6 z - 3\right)}{2}\\\displaystyle 36 x y z^{2} - 72 x y z + 36 x y - 27 x z^{2} + 51 x z - 24 x - 27 y z^{2} + 51 y z - 24 y + 21 z^{2} - 37 z + 16\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
where \(f_{0}\) is the 0th face;
\(\hat{\boldsymbol{n}}_{0}\) is the normal to facet 0;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{0}\).
\(\displaystyle \boldsymbol{\phi}_{0} = \left(\begin{array}{c}\displaystyle \frac{9 x \left(8 x y z - 4 x y - 6 x z + 3 x - 8 y z + 4 y + 6 z - 3\right)}{2}\\\displaystyle \frac{9 y \left(8 x y z - 4 x y - 8 x z + 4 x - 6 y z + 3 y + 6 z - 3\right)}{2}\\\displaystyle 36 x y z^{2} - 72 x y z + 36 x y - 27 x z^{2} + 51 x z - 24 x - 27 y z^{2} + 51 y z - 24 y + 21 z^{2} - 37 z + 16\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
\(\displaystyle l_{1}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{0}}\boldsymbol{v}\cdot(s_{0} \left(1 - s_{1}\right))\hat{\boldsymbol{n}}_{0}\)
where \(f_{0}\) is the 0th face;
\(\hat{\boldsymbol{n}}_{0}\) is the normal to facet 0;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{0}\).
\(\displaystyle \boldsymbol{\phi}_{1} = \left(\begin{array}{c}\displaystyle \frac{9 x \left(- 8 x y z + 4 x y + 6 x z - 3 x + 8 y z - 4 y - 6 z + 3\right)}{2}\\\displaystyle \frac{9 y \left(- 8 x y z + 4 x y + 8 x z - 4 x + 2 y z - y - 2 z + 1\right)}{2}\\\displaystyle - 36 x y z^{2} + 72 x y z - 36 x y + 27 x z^{2} - 51 x z + 24 x + 9 y z^{2} - 21 y z + 12 y - 6 z^{2} + 14 z - 8\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
where \(f_{0}\) is the 0th face;
\(\hat{\boldsymbol{n}}_{0}\) is the normal to facet 0;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{0}\).
\(\displaystyle \boldsymbol{\phi}_{1} = \left(\begin{array}{c}\displaystyle \frac{9 x \left(- 8 x y z + 4 x y + 6 x z - 3 x + 8 y z - 4 y - 6 z + 3\right)}{2}\\\displaystyle \frac{9 y \left(- 8 x y z + 4 x y + 8 x z - 4 x + 2 y z - y - 2 z + 1\right)}{2}\\\displaystyle - 36 x y z^{2} + 72 x y z - 36 x y + 27 x z^{2} - 51 x z + 24 x + 9 y z^{2} - 21 y z + 12 y - 6 z^{2} + 14 z - 8\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
\(\displaystyle l_{2}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{0}}\boldsymbol{v}\cdot(s_{1} \left(1 - s_{0}\right))\hat{\boldsymbol{n}}_{0}\)
where \(f_{0}\) is the 0th face;
\(\hat{\boldsymbol{n}}_{0}\) is the normal to facet 0;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{0}\).
\(\displaystyle \boldsymbol{\phi}_{2} = \left(\begin{array}{c}\displaystyle \frac{9 x \left(- 8 x y z + 4 x y + 2 x z - x + 8 y z - 4 y - 2 z + 1\right)}{2}\\\displaystyle \frac{9 y \left(- 8 x y z + 4 x y + 8 x z - 4 x + 6 y z - 3 y - 6 z + 3\right)}{2}\\\displaystyle - 36 x y z^{2} + 72 x y z - 36 x y + 9 x z^{2} - 21 x z + 12 x + 27 y z^{2} - 51 y z + 24 y - 6 z^{2} + 14 z - 8\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
where \(f_{0}\) is the 0th face;
\(\hat{\boldsymbol{n}}_{0}\) is the normal to facet 0;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{0}\).
\(\displaystyle \boldsymbol{\phi}_{2} = \left(\begin{array}{c}\displaystyle \frac{9 x \left(- 8 x y z + 4 x y + 2 x z - x + 8 y z - 4 y - 2 z + 1\right)}{2}\\\displaystyle \frac{9 y \left(- 8 x y z + 4 x y + 8 x z - 4 x + 6 y z - 3 y - 6 z + 3\right)}{2}\\\displaystyle - 36 x y z^{2} + 72 x y z - 36 x y + 9 x z^{2} - 21 x z + 12 x + 27 y z^{2} - 51 y z + 24 y - 6 z^{2} + 14 z - 8\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
\(\displaystyle l_{3}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{0}}\boldsymbol{v}\cdot(s_{0} s_{1})\hat{\boldsymbol{n}}_{0}\)
where \(f_{0}\) is the 0th face;
\(\hat{\boldsymbol{n}}_{0}\) is the normal to facet 0;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{0}\).
\(\displaystyle \boldsymbol{\phi}_{3} = \left(\begin{array}{c}\displaystyle \frac{9 x \left(8 x y z - 4 x y - 2 x z + x - 8 y z + 4 y + 2 z - 1\right)}{2}\\\displaystyle \frac{9 y \left(8 x y z - 4 x y - 8 x z + 4 x - 2 y z + y + 2 z - 1\right)}{2}\\\displaystyle 36 x y z^{2} - 72 x y z + 36 x y - 9 x z^{2} + 21 x z - 12 x - 9 y z^{2} + 21 y z - 12 y + 3 z^{2} - 7 z + 4\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
where \(f_{0}\) is the 0th face;
\(\hat{\boldsymbol{n}}_{0}\) is the normal to facet 0;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{0}\).
\(\displaystyle \boldsymbol{\phi}_{3} = \left(\begin{array}{c}\displaystyle \frac{9 x \left(8 x y z - 4 x y - 2 x z + x - 8 y z + 4 y + 2 z - 1\right)}{2}\\\displaystyle \frac{9 y \left(8 x y z - 4 x y - 8 x z + 4 x - 2 y z + y + 2 z - 1\right)}{2}\\\displaystyle 36 x y z^{2} - 72 x y z + 36 x y - 9 x z^{2} + 21 x z - 12 x - 9 y z^{2} + 21 y z - 12 y + 3 z^{2} - 7 z + 4\end{array}\right)\)
This DOF is associated with face 0 of the reference cell.
\(\displaystyle l_{4}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{1}}\boldsymbol{v}\cdot(s_{0} s_{1} - s_{0} - s_{1} + 1)\hat{\boldsymbol{n}}_{1}\)
where \(f_{1}\) is the 1st face;
\(\hat{\boldsymbol{n}}_{1}\) is the normal to facet 1;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{1}\).
\(\displaystyle \boldsymbol{\phi}_{4} = \left(\begin{array}{c}\displaystyle \frac{9 x \left(- 8 x y z + 6 x y + 4 x z - 3 x + 8 y z - 6 y - 4 z + 3\right)}{2}\\\displaystyle - 36 x y^{2} z + 27 x y^{2} + 72 x y z - 51 x y - 36 x z + 24 x + 27 y^{2} z - 21 y^{2} - 51 y z + 37 y + 24 z - 16\\\displaystyle \frac{9 z \left(- 8 x y z + 8 x y + 4 x z - 4 x + 6 y z - 6 y - 3 z + 3\right)}{2}\end{array}\right)\)
This DOF is associated with face 1 of the reference cell.
where \(f_{1}\) is the 1st face;
\(\hat{\boldsymbol{n}}_{1}\) is the normal to facet 1;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{1}\).
\(\displaystyle \boldsymbol{\phi}_{4} = \left(\begin{array}{c}\displaystyle \frac{9 x \left(- 8 x y z + 6 x y + 4 x z - 3 x + 8 y z - 6 y - 4 z + 3\right)}{2}\\\displaystyle - 36 x y^{2} z + 27 x y^{2} + 72 x y z - 51 x y - 36 x z + 24 x + 27 y^{2} z - 21 y^{2} - 51 y z + 37 y + 24 z - 16\\\displaystyle \frac{9 z \left(- 8 x y z + 8 x y + 4 x z - 4 x + 6 y z - 6 y - 3 z + 3\right)}{2}\end{array}\right)\)
This DOF is associated with face 1 of the reference cell.
\(\displaystyle l_{5}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{1}}\boldsymbol{v}\cdot(s_{0} \left(1 - s_{1}\right))\hat{\boldsymbol{n}}_{1}\)
where \(f_{1}\) is the 1st face;
\(\hat{\boldsymbol{n}}_{1}\) is the normal to facet 1;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{1}\).
\(\displaystyle \boldsymbol{\phi}_{5} = \left(\begin{array}{c}\displaystyle \frac{9 x \left(8 x y z - 6 x y - 4 x z + 3 x - 8 y z + 6 y + 4 z - 3\right)}{2}\\\displaystyle 36 x y^{2} z - 27 x y^{2} - 72 x y z + 51 x y + 36 x z - 24 x - 9 y^{2} z + 6 y^{2} + 21 y z - 14 y - 12 z + 8\\\displaystyle \frac{9 z \left(8 x y z - 8 x y - 4 x z + 4 x - 2 y z + 2 y + z - 1\right)}{2}\end{array}\right)\)
This DOF is associated with face 1 of the reference cell.
where \(f_{1}\) is the 1st face;
\(\hat{\boldsymbol{n}}_{1}\) is the normal to facet 1;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{1}\).
\(\displaystyle \boldsymbol{\phi}_{5} = \left(\begin{array}{c}\displaystyle \frac{9 x \left(8 x y z - 6 x y - 4 x z + 3 x - 8 y z + 6 y + 4 z - 3\right)}{2}\\\displaystyle 36 x y^{2} z - 27 x y^{2} - 72 x y z + 51 x y + 36 x z - 24 x - 9 y^{2} z + 6 y^{2} + 21 y z - 14 y - 12 z + 8\\\displaystyle \frac{9 z \left(8 x y z - 8 x y - 4 x z + 4 x - 2 y z + 2 y + z - 1\right)}{2}\end{array}\right)\)
This DOF is associated with face 1 of the reference cell.
\(\displaystyle l_{6}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{1}}\boldsymbol{v}\cdot(s_{1} \left(1 - s_{0}\right))\hat{\boldsymbol{n}}_{1}\)
where \(f_{1}\) is the 1st face;
\(\hat{\boldsymbol{n}}_{1}\) is the normal to facet 1;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{1}\).
\(\displaystyle \boldsymbol{\phi}_{6} = \left(\begin{array}{c}\displaystyle \frac{9 x \left(8 x y z - 2 x y - 4 x z + x - 8 y z + 2 y + 4 z - 1\right)}{2}\\\displaystyle 36 x y^{2} z - 9 x y^{2} - 72 x y z + 21 x y + 36 x z - 12 x - 27 y^{2} z + 6 y^{2} + 51 y z - 14 y - 24 z + 8\\\displaystyle \frac{9 z \left(8 x y z - 8 x y - 4 x z + 4 x - 6 y z + 6 y + 3 z - 3\right)}{2}\end{array}\right)\)
This DOF is associated with face 1 of the reference cell.
where \(f_{1}\) is the 1st face;
\(\hat{\boldsymbol{n}}_{1}\) is the normal to facet 1;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{1}\).
\(\displaystyle \boldsymbol{\phi}_{6} = \left(\begin{array}{c}\displaystyle \frac{9 x \left(8 x y z - 2 x y - 4 x z + x - 8 y z + 2 y + 4 z - 1\right)}{2}\\\displaystyle 36 x y^{2} z - 9 x y^{2} - 72 x y z + 21 x y + 36 x z - 12 x - 27 y^{2} z + 6 y^{2} + 51 y z - 14 y - 24 z + 8\\\displaystyle \frac{9 z \left(8 x y z - 8 x y - 4 x z + 4 x - 6 y z + 6 y + 3 z - 3\right)}{2}\end{array}\right)\)
This DOF is associated with face 1 of the reference cell.
\(\displaystyle l_{7}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{1}}\boldsymbol{v}\cdot(s_{0} s_{1})\hat{\boldsymbol{n}}_{1}\)
where \(f_{1}\) is the 1st face;
\(\hat{\boldsymbol{n}}_{1}\) is the normal to facet 1;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{1}\).
\(\displaystyle \boldsymbol{\phi}_{7} = \left(\begin{array}{c}\displaystyle \frac{9 x \left(- 8 x y z + 2 x y + 4 x z - x + 8 y z - 2 y - 4 z + 1\right)}{2}\\\displaystyle - 36 x y^{2} z + 9 x y^{2} + 72 x y z - 21 x y - 36 x z + 12 x + 9 y^{2} z - 3 y^{2} - 21 y z + 7 y + 12 z - 4\\\displaystyle \frac{9 z \left(- 8 x y z + 8 x y + 4 x z - 4 x + 2 y z - 2 y - z + 1\right)}{2}\end{array}\right)\)
This DOF is associated with face 1 of the reference cell.
where \(f_{1}\) is the 1st face;
\(\hat{\boldsymbol{n}}_{1}\) is the normal to facet 1;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{1}\).
\(\displaystyle \boldsymbol{\phi}_{7} = \left(\begin{array}{c}\displaystyle \frac{9 x \left(- 8 x y z + 2 x y + 4 x z - x + 8 y z - 2 y - 4 z + 1\right)}{2}\\\displaystyle - 36 x y^{2} z + 9 x y^{2} + 72 x y z - 21 x y - 36 x z + 12 x + 9 y^{2} z - 3 y^{2} - 21 y z + 7 y + 12 z - 4\\\displaystyle \frac{9 z \left(- 8 x y z + 8 x y + 4 x z - 4 x + 2 y z - 2 y - z + 1\right)}{2}\end{array}\right)\)
This DOF is associated with face 1 of the reference cell.
\(\displaystyle l_{8}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{2}}\boldsymbol{v}\cdot(s_{0} s_{1} - s_{0} - s_{1} + 1)\hat{\boldsymbol{n}}_{2}\)
where \(f_{2}\) is the 2nd face;
\(\hat{\boldsymbol{n}}_{2}\) is the normal to facet 2;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{2}\).
\(\displaystyle \boldsymbol{\phi}_{8} = \left(\begin{array}{c}\displaystyle 36 x^{2} y z - 27 x^{2} y - 27 x^{2} z + 21 x^{2} - 72 x y z + 51 x y + 51 x z - 37 x + 36 y z - 24 y - 24 z + 16\\\displaystyle \frac{9 y \left(8 x y z - 6 x y - 8 x z + 6 x - 4 y z + 3 y + 4 z - 3\right)}{2}\\\displaystyle \frac{9 z \left(8 x y z - 8 x y - 6 x z + 6 x - 4 y z + 4 y + 3 z - 3\right)}{2}\end{array}\right)\)
This DOF is associated with face 2 of the reference cell.
where \(f_{2}\) is the 2nd face;
\(\hat{\boldsymbol{n}}_{2}\) is the normal to facet 2;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{2}\).
\(\displaystyle \boldsymbol{\phi}_{8} = \left(\begin{array}{c}\displaystyle 36 x^{2} y z - 27 x^{2} y - 27 x^{2} z + 21 x^{2} - 72 x y z + 51 x y + 51 x z - 37 x + 36 y z - 24 y - 24 z + 16\\\displaystyle \frac{9 y \left(8 x y z - 6 x y - 8 x z + 6 x - 4 y z + 3 y + 4 z - 3\right)}{2}\\\displaystyle \frac{9 z \left(8 x y z - 8 x y - 6 x z + 6 x - 4 y z + 4 y + 3 z - 3\right)}{2}\end{array}\right)\)
This DOF is associated with face 2 of the reference cell.
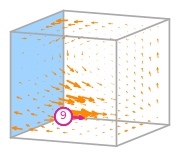
\(\displaystyle l_{9}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{2}}\boldsymbol{v}\cdot(s_{0} \left(1 - s_{1}\right))\hat{\boldsymbol{n}}_{2}\)
where \(f_{2}\) is the 2nd face;
\(\hat{\boldsymbol{n}}_{2}\) is the normal to facet 2;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{2}\).
\(\displaystyle \boldsymbol{\phi}_{9} = \left(\begin{array}{c}\displaystyle - 36 x^{2} y z + 27 x^{2} y + 9 x^{2} z - 6 x^{2} + 72 x y z - 51 x y - 21 x z + 14 x - 36 y z + 24 y + 12 z - 8\\\displaystyle \frac{9 y \left(- 8 x y z + 6 x y + 8 x z - 6 x + 4 y z - 3 y - 4 z + 3\right)}{2}\\\displaystyle \frac{9 z \left(- 8 x y z + 8 x y + 2 x z - 2 x + 4 y z - 4 y - z + 1\right)}{2}\end{array}\right)\)
This DOF is associated with face 2 of the reference cell.
where \(f_{2}\) is the 2nd face;
\(\hat{\boldsymbol{n}}_{2}\) is the normal to facet 2;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{2}\).
\(\displaystyle \boldsymbol{\phi}_{9} = \left(\begin{array}{c}\displaystyle - 36 x^{2} y z + 27 x^{2} y + 9 x^{2} z - 6 x^{2} + 72 x y z - 51 x y - 21 x z + 14 x - 36 y z + 24 y + 12 z - 8\\\displaystyle \frac{9 y \left(- 8 x y z + 6 x y + 8 x z - 6 x + 4 y z - 3 y - 4 z + 3\right)}{2}\\\displaystyle \frac{9 z \left(- 8 x y z + 8 x y + 2 x z - 2 x + 4 y z - 4 y - z + 1\right)}{2}\end{array}\right)\)
This DOF is associated with face 2 of the reference cell.
\(\displaystyle l_{10}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{2}}\boldsymbol{v}\cdot(s_{1} \left(1 - s_{0}\right))\hat{\boldsymbol{n}}_{2}\)
where \(f_{2}\) is the 2nd face;
\(\hat{\boldsymbol{n}}_{2}\) is the normal to facet 2;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{2}\).
\(\displaystyle \boldsymbol{\phi}_{10} = \left(\begin{array}{c}\displaystyle - 36 x^{2} y z + 9 x^{2} y + 27 x^{2} z - 6 x^{2} + 72 x y z - 21 x y - 51 x z + 14 x - 36 y z + 12 y + 24 z - 8\\\displaystyle \frac{9 y \left(- 8 x y z + 2 x y + 8 x z - 2 x + 4 y z - y - 4 z + 1\right)}{2}\\\displaystyle \frac{9 z \left(- 8 x y z + 8 x y + 6 x z - 6 x + 4 y z - 4 y - 3 z + 3\right)}{2}\end{array}\right)\)
This DOF is associated with face 2 of the reference cell.
where \(f_{2}\) is the 2nd face;
\(\hat{\boldsymbol{n}}_{2}\) is the normal to facet 2;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{2}\).
\(\displaystyle \boldsymbol{\phi}_{10} = \left(\begin{array}{c}\displaystyle - 36 x^{2} y z + 9 x^{2} y + 27 x^{2} z - 6 x^{2} + 72 x y z - 21 x y - 51 x z + 14 x - 36 y z + 12 y + 24 z - 8\\\displaystyle \frac{9 y \left(- 8 x y z + 2 x y + 8 x z - 2 x + 4 y z - y - 4 z + 1\right)}{2}\\\displaystyle \frac{9 z \left(- 8 x y z + 8 x y + 6 x z - 6 x + 4 y z - 4 y - 3 z + 3\right)}{2}\end{array}\right)\)
This DOF is associated with face 2 of the reference cell.
\(\displaystyle l_{11}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{2}}\boldsymbol{v}\cdot(s_{0} s_{1})\hat{\boldsymbol{n}}_{2}\)
where \(f_{2}\) is the 2nd face;
\(\hat{\boldsymbol{n}}_{2}\) is the normal to facet 2;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{2}\).
\(\displaystyle \boldsymbol{\phi}_{11} = \left(\begin{array}{c}\displaystyle 36 x^{2} y z - 9 x^{2} y - 9 x^{2} z + 3 x^{2} - 72 x y z + 21 x y + 21 x z - 7 x + 36 y z - 12 y - 12 z + 4\\\displaystyle \frac{9 y \left(8 x y z - 2 x y - 8 x z + 2 x - 4 y z + y + 4 z - 1\right)}{2}\\\displaystyle \frac{9 z \left(8 x y z - 8 x y - 2 x z + 2 x - 4 y z + 4 y + z - 1\right)}{2}\end{array}\right)\)
This DOF is associated with face 2 of the reference cell.
where \(f_{2}\) is the 2nd face;
\(\hat{\boldsymbol{n}}_{2}\) is the normal to facet 2;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{2}\).
\(\displaystyle \boldsymbol{\phi}_{11} = \left(\begin{array}{c}\displaystyle 36 x^{2} y z - 9 x^{2} y - 9 x^{2} z + 3 x^{2} - 72 x y z + 21 x y + 21 x z - 7 x + 36 y z - 12 y - 12 z + 4\\\displaystyle \frac{9 y \left(8 x y z - 2 x y - 8 x z + 2 x - 4 y z + y + 4 z - 1\right)}{2}\\\displaystyle \frac{9 z \left(8 x y z - 8 x y - 2 x z + 2 x - 4 y z + 4 y + z - 1\right)}{2}\end{array}\right)\)
This DOF is associated with face 2 of the reference cell.
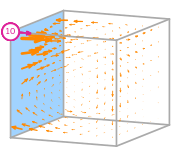
\(\displaystyle l_{12}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{3}}\boldsymbol{v}\cdot(s_{0} s_{1} - s_{0} - s_{1} + 1)\hat{\boldsymbol{n}}_{3}\)
where \(f_{3}\) is the 3rd face;
\(\hat{\boldsymbol{n}}_{3}\) is the normal to facet 3;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{3}\).
\(\displaystyle \boldsymbol{\phi}_{12} = \left(\begin{array}{c}\displaystyle x \left(36 x y z - 27 x y - 27 x z + 21 x + 3 y + 3 z - 5\right)\\\displaystyle \frac{9 y \left(8 x y z - 6 x y - 8 x z + 6 x - 4 y z + 3 y + 4 z - 3\right)}{2}\\\displaystyle \frac{9 z \left(8 x y z - 8 x y - 6 x z + 6 x - 4 y z + 4 y + 3 z - 3\right)}{2}\end{array}\right)\)
This DOF is associated with face 3 of the reference cell.
where \(f_{3}\) is the 3rd face;
\(\hat{\boldsymbol{n}}_{3}\) is the normal to facet 3;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{3}\).
\(\displaystyle \boldsymbol{\phi}_{12} = \left(\begin{array}{c}\displaystyle x \left(36 x y z - 27 x y - 27 x z + 21 x + 3 y + 3 z - 5\right)\\\displaystyle \frac{9 y \left(8 x y z - 6 x y - 8 x z + 6 x - 4 y z + 3 y + 4 z - 3\right)}{2}\\\displaystyle \frac{9 z \left(8 x y z - 8 x y - 6 x z + 6 x - 4 y z + 4 y + 3 z - 3\right)}{2}\end{array}\right)\)
This DOF is associated with face 3 of the reference cell.
\(\displaystyle l_{13}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{3}}\boldsymbol{v}\cdot(s_{0} \left(1 - s_{1}\right))\hat{\boldsymbol{n}}_{3}\)
where \(f_{3}\) is the 3rd face;
\(\hat{\boldsymbol{n}}_{3}\) is the normal to facet 3;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{3}\).
\(\displaystyle \boldsymbol{\phi}_{13} = \left(\begin{array}{c}\displaystyle x \left(- 36 x y z + 27 x y + 9 x z - 6 x - 3 y + 3 z - 2\right)\\\displaystyle \frac{9 y \left(- 8 x y z + 6 x y + 8 x z - 6 x + 4 y z - 3 y - 4 z + 3\right)}{2}\\\displaystyle \frac{9 z \left(- 8 x y z + 8 x y + 2 x z - 2 x + 4 y z - 4 y - z + 1\right)}{2}\end{array}\right)\)
This DOF is associated with face 3 of the reference cell.
where \(f_{3}\) is the 3rd face;
\(\hat{\boldsymbol{n}}_{3}\) is the normal to facet 3;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{3}\).
\(\displaystyle \boldsymbol{\phi}_{13} = \left(\begin{array}{c}\displaystyle x \left(- 36 x y z + 27 x y + 9 x z - 6 x - 3 y + 3 z - 2\right)\\\displaystyle \frac{9 y \left(- 8 x y z + 6 x y + 8 x z - 6 x + 4 y z - 3 y - 4 z + 3\right)}{2}\\\displaystyle \frac{9 z \left(- 8 x y z + 8 x y + 2 x z - 2 x + 4 y z - 4 y - z + 1\right)}{2}\end{array}\right)\)
This DOF is associated with face 3 of the reference cell.
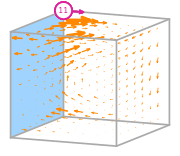
\(\displaystyle l_{14}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{3}}\boldsymbol{v}\cdot(s_{1} \left(1 - s_{0}\right))\hat{\boldsymbol{n}}_{3}\)
where \(f_{3}\) is the 3rd face;
\(\hat{\boldsymbol{n}}_{3}\) is the normal to facet 3;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{3}\).
\(\displaystyle \boldsymbol{\phi}_{14} = \left(\begin{array}{c}\displaystyle x \left(- 36 x y z + 9 x y + 27 x z - 6 x + 3 y - 3 z - 2\right)\\\displaystyle \frac{9 y \left(- 8 x y z + 2 x y + 8 x z - 2 x + 4 y z - y - 4 z + 1\right)}{2}\\\displaystyle \frac{9 z \left(- 8 x y z + 8 x y + 6 x z - 6 x + 4 y z - 4 y - 3 z + 3\right)}{2}\end{array}\right)\)
This DOF is associated with face 3 of the reference cell.
where \(f_{3}\) is the 3rd face;
\(\hat{\boldsymbol{n}}_{3}\) is the normal to facet 3;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{3}\).
\(\displaystyle \boldsymbol{\phi}_{14} = \left(\begin{array}{c}\displaystyle x \left(- 36 x y z + 9 x y + 27 x z - 6 x + 3 y - 3 z - 2\right)\\\displaystyle \frac{9 y \left(- 8 x y z + 2 x y + 8 x z - 2 x + 4 y z - y - 4 z + 1\right)}{2}\\\displaystyle \frac{9 z \left(- 8 x y z + 8 x y + 6 x z - 6 x + 4 y z - 4 y - 3 z + 3\right)}{2}\end{array}\right)\)
This DOF is associated with face 3 of the reference cell.
\(\displaystyle l_{15}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{3}}\boldsymbol{v}\cdot(s_{0} s_{1})\hat{\boldsymbol{n}}_{3}\)
where \(f_{3}\) is the 3rd face;
\(\hat{\boldsymbol{n}}_{3}\) is the normal to facet 3;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{3}\).
\(\displaystyle \boldsymbol{\phi}_{15} = \left(\begin{array}{c}\displaystyle x \left(36 x y z - 9 x y - 9 x z + 3 x - 3 y - 3 z + 1\right)\\\displaystyle \frac{9 y \left(8 x y z - 2 x y - 8 x z + 2 x - 4 y z + y + 4 z - 1\right)}{2}\\\displaystyle \frac{9 z \left(8 x y z - 8 x y - 2 x z + 2 x - 4 y z + 4 y + z - 1\right)}{2}\end{array}\right)\)
This DOF is associated with face 3 of the reference cell.
where \(f_{3}\) is the 3rd face;
\(\hat{\boldsymbol{n}}_{3}\) is the normal to facet 3;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{3}\).
\(\displaystyle \boldsymbol{\phi}_{15} = \left(\begin{array}{c}\displaystyle x \left(36 x y z - 9 x y - 9 x z + 3 x - 3 y - 3 z + 1\right)\\\displaystyle \frac{9 y \left(8 x y z - 2 x y - 8 x z + 2 x - 4 y z + y + 4 z - 1\right)}{2}\\\displaystyle \frac{9 z \left(8 x y z - 8 x y - 2 x z + 2 x - 4 y z + 4 y + z - 1\right)}{2}\end{array}\right)\)
This DOF is associated with face 3 of the reference cell.
\(\displaystyle l_{16}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{4}}\boldsymbol{v}\cdot(s_{0} s_{1} - s_{0} - s_{1} + 1)\hat{\boldsymbol{n}}_{4}\)
where \(f_{4}\) is the 4th face;
\(\hat{\boldsymbol{n}}_{4}\) is the normal to facet 4;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{4}\).
\(\displaystyle \boldsymbol{\phi}_{16} = \left(\begin{array}{c}\displaystyle \frac{9 x \left(- 8 x y z + 6 x y + 4 x z - 3 x + 8 y z - 6 y - 4 z + 3\right)}{2}\\\displaystyle y \left(- 36 x y z + 27 x y - 3 x + 27 y z - 21 y - 3 z + 5\right)\\\displaystyle \frac{9 z \left(- 8 x y z + 8 x y + 4 x z - 4 x + 6 y z - 6 y - 3 z + 3\right)}{2}\end{array}\right)\)
This DOF is associated with face 4 of the reference cell.
where \(f_{4}\) is the 4th face;
\(\hat{\boldsymbol{n}}_{4}\) is the normal to facet 4;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{4}\).
\(\displaystyle \boldsymbol{\phi}_{16} = \left(\begin{array}{c}\displaystyle \frac{9 x \left(- 8 x y z + 6 x y + 4 x z - 3 x + 8 y z - 6 y - 4 z + 3\right)}{2}\\\displaystyle y \left(- 36 x y z + 27 x y - 3 x + 27 y z - 21 y - 3 z + 5\right)\\\displaystyle \frac{9 z \left(- 8 x y z + 8 x y + 4 x z - 4 x + 6 y z - 6 y - 3 z + 3\right)}{2}\end{array}\right)\)
This DOF is associated with face 4 of the reference cell.
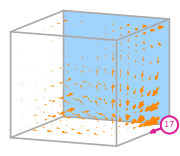
\(\displaystyle l_{17}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{4}}\boldsymbol{v}\cdot(s_{0} \left(1 - s_{1}\right))\hat{\boldsymbol{n}}_{4}\)
where \(f_{4}\) is the 4th face;
\(\hat{\boldsymbol{n}}_{4}\) is the normal to facet 4;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{4}\).
\(\displaystyle \boldsymbol{\phi}_{17} = \left(\begin{array}{c}\displaystyle \frac{9 x \left(8 x y z - 6 x y - 4 x z + 3 x - 8 y z + 6 y + 4 z - 3\right)}{2}\\\displaystyle y \left(36 x y z - 27 x y + 3 x - 9 y z + 6 y - 3 z + 2\right)\\\displaystyle \frac{9 z \left(8 x y z - 8 x y - 4 x z + 4 x - 2 y z + 2 y + z - 1\right)}{2}\end{array}\right)\)
This DOF is associated with face 4 of the reference cell.
where \(f_{4}\) is the 4th face;
\(\hat{\boldsymbol{n}}_{4}\) is the normal to facet 4;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{4}\).
\(\displaystyle \boldsymbol{\phi}_{17} = \left(\begin{array}{c}\displaystyle \frac{9 x \left(8 x y z - 6 x y - 4 x z + 3 x - 8 y z + 6 y + 4 z - 3\right)}{2}\\\displaystyle y \left(36 x y z - 27 x y + 3 x - 9 y z + 6 y - 3 z + 2\right)\\\displaystyle \frac{9 z \left(8 x y z - 8 x y - 4 x z + 4 x - 2 y z + 2 y + z - 1\right)}{2}\end{array}\right)\)
This DOF is associated with face 4 of the reference cell.
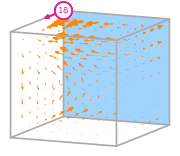
\(\displaystyle l_{18}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{4}}\boldsymbol{v}\cdot(s_{1} \left(1 - s_{0}\right))\hat{\boldsymbol{n}}_{4}\)
where \(f_{4}\) is the 4th face;
\(\hat{\boldsymbol{n}}_{4}\) is the normal to facet 4;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{4}\).
\(\displaystyle \boldsymbol{\phi}_{18} = \left(\begin{array}{c}\displaystyle \frac{9 x \left(8 x y z - 2 x y - 4 x z + x - 8 y z + 2 y + 4 z - 1\right)}{2}\\\displaystyle y \left(36 x y z - 9 x y - 3 x - 27 y z + 6 y + 3 z + 2\right)\\\displaystyle \frac{9 z \left(8 x y z - 8 x y - 4 x z + 4 x - 6 y z + 6 y + 3 z - 3\right)}{2}\end{array}\right)\)
This DOF is associated with face 4 of the reference cell.
where \(f_{4}\) is the 4th face;
\(\hat{\boldsymbol{n}}_{4}\) is the normal to facet 4;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{4}\).
\(\displaystyle \boldsymbol{\phi}_{18} = \left(\begin{array}{c}\displaystyle \frac{9 x \left(8 x y z - 2 x y - 4 x z + x - 8 y z + 2 y + 4 z - 1\right)}{2}\\\displaystyle y \left(36 x y z - 9 x y - 3 x - 27 y z + 6 y + 3 z + 2\right)\\\displaystyle \frac{9 z \left(8 x y z - 8 x y - 4 x z + 4 x - 6 y z + 6 y + 3 z - 3\right)}{2}\end{array}\right)\)
This DOF is associated with face 4 of the reference cell.
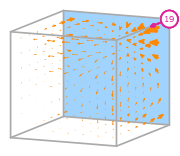
\(\displaystyle l_{19}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{4}}\boldsymbol{v}\cdot(s_{0} s_{1})\hat{\boldsymbol{n}}_{4}\)
where \(f_{4}\) is the 4th face;
\(\hat{\boldsymbol{n}}_{4}\) is the normal to facet 4;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{4}\).
\(\displaystyle \boldsymbol{\phi}_{19} = \left(\begin{array}{c}\displaystyle \frac{9 x \left(- 8 x y z + 2 x y + 4 x z - x + 8 y z - 2 y - 4 z + 1\right)}{2}\\\displaystyle y \left(- 36 x y z + 9 x y + 3 x + 9 y z - 3 y + 3 z - 1\right)\\\displaystyle \frac{9 z \left(- 8 x y z + 8 x y + 4 x z - 4 x + 2 y z - 2 y - z + 1\right)}{2}\end{array}\right)\)
This DOF is associated with face 4 of the reference cell.
where \(f_{4}\) is the 4th face;
\(\hat{\boldsymbol{n}}_{4}\) is the normal to facet 4;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{4}\).
\(\displaystyle \boldsymbol{\phi}_{19} = \left(\begin{array}{c}\displaystyle \frac{9 x \left(- 8 x y z + 2 x y + 4 x z - x + 8 y z - 2 y - 4 z + 1\right)}{2}\\\displaystyle y \left(- 36 x y z + 9 x y + 3 x + 9 y z - 3 y + 3 z - 1\right)\\\displaystyle \frac{9 z \left(- 8 x y z + 8 x y + 4 x z - 4 x + 2 y z - 2 y - z + 1\right)}{2}\end{array}\right)\)
This DOF is associated with face 4 of the reference cell.
\(\displaystyle l_{20}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{5}}\boldsymbol{v}\cdot(s_{0} s_{1} - s_{0} - s_{1} + 1)\hat{\boldsymbol{n}}_{5}\)
where \(f_{5}\) is the 5th face;
\(\hat{\boldsymbol{n}}_{5}\) is the normal to facet 5;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{5}\).
\(\displaystyle \boldsymbol{\phi}_{20} = \left(\begin{array}{c}\displaystyle \frac{9 x \left(8 x y z - 4 x y - 6 x z + 3 x - 8 y z + 4 y + 6 z - 3\right)}{2}\\\displaystyle \frac{9 y \left(8 x y z - 4 x y - 8 x z + 4 x - 6 y z + 3 y + 6 z - 3\right)}{2}\\\displaystyle z \left(36 x y z - 27 x z + 3 x - 27 y z + 3 y + 21 z - 5\right)\end{array}\right)\)
This DOF is associated with face 5 of the reference cell.
where \(f_{5}\) is the 5th face;
\(\hat{\boldsymbol{n}}_{5}\) is the normal to facet 5;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{5}\).
\(\displaystyle \boldsymbol{\phi}_{20} = \left(\begin{array}{c}\displaystyle \frac{9 x \left(8 x y z - 4 x y - 6 x z + 3 x - 8 y z + 4 y + 6 z - 3\right)}{2}\\\displaystyle \frac{9 y \left(8 x y z - 4 x y - 8 x z + 4 x - 6 y z + 3 y + 6 z - 3\right)}{2}\\\displaystyle z \left(36 x y z - 27 x z + 3 x - 27 y z + 3 y + 21 z - 5\right)\end{array}\right)\)
This DOF is associated with face 5 of the reference cell.
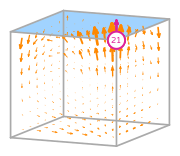
\(\displaystyle l_{21}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{5}}\boldsymbol{v}\cdot(s_{0} \left(1 - s_{1}\right))\hat{\boldsymbol{n}}_{5}\)
where \(f_{5}\) is the 5th face;
\(\hat{\boldsymbol{n}}_{5}\) is the normal to facet 5;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{5}\).
\(\displaystyle \boldsymbol{\phi}_{21} = \left(\begin{array}{c}\displaystyle \frac{9 x \left(- 8 x y z + 4 x y + 6 x z - 3 x + 8 y z - 4 y - 6 z + 3\right)}{2}\\\displaystyle \frac{9 y \left(- 8 x y z + 4 x y + 8 x z - 4 x + 2 y z - y - 2 z + 1\right)}{2}\\\displaystyle z \left(- 36 x y z + 27 x z - 3 x + 9 y z + 3 y - 6 z - 2\right)\end{array}\right)\)
This DOF is associated with face 5 of the reference cell.
where \(f_{5}\) is the 5th face;
\(\hat{\boldsymbol{n}}_{5}\) is the normal to facet 5;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{5}\).
\(\displaystyle \boldsymbol{\phi}_{21} = \left(\begin{array}{c}\displaystyle \frac{9 x \left(- 8 x y z + 4 x y + 6 x z - 3 x + 8 y z - 4 y - 6 z + 3\right)}{2}\\\displaystyle \frac{9 y \left(- 8 x y z + 4 x y + 8 x z - 4 x + 2 y z - y - 2 z + 1\right)}{2}\\\displaystyle z \left(- 36 x y z + 27 x z - 3 x + 9 y z + 3 y - 6 z - 2\right)\end{array}\right)\)
This DOF is associated with face 5 of the reference cell.
\(\displaystyle l_{22}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{5}}\boldsymbol{v}\cdot(s_{1} \left(1 - s_{0}\right))\hat{\boldsymbol{n}}_{5}\)
where \(f_{5}\) is the 5th face;
\(\hat{\boldsymbol{n}}_{5}\) is the normal to facet 5;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{5}\).
\(\displaystyle \boldsymbol{\phi}_{22} = \left(\begin{array}{c}\displaystyle \frac{9 x \left(- 8 x y z + 4 x y + 2 x z - x + 8 y z - 4 y - 2 z + 1\right)}{2}\\\displaystyle \frac{9 y \left(- 8 x y z + 4 x y + 8 x z - 4 x + 6 y z - 3 y - 6 z + 3\right)}{2}\\\displaystyle z \left(- 36 x y z + 9 x z + 3 x + 27 y z - 3 y - 6 z - 2\right)\end{array}\right)\)
This DOF is associated with face 5 of the reference cell.
where \(f_{5}\) is the 5th face;
\(\hat{\boldsymbol{n}}_{5}\) is the normal to facet 5;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{5}\).
\(\displaystyle \boldsymbol{\phi}_{22} = \left(\begin{array}{c}\displaystyle \frac{9 x \left(- 8 x y z + 4 x y + 2 x z - x + 8 y z - 4 y - 2 z + 1\right)}{2}\\\displaystyle \frac{9 y \left(- 8 x y z + 4 x y + 8 x z - 4 x + 6 y z - 3 y - 6 z + 3\right)}{2}\\\displaystyle z \left(- 36 x y z + 9 x z + 3 x + 27 y z - 3 y - 6 z - 2\right)\end{array}\right)\)
This DOF is associated with face 5 of the reference cell.
\(\displaystyle l_{23}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{5}}\boldsymbol{v}\cdot(s_{0} s_{1})\hat{\boldsymbol{n}}_{5}\)
where \(f_{5}\) is the 5th face;
\(\hat{\boldsymbol{n}}_{5}\) is the normal to facet 5;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{5}\).
\(\displaystyle \boldsymbol{\phi}_{23} = \left(\begin{array}{c}\displaystyle \frac{9 x \left(8 x y z - 4 x y - 2 x z + x - 8 y z + 4 y + 2 z - 1\right)}{2}\\\displaystyle \frac{9 y \left(8 x y z - 4 x y - 8 x z + 4 x - 2 y z + y + 2 z - 1\right)}{2}\\\displaystyle z \left(36 x y z - 9 x z - 3 x - 9 y z - 3 y + 3 z + 1\right)\end{array}\right)\)
This DOF is associated with face 5 of the reference cell.
where \(f_{5}\) is the 5th face;
\(\hat{\boldsymbol{n}}_{5}\) is the normal to facet 5;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{5}\).
\(\displaystyle \boldsymbol{\phi}_{23} = \left(\begin{array}{c}\displaystyle \frac{9 x \left(8 x y z - 4 x y - 2 x z + x - 8 y z + 4 y + 2 z - 1\right)}{2}\\\displaystyle \frac{9 y \left(8 x y z - 4 x y - 8 x z + 4 x - 2 y z + y + 2 z - 1\right)}{2}\\\displaystyle z \left(36 x y z - 9 x z - 3 x - 9 y z - 3 y + 3 z + 1\right)\end{array}\right)\)
This DOF is associated with face 5 of the reference cell.
\(\displaystyle l_{24}:\mathbf{v}\mapsto\displaystyle\int_{R}\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 1\end{array}\right)\cdot\mathbf{v}\)
where \(R\) is the reference element.
\(\displaystyle \boldsymbol{\phi}_{24} = \left(\begin{array}{c}\displaystyle 9 x \left(- 8 x y z + 4 x y + 6 x z - 3 x + 8 y z - 4 y - 6 z + 3\right)\\\displaystyle 9 y \left(- 8 x y z + 4 x y + 8 x z - 4 x + 6 y z - 3 y - 6 z + 3\right)\\\displaystyle 6 z \left(- 12 x y z + 12 x y + 9 x z - 9 x + 9 y z - 9 y - 7 z + 7\right)\end{array}\right)\)
This DOF is associated with volume 0 of the reference cell.
where \(R\) is the reference element.
\(\displaystyle \boldsymbol{\phi}_{24} = \left(\begin{array}{c}\displaystyle 9 x \left(- 8 x y z + 4 x y + 6 x z - 3 x + 8 y z - 4 y - 6 z + 3\right)\\\displaystyle 9 y \left(- 8 x y z + 4 x y + 8 x z - 4 x + 6 y z - 3 y - 6 z + 3\right)\\\displaystyle 6 z \left(- 12 x y z + 12 x y + 9 x z - 9 x + 9 y z - 9 y - 7 z + 7\right)\end{array}\right)\)
This DOF is associated with volume 0 of the reference cell.
\(\displaystyle l_{25}:\mathbf{v}\mapsto\displaystyle\int_{R}\left(\begin{array}{c}\displaystyle 0\\\displaystyle 1\\\displaystyle 0\end{array}\right)\cdot\mathbf{v}\)
where \(R\) is the reference element.
\(\displaystyle \boldsymbol{\phi}_{25} = \left(\begin{array}{c}\displaystyle 9 x \left(- 8 x y z + 6 x y + 4 x z - 3 x + 8 y z - 6 y - 4 z + 3\right)\\\displaystyle 6 y \left(- 12 x y z + 9 x y + 12 x z - 9 x + 9 y z - 7 y - 9 z + 7\right)\\\displaystyle 9 z \left(- 8 x y z + 8 x y + 4 x z - 4 x + 6 y z - 6 y - 3 z + 3\right)\end{array}\right)\)
This DOF is associated with volume 0 of the reference cell.
where \(R\) is the reference element.
\(\displaystyle \boldsymbol{\phi}_{25} = \left(\begin{array}{c}\displaystyle 9 x \left(- 8 x y z + 6 x y + 4 x z - 3 x + 8 y z - 6 y - 4 z + 3\right)\\\displaystyle 6 y \left(- 12 x y z + 9 x y + 12 x z - 9 x + 9 y z - 7 y - 9 z + 7\right)\\\displaystyle 9 z \left(- 8 x y z + 8 x y + 4 x z - 4 x + 6 y z - 6 y - 3 z + 3\right)\end{array}\right)\)
This DOF is associated with volume 0 of the reference cell.
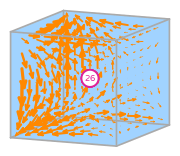
\(\displaystyle l_{26}:\mathbf{v}\mapsto\displaystyle\int_{R}\left(\begin{array}{c}\displaystyle 0\\\displaystyle s_{2}\\\displaystyle s_{1}\end{array}\right)\cdot\mathbf{v}\)
where \(R\) is the reference element;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{26} = \left(\begin{array}{c}\displaystyle 36 x \left(4 x y z - 2 x y - 2 x z + x - 4 y z + 2 y + 2 z - 1\right)\\\displaystyle 18 y \left(8 x y z - 4 x y - 8 x z + 4 x - 6 y z + 3 y + 6 z - 3\right)\\\displaystyle 18 z \left(8 x y z - 8 x y - 4 x z + 4 x - 6 y z + 6 y + 3 z - 3\right)\end{array}\right)\)
This DOF is associated with volume 0 of the reference cell.
where \(R\) is the reference element;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{26} = \left(\begin{array}{c}\displaystyle 36 x \left(4 x y z - 2 x y - 2 x z + x - 4 y z + 2 y + 2 z - 1\right)\\\displaystyle 18 y \left(8 x y z - 4 x y - 8 x z + 4 x - 6 y z + 3 y + 6 z - 3\right)\\\displaystyle 18 z \left(8 x y z - 8 x y - 4 x z + 4 x - 6 y z + 6 y + 3 z - 3\right)\end{array}\right)\)
This DOF is associated with volume 0 of the reference cell.
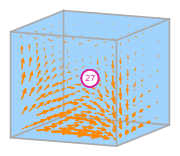
\(\displaystyle l_{27}:\mathbf{v}\mapsto\displaystyle\int_{R}\left(\begin{array}{c}\displaystyle 1\\\displaystyle 0\\\displaystyle 0\end{array}\right)\cdot\mathbf{v}\)
where \(R\) is the reference element.
\(\displaystyle \boldsymbol{\phi}_{27} = \left(\begin{array}{c}\displaystyle 6 x \left(- 12 x y z + 9 x y + 9 x z - 7 x + 12 y z - 9 y - 9 z + 7\right)\\\displaystyle 9 y \left(- 8 x y z + 6 x y + 8 x z - 6 x + 4 y z - 3 y - 4 z + 3\right)\\\displaystyle 9 z \left(- 8 x y z + 8 x y + 6 x z - 6 x + 4 y z - 4 y - 3 z + 3\right)\end{array}\right)\)
This DOF is associated with volume 0 of the reference cell.
where \(R\) is the reference element.
\(\displaystyle \boldsymbol{\phi}_{27} = \left(\begin{array}{c}\displaystyle 6 x \left(- 12 x y z + 9 x y + 9 x z - 7 x + 12 y z - 9 y - 9 z + 7\right)\\\displaystyle 9 y \left(- 8 x y z + 6 x y + 8 x z - 6 x + 4 y z - 3 y - 4 z + 3\right)\\\displaystyle 9 z \left(- 8 x y z + 8 x y + 6 x z - 6 x + 4 y z - 4 y - 3 z + 3\right)\end{array}\right)\)
This DOF is associated with volume 0 of the reference cell.
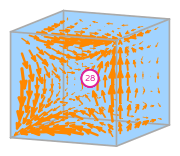
\(\displaystyle l_{28}:\mathbf{v}\mapsto\displaystyle\int_{R}\left(\begin{array}{c}\displaystyle s_{2}\\\displaystyle 0\\\displaystyle s_{0}\end{array}\right)\cdot\mathbf{v}\)
where \(R\) is the reference element;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{28} = \left(\begin{array}{c}\displaystyle 18 x \left(8 x y z - 4 x y - 6 x z + 3 x - 8 y z + 4 y + 6 z - 3\right)\\\displaystyle 36 y \left(4 x y z - 2 x y - 4 x z + 2 x - 2 y z + y + 2 z - 1\right)\\\displaystyle 18 z \left(8 x y z - 8 x y - 6 x z + 6 x - 4 y z + 4 y + 3 z - 3\right)\end{array}\right)\)
This DOF is associated with volume 0 of the reference cell.
where \(R\) is the reference element;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{28} = \left(\begin{array}{c}\displaystyle 18 x \left(8 x y z - 4 x y - 6 x z + 3 x - 8 y z + 4 y + 6 z - 3\right)\\\displaystyle 36 y \left(4 x y z - 2 x y - 4 x z + 2 x - 2 y z + y + 2 z - 1\right)\\\displaystyle 18 z \left(8 x y z - 8 x y - 6 x z + 6 x - 4 y z + 4 y + 3 z - 3\right)\end{array}\right)\)
This DOF is associated with volume 0 of the reference cell.
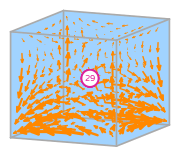
\(\displaystyle l_{29}:\mathbf{v}\mapsto\displaystyle\int_{R}\left(\begin{array}{c}\displaystyle s_{1}\\\displaystyle s_{0}\\\displaystyle 0\end{array}\right)\cdot\mathbf{v}\)
where \(R\) is the reference element;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{29} = \left(\begin{array}{c}\displaystyle 18 x \left(8 x y z - 6 x y - 4 x z + 3 x - 8 y z + 6 y + 4 z - 3\right)\\\displaystyle 18 y \left(8 x y z - 6 x y - 8 x z + 6 x - 4 y z + 3 y + 4 z - 3\right)\\\displaystyle 36 z \left(4 x y z - 4 x y - 2 x z + 2 x - 2 y z + 2 y + z - 1\right)\end{array}\right)\)
This DOF is associated with volume 0 of the reference cell.
where \(R\) is the reference element;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{29} = \left(\begin{array}{c}\displaystyle 18 x \left(8 x y z - 6 x y - 4 x z + 3 x - 8 y z + 6 y + 4 z - 3\right)\\\displaystyle 18 y \left(8 x y z - 6 x y - 8 x z + 6 x - 4 y z + 3 y + 4 z - 3\right)\\\displaystyle 36 z \left(4 x y z - 4 x y - 2 x z + 2 x - 2 y z + 2 y + z - 1\right)\end{array}\right)\)
This DOF is associated with volume 0 of the reference cell.
\(\displaystyle l_{30}:\mathbf{v}\mapsto\displaystyle\int_{R}\left(\begin{array}{c}\displaystyle s_{1} s_{2}\\\displaystyle s_{0} s_{2}\\\displaystyle s_{0} s_{1}\end{array}\right)\cdot\mathbf{v}\)
where \(R\) is the reference element;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{30} = \left(\begin{array}{c}\displaystyle 72 x \left(- 4 x y z + 2 x y + 2 x z - x + 4 y z - 2 y - 2 z + 1\right)\\\displaystyle 72 y \left(- 4 x y z + 2 x y + 4 x z - 2 x + 2 y z - y - 2 z + 1\right)\\\displaystyle 72 z \left(- 4 x y z + 4 x y + 2 x z - 2 x + 2 y z - 2 y - z + 1\right)\end{array}\right)\)
This DOF is associated with volume 0 of the reference cell.
where \(R\) is the reference element;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(R\).
\(\displaystyle \boldsymbol{\phi}_{30} = \left(\begin{array}{c}\displaystyle 72 x \left(- 4 x y z + 2 x y + 2 x z - x + 4 y z - 2 y - 2 z + 1\right)\\\displaystyle 72 y \left(- 4 x y z + 2 x y + 4 x z - 2 x + 2 y z - y - 2 z + 1\right)\\\displaystyle 72 z \left(- 4 x y z + 4 x y + 2 x z - 2 x + 2 y z - 2 y - z + 1\right)\end{array}\right)\)
This DOF is associated with volume 0 of the reference cell.