Degree 2 Guzmán–Neilan (second kind) on a tetrahedron

◀ Back to Guzmán–Neilan (second kind) definition page

In this example:

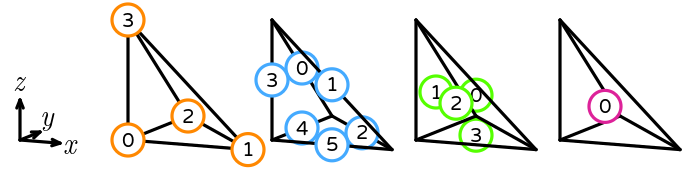
\(R\) is the reference tetrahedron. The following numbering of the subentities of the reference is used:

\(\mathcal{V}\) is spanned by: \(\begin{cases} \left(\begin{array}{c}\displaystyle 2 x^{2} + 4 x y + 8 x z - 3 x + 2 y^{2} + 8 y z - 3 y + 8 z^{2} - 6 z + 1\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 2 x^{2} + 8 x y + 4 x z - 3 x + 8 y^{2} + 8 y z - 6 y + 2 z^{2} - 3 z + 1\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 8 x^{2} + 8 x y + 8 x z - 6 x + 2 y^{2} + 4 y z - 3 y + 2 z^{2} - 3 z + 1\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 2 x^{2} + 4 x y + 8 x z - 3 x + 2 y^{2} + 8 y z - 3 y + 8 z^{2} - 6 z + 1\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 2 x^{2} + 8 x y + 4 x z - 3 x + 8 y^{2} + 8 y z - 6 y + 2 z^{2} - 3 z + 1\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 8 x^{2} + 8 x y + 8 x z - 6 x + 2 y^{2} + 4 y z - 3 y + 2 z^{2} - 3 z + 1\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 2 x^{2} + 4 x y + 8 x z - 3 x + 2 y^{2} + 8 y z - 3 y + 8 z^{2} - 6 z + 1\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 2 x^{2} + 8 x y + 4 x z - 3 x + 8 y^{2} + 8 y z - 6 y + 2 z^{2} - 3 z + 1\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 8 x^{2} + 8 x y + 8 x z - 6 x + 2 y^{2} + 4 y z - 3 y + 2 z^{2} - 3 z + 1\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 2 x^{2} - 4 x z - x + 2 z^{2} + z\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 2 x^{2} - 4 x y - x + 2 y^{2} + y\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 8 x^{2} + 8 x y + 8 x z - 10 x + 2 y^{2} + 4 y z - 5 y + 2 z^{2} - 5 z + 3\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 2 x^{2} - 4 x z - x + 2 z^{2} + z\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 2 x^{2} - 4 x y - x + 2 y^{2} + y\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 8 x^{2} + 8 x y + 8 x z - 10 x + 2 y^{2} + 4 y z - 5 y + 2 z^{2} - 5 z + 3\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 2 x^{2} - 4 x z - x + 2 z^{2} + z\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 2 x^{2} - 4 x y - x + 2 y^{2} + y\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 8 x^{2} + 8 x y + 8 x z - 10 x + 2 y^{2} + 4 y z - 5 y + 2 z^{2} - 5 z + 3\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 2 y^{2} - 4 y z - y + 2 z^{2} + z\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 2 x^{2} - 4 x y + x + 2 y^{2} - y\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 2 x^{2} + 8 x y + 4 x z - 5 x + 8 y^{2} + 8 y z - 10 y + 2 z^{2} - 5 z + 3\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 2 y^{2} - 4 y z - y + 2 z^{2} + z\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 2 x^{2} - 4 x y + x + 2 y^{2} - y\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 2 x^{2} + 8 x y + 4 x z - 5 x + 8 y^{2} + 8 y z - 10 y + 2 z^{2} - 5 z + 3\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 2 y^{2} - 4 y z - y + 2 z^{2} + z\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 2 x^{2} - 4 x y + x + 2 y^{2} - y\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 2 x^{2} + 8 x y + 4 x z - 5 x + 8 y^{2} + 8 y z - 10 y + 2 z^{2} - 5 z + 3\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 2 y^{2} - 4 y z + y + 2 z^{2} - z\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 2 x^{2} - 4 x z + x + 2 z^{2} - z\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 2 x^{2} + 4 x y + 8 x z - 5 x + 2 y^{2} + 8 y z - 5 y + 8 z^{2} - 10 z + 3\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 2 y^{2} - 4 y z + y + 2 z^{2} - z\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 2 x^{2} - 4 x z + x + 2 z^{2} - z\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 2 x^{2} + 4 x y + 8 x z - 5 x + 2 y^{2} + 8 y z - 5 y + 8 z^{2} - 10 z + 3\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 2 y^{2} - 4 y z + y + 2 z^{2} - z\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 2 x^{2} - 4 x z + x + 2 z^{2} - z\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 2 x^{2} + 4 x y + 8 x z - 5 x + 2 y^{2} + 8 y z - 5 y + 8 z^{2} - 10 z + 3\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 4 z \left(8 z - 1\right)\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 4 y \left(8 y - 1\right)\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 4 x \left(8 x - 1\right)\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 32 x^{2} + 64 x y + 64 x z - 60 x + 32 y^{2} + 64 y z - 60 y + 32 z^{2} - 60 z + 28\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 4 z \left(8 z - 1\right)\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 4 y \left(8 y - 1\right)\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 4 x \left(8 x - 1\right)\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 32 x^{2} + 64 x y + 64 x z - 60 x + 32 y^{2} + 64 y z - 60 y + 32 z^{2} - 60 z + 28\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 4 z \left(8 z - 1\right)\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 4 y \left(8 y - 1\right)\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 4 x \left(8 x - 1\right)\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 32 x^{2} + 64 x y + 64 x z - 60 x + 32 y^{2} + 64 y z - 60 y + 32 z^{2} - 60 z + 28\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 4 x y - 4 x z - 4 y z + 4 z^{2}\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 8 x^{2} + 20 x y + 12 x z - 12 x + 8 y^{2} + 12 y z - 12 y + 4 z^{2} - 8 z + 4\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 4 x y - 4 x z - 4 y z + 4 z^{2}\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 8 x^{2} + 20 x y + 12 x z - 12 x + 8 y^{2} + 12 y z - 12 y + 4 z^{2} - 8 z + 4\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 4 x y - 4 x z - 4 y z + 4 z^{2}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 8 x^{2} + 20 x y + 12 x z - 12 x + 8 y^{2} + 12 y z - 12 y + 4 z^{2} - 8 z + 4\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle - 4 x^{2} - 4 x y - 4 x z + 4 x + 4 y z + 8 z^{2} - 4 z\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle - 4 x^{2} - 4 x y - 4 x z + 4 x + 8 y^{2} + 4 y z - 4 y\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle - 4 x^{2} - 4 x y - 4 x z + 4 x + 4 y z + 8 z^{2} - 4 z\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle - 4 x^{2} - 4 x y - 4 x z + 4 x + 8 y^{2} + 4 y z - 4 y\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle - 4 x^{2} - 4 x y - 4 x z + 4 x + 4 y z + 8 z^{2} - 4 z\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle - 4 x^{2} - 4 x y - 4 x z + 4 x + 8 y^{2} + 4 y z - 4 y\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle - 4 x y + 4 x z - 4 y^{2} - 4 y z + 4 y + 8 z^{2} - 4 z\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 8 x^{2} - 4 x y + 4 x z - 4 x - 4 y^{2} - 4 y z + 4 y\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle - 4 x y + 4 x z - 4 y^{2} - 4 y z + 4 y + 8 z^{2} - 4 z\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 8 x^{2} - 4 x y + 4 x z - 4 x - 4 y^{2} - 4 y z + 4 y\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle - 4 x y + 4 x z - 4 y^{2} - 4 y z + 4 y + 8 z^{2} - 4 z\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 8 x^{2} - 4 x y + 4 x z - 4 x - 4 y^{2} - 4 y z + 4 y\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle - 4 x y + 4 x z + 4 y^{2} - 4 y z\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 8 x^{2} + 12 x y + 20 x z - 12 x + 4 y^{2} + 12 y z - 8 y + 8 z^{2} - 12 z + 4\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle - 4 x y + 4 x z + 4 y^{2} - 4 y z\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 8 x^{2} + 12 x y + 20 x z - 12 x + 4 y^{2} + 12 y z - 8 y + 8 z^{2} - 12 z + 4\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle - 4 x y + 4 x z + 4 y^{2} - 4 y z\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 8 x^{2} + 12 x y + 20 x z - 12 x + 4 y^{2} + 12 y z - 8 y + 8 z^{2} - 12 z + 4\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 4 x y - 4 x z + 8 y^{2} - 4 y z - 4 y - 4 z^{2} + 4 z\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 8 x^{2} + 4 x y - 4 x z - 4 x - 4 y z - 4 z^{2} + 4 z\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 4 x y - 4 x z + 8 y^{2} - 4 y z - 4 y - 4 z^{2} + 4 z\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 8 x^{2} + 4 x y - 4 x z - 4 x - 4 y z - 4 z^{2} + 4 z\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 4 x y - 4 x z + 8 y^{2} - 4 y z - 4 y - 4 z^{2} + 4 z\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 8 x^{2} + 4 x y - 4 x z - 4 x - 4 y z - 4 z^{2} + 4 z\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 4 x^{2} - 4 x y - 4 x z + 4 y z\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 4 x^{2} + 12 x y + 12 x z - 8 x + 8 y^{2} + 20 y z - 12 y + 8 z^{2} - 12 z + 4\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 4 x^{2} - 4 x y - 4 x z + 4 y z\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 4 x^{2} + 12 x y + 12 x z - 8 x + 8 y^{2} + 20 y z - 12 y + 8 z^{2} - 12 z + 4\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 4 x^{2} - 4 x y - 4 x z + 4 y z\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 4 x^{2} + 12 x y + 12 x z - 8 x + 8 y^{2} + 20 y z - 12 y + 8 z^{2} - 12 z + 4\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 16 z \left(- x - y - 2 z + 1\right)\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 16 y \left(- x - 2 y - z + 1\right)\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 16 x \left(- 2 x - y - z + 1\right)\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 16 z \left(- x - y - 2 z + 1\right)\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 16 y \left(- x - 2 y - z + 1\right)\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 16 x \left(- 2 x - y - z + 1\right)\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 16 z \left(- x - y - 2 z + 1\right)\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 16 y \left(- x - 2 y - z + 1\right)\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 16 x \left(- 2 x - y - z + 1\right)\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 16 y \left(- y + z\right)\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 16 x \left(- x + z\right)\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle - 16 x^{2} - 32 x y - 48 x z + 32 x - 16 y^{2} - 48 y z + 32 y - 32 z^{2} + 48 z - 16\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 16 y \left(- y + z\right)\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 16 x \left(- x + z\right)\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle - 16 x^{2} - 32 x y - 48 x z + 32 x - 16 y^{2} - 48 y z + 32 y - 32 z^{2} + 48 z - 16\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 16 y \left(- y + z\right)\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 16 x \left(- x + z\right)\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle - 16 x^{2} - 32 x y - 48 x z + 32 x - 16 y^{2} - 48 y z + 32 y - 32 z^{2} + 48 z - 16\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 16 z \left(y - z\right)\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 16 x \left(- x + y\right)\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle - 16 x^{2} - 48 x y - 32 x z + 32 x - 32 y^{2} - 48 y z + 48 y - 16 z^{2} + 32 z - 16\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 16 z \left(y - z\right)\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 16 x \left(- x + y\right)\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle - 16 x^{2} - 48 x y - 32 x z + 32 x - 32 y^{2} - 48 y z + 48 y - 16 z^{2} + 32 z - 16\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 16 z \left(y - z\right)\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 16 x \left(- x + y\right)\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle - 16 x^{2} - 48 x y - 32 x z + 32 x - 32 y^{2} - 48 y z + 48 y - 16 z^{2} + 32 z - 16\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 16 z \left(x - z\right)\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 16 y \left(x - y\right)\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle - 32 x^{2} - 48 x y - 48 x z + 48 x - 16 y^{2} - 32 y z + 32 y - 16 z^{2} + 32 z - 16\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 16 z \left(x - z\right)\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 16 y \left(x - y\right)\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle - 32 x^{2} - 48 x y - 48 x z + 48 x - 16 y^{2} - 32 y z + 32 y - 16 z^{2} + 32 z - 16\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 16 z \left(x - z\right)\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 16 y \left(x - y\right)\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle - 32 x^{2} - 48 x y - 48 x z + 48 x - 16 y^{2} - 32 y z + 32 y - 16 z^{2} + 32 z - 16\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} z \left(60 x^{2} + 180 x y + 132 x z - 96 x - 330 y z - 430 z^{2} + 192 z + 9\right)}{180}\\\displaystyle \tfrac{\sqrt{3} z \left(180 x y - 330 x z + 60 y^{2} + 132 y z - 96 y - 430 z^{2} + 192 z + 9\right)}{180}\\\displaystyle \tfrac{\sqrt{3} z \left(- 150 x z - 150 y z - 88 z^{2} + 96 z + 9\right)}{180}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} y \left(60 x^{2} + 132 x y + 180 x z - 96 x - 430 y^{2} - 330 y z + 192 y + 9\right)}{180}\\\displaystyle \tfrac{\sqrt{3} y \left(- 150 x y - 88 y^{2} - 150 y z + 96 y + 9\right)}{180}\\\displaystyle \tfrac{\sqrt{3} y \left(- 330 x y + 180 x z - 430 y^{2} + 132 y z + 192 y + 60 z^{2} - 96 z + 9\right)}{180}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} x \left(- 88 x^{2} - 150 x y - 150 x z + 96 x + 9\right)}{180}\\\displaystyle \tfrac{\sqrt{3} x \left(- 430 x^{2} + 132 x y - 330 x z + 192 x + 60 y^{2} + 180 y z - 96 y + 9\right)}{180}\\\displaystyle \tfrac{\sqrt{3} x \left(- 430 x^{2} - 330 x y + 132 x z + 192 x + 180 y z + 60 z^{2} - 96 z + 9\right)}{180}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{\sqrt{3} \left(112 x^{3} + 54 x^{2} y + 54 x^{2} z - 156 x^{2} - 168 x y^{2} - 216 x y z + 156 x y - 168 x z^{2} + 156 x z + 3 x - 110 y^{3} - 270 y^{2} z + 252 y^{2} - 270 y z^{2} + 444 y z - 183 y - 110 z^{3} + 252 z^{2} - 183 z + 41\right)}{180}\\\displaystyle \tfrac{\sqrt{3} \left(- 110 x^{3} - 168 x^{2} y - 270 x^{2} z + 252 x^{2} + 54 x y^{2} - 216 x y z + 156 x y - 270 x z^{2} + 444 x z - 183 x + 112 y^{3} + 54 y^{2} z - 156 y^{2} - 168 y z^{2} + 156 y z + 3 y - 110 z^{3} + 252 z^{2} - 183 z + 41\right)}{180}\\\displaystyle \tfrac{\sqrt{3} \left(- 110 x^{3} - 270 x^{2} y - 168 x^{2} z + 252 x^{2} - 270 x y^{2} - 216 x y z + 444 x y + 54 x z^{2} + 156 x z - 183 x - 110 y^{3} - 168 y^{2} z + 252 y^{2} + 54 y z^{2} + 156 y z - 183 y + 112 z^{3} - 156 z^{2} + 3 z + 41\right)}{180}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle \tfrac{z \left(- 90 x y + 216 x z + 12 x - 60 y^{2} + 105 y z + 60 y - 55 z^{2} - 54 z - 3\right)}{60}\\\displaystyle \tfrac{z \left(- 30 y^{2} - 219 y z + 42 y + 325 z^{2} - 69 z - 3\right)}{60}\\\displaystyle \tfrac{z \left(75 y z + z^{2} - 27 z - 3\right)}{60}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{y \left(216 x y - 90 x z + 12 x - 55 y^{2} + 105 y z - 54 y - 60 z^{2} + 60 z - 3\right)}{60}\\\displaystyle \tfrac{y \left(y^{2} + 75 y z - 27 y - 3\right)}{60}\\\displaystyle \tfrac{y \left(325 y^{2} - 219 y z - 69 y - 30 z^{2} + 42 z - 3\right)}{60}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{43 x^{3}}{30} + \tfrac{5 x^{2} y}{4} + \tfrac{5 x^{2} z}{4} - \tfrac{7 x^{2}}{10} - \tfrac{x}{20} - y^{2} z - y z^{2} + y z\\\displaystyle \tfrac{x \left(160 x^{2} - 129 x y + 165 x z - 69 x - 30 y^{2} - 90 y z + 42 y - 3\right)}{60}\\\displaystyle \tfrac{x \left(160 x^{2} + 165 x y - 129 x z - 69 x - 90 y z - 30 z^{2} + 42 z - 3\right)}{60}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{203 x^{3}}{30} + \tfrac{369 x^{2} y}{20} + \tfrac{369 x^{2} z}{20} - \tfrac{173 x^{2}}{10} + \tfrac{161 x y^{2}}{10} + \tfrac{151 x y z}{5} - \tfrac{151 x y}{5} + \tfrac{161 x z^{2}}{10} - \tfrac{151 x z}{5} + \tfrac{283 x}{20} + \tfrac{53 y^{3}}{12} + \tfrac{45 y^{2} z}{4} - \tfrac{62 y^{2}}{5} + \tfrac{45 y z^{2}}{4} - \tfrac{114 y z}{5} + \tfrac{58 y}{5} + \tfrac{53 z^{3}}{12} - \tfrac{62 z^{2}}{5} + \tfrac{58 z}{5} - \tfrac{217}{60}\\\displaystyle - \tfrac{8 x^{3}}{3} - \tfrac{203 x^{2} y}{20} - \tfrac{21 x^{2} z}{4} + \tfrac{137 x^{2}}{20} - \tfrac{59 x y^{2}}{5} - \tfrac{133 x y z}{10} + \tfrac{173 x y}{10} - \tfrac{5 x z^{2}}{2} + \tfrac{41 x z}{5} - \tfrac{113 x}{20} - \tfrac{259 y^{3}}{60} - \tfrac{151 y^{2} z}{20} + \tfrac{199 y^{2}}{20} - \tfrac{63 y z^{2}}{20} + \tfrac{103 y z}{10} - \tfrac{71 y}{10} + \tfrac{z^{3}}{12} + \tfrac{27 z^{2}}{20} - \tfrac{29 z}{10} + \tfrac{22}{15}\\\displaystyle - \tfrac{8 x^{3}}{3} - \tfrac{21 x^{2} y}{4} - \tfrac{203 x^{2} z}{20} + \tfrac{137 x^{2}}{20} - \tfrac{5 x y^{2}}{2} - \tfrac{133 x y z}{10} + \tfrac{41 x y}{5} - \tfrac{59 x z^{2}}{5} + \tfrac{173 x z}{10} - \tfrac{113 x}{20} + \tfrac{y^{3}}{12} - \tfrac{63 y^{2} z}{20} + \tfrac{27 y^{2}}{20} - \tfrac{151 y z^{2}}{20} + \tfrac{103 y z}{10} - \tfrac{29 y}{10} - \tfrac{259 z^{3}}{60} + \tfrac{199 z^{2}}{20} - \tfrac{71 z}{10} + \tfrac{22}{15}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle \tfrac{z \left(30 x^{2} + 219 x z - 42 x - 325 z^{2} + 69 z + 3\right)}{60}\\\displaystyle \tfrac{z \left(60 x^{2} + 90 x y - 105 x z - 60 x - 216 y z - 12 y + 55 z^{2} + 54 z + 3\right)}{60}\\\displaystyle \tfrac{z \left(- 75 x z - z^{2} + 27 z + 3\right)}{60}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{y \left(30 x^{2} + 129 x y + 90 x z - 42 x - 160 y^{2} - 165 y z + 69 y + 3\right)}{60}\\\displaystyle x^{2} z - \tfrac{5 x y^{2}}{4} + x z^{2} - x z - \tfrac{43 y^{3}}{30} - \tfrac{5 y^{2} z}{4} + \tfrac{7 y^{2}}{10} + \tfrac{y}{20}\\\displaystyle \tfrac{y \left(- 165 x y + 90 x z - 160 y^{2} + 129 y z + 69 y + 30 z^{2} - 42 z + 3\right)}{60}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{x \left(- x^{2} - 75 x z + 27 x + 3\right)}{60}\\\displaystyle \tfrac{x \left(55 x^{2} - 216 x y - 105 x z + 54 x + 90 y z - 12 y + 60 z^{2} - 60 z + 3\right)}{60}\\\displaystyle \tfrac{x \left(- 325 x^{2} + 219 x z + 69 x + 30 z^{2} - 42 z + 3\right)}{60}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{259 x^{3}}{60} + \tfrac{59 x^{2} y}{5} + \tfrac{151 x^{2} z}{20} - \tfrac{199 x^{2}}{20} + \tfrac{203 x y^{2}}{20} + \tfrac{133 x y z}{10} - \tfrac{173 x y}{10} + \tfrac{63 x z^{2}}{20} - \tfrac{103 x z}{10} + \tfrac{71 x}{10} + \tfrac{8 y^{3}}{3} + \tfrac{21 y^{2} z}{4} - \tfrac{137 y^{2}}{20} + \tfrac{5 y z^{2}}{2} - \tfrac{41 y z}{5} + \tfrac{113 y}{20} - \tfrac{z^{3}}{12} - \tfrac{27 z^{2}}{20} + \tfrac{29 z}{10} - \tfrac{22}{15}\\\displaystyle - \tfrac{53 x^{3}}{12} - \tfrac{161 x^{2} y}{10} - \tfrac{45 x^{2} z}{4} + \tfrac{62 x^{2}}{5} - \tfrac{369 x y^{2}}{20} - \tfrac{151 x y z}{5} + \tfrac{151 x y}{5} - \tfrac{45 x z^{2}}{4} + \tfrac{114 x z}{5} - \tfrac{58 x}{5} - \tfrac{203 y^{3}}{30} - \tfrac{369 y^{2} z}{20} + \tfrac{173 y^{2}}{10} - \tfrac{161 y z^{2}}{10} + \tfrac{151 y z}{5} - \tfrac{283 y}{20} - \tfrac{53 z^{3}}{12} + \tfrac{62 z^{2}}{5} - \tfrac{58 z}{5} + \tfrac{217}{60}\\\displaystyle - \tfrac{x^{3}}{12} + \tfrac{5 x^{2} y}{2} + \tfrac{63 x^{2} z}{20} - \tfrac{27 x^{2}}{20} + \tfrac{21 x y^{2}}{4} + \tfrac{133 x y z}{10} - \tfrac{41 x y}{5} + \tfrac{151 x z^{2}}{20} - \tfrac{103 x z}{10} + \tfrac{29 x}{10} + \tfrac{8 y^{3}}{3} + \tfrac{203 y^{2} z}{20} - \tfrac{137 y^{2}}{20} + \tfrac{59 y z^{2}}{5} - \tfrac{173 y z}{10} + \tfrac{113 y}{20} + \tfrac{259 z^{3}}{60} - \tfrac{199 z^{2}}{20} + \tfrac{71 z}{10} - \tfrac{22}{15}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\), \(\begin{cases} \left(\begin{array}{c}\displaystyle \tfrac{z \left(- 30 x^{2} - 90 x y - 129 x z + 42 x + 165 y z + 160 z^{2} - 69 z - 3\right)}{60}\\\displaystyle \tfrac{z \left(- 90 x y + 165 x z - 30 y^{2} - 129 y z + 42 y + 160 z^{2} - 69 z - 3\right)}{60}\\\displaystyle - x^{2} y - x y^{2} + x y + \tfrac{5 x z^{2}}{4} + \tfrac{5 y z^{2}}{4} + \tfrac{43 z^{3}}{30} - \tfrac{7 z^{2}}{10} - \tfrac{z}{20}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{y \left(- 30 x^{2} - 219 x y + 42 x + 325 y^{2} - 69 y - 3\right)}{60}\\\displaystyle \tfrac{y \left(75 x y + y^{2} - 27 y - 3\right)}{60}\\\displaystyle \tfrac{y \left(- 60 x^{2} + 105 x y - 90 x z + 60 x - 55 y^{2} + 216 y z - 54 y + 12 z - 3\right)}{60}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{x \left(x^{2} + 75 x y - 27 x - 3\right)}{60}\\\displaystyle \tfrac{x \left(325 x^{2} - 219 x y - 69 x - 30 y^{2} + 42 y - 3\right)}{60}\\\displaystyle \tfrac{x \left(- 55 x^{2} + 105 x y + 216 x z - 54 x - 60 y^{2} - 90 y z + 60 y + 12 z - 3\right)}{60}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle - \tfrac{259 x^{3}}{60} - \tfrac{151 x^{2} y}{20} - \tfrac{59 x^{2} z}{5} + \tfrac{199 x^{2}}{20} - \tfrac{63 x y^{2}}{20} - \tfrac{133 x y z}{10} + \tfrac{103 x y}{10} - \tfrac{203 x z^{2}}{20} + \tfrac{173 x z}{10} - \tfrac{71 x}{10} + \tfrac{y^{3}}{12} - \tfrac{5 y^{2} z}{2} + \tfrac{27 y^{2}}{20} - \tfrac{21 y z^{2}}{4} + \tfrac{41 y z}{5} - \tfrac{29 y}{10} - \tfrac{8 z^{3}}{3} + \tfrac{137 z^{2}}{20} - \tfrac{113 z}{20} + \tfrac{22}{15}\\\displaystyle \tfrac{x^{3}}{12} - \tfrac{63 x^{2} y}{20} - \tfrac{5 x^{2} z}{2} + \tfrac{27 x^{2}}{20} - \tfrac{151 x y^{2}}{20} - \tfrac{133 x y z}{10} + \tfrac{103 x y}{10} - \tfrac{21 x z^{2}}{4} + \tfrac{41 x z}{5} - \tfrac{29 x}{10} - \tfrac{259 y^{3}}{60} - \tfrac{59 y^{2} z}{5} + \tfrac{199 y^{2}}{20} - \tfrac{203 y z^{2}}{20} + \tfrac{173 y z}{10} - \tfrac{71 y}{10} - \tfrac{8 z^{3}}{3} + \tfrac{137 z^{2}}{20} - \tfrac{113 z}{20} + \tfrac{22}{15}\\\displaystyle \tfrac{53 x^{3}}{12} + \tfrac{45 x^{2} y}{4} + \tfrac{161 x^{2} z}{10} - \tfrac{62 x^{2}}{5} + \tfrac{45 x y^{2}}{4} + \tfrac{151 x y z}{5} - \tfrac{114 x y}{5} + \tfrac{369 x z^{2}}{20} - \tfrac{151 x z}{5} + \tfrac{58 x}{5} + \tfrac{53 y^{3}}{12} + \tfrac{161 y^{2} z}{10} - \tfrac{62 y^{2}}{5} + \tfrac{369 y z^{2}}{20} - \tfrac{151 y z}{5} + \tfrac{58 y}{5} + \tfrac{203 z^{3}}{30} - \tfrac{173 z^{2}}{10} + \tfrac{283 z}{20} - \tfrac{217}{60}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)
\(\mathcal{L}=\{l_0,...,l_{48}\}\)
Functionals and basis functions:

\(\displaystyle l_{0}:\boldsymbol{v}\mapsto\boldsymbol{v}(0,0,0)\cdot\left(\begin{array}{c}1\\0\\0\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{0} = \begin{cases} \left(\begin{array}{c}\displaystyle 2 x^{2} + 4 x y + 8 x z - 3 x + 2 y^{2} + 8 y z - 3 y + 8 z^{2} - 6 z + 1\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 2 x^{2} + 8 x y + 4 x z - 3 x + 8 y^{2} + 8 y z - 6 y + 2 z^{2} - 3 z + 1\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 8 x^{2} + 8 x y + 8 x z - 6 x + 2 y^{2} + 4 y z - 3 y + 2 z^{2} - 3 z + 1\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with vertex 0 of the reference element.

\(\displaystyle l_{1}:\boldsymbol{v}\mapsto\boldsymbol{v}(0,0,0)\cdot\left(\begin{array}{c}0\\1\\0\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{1} = \begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 2 x^{2} + 4 x y + 8 x z - 3 x + 2 y^{2} + 8 y z - 3 y + 8 z^{2} - 6 z + 1\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 2 x^{2} + 8 x y + 4 x z - 3 x + 8 y^{2} + 8 y z - 6 y + 2 z^{2} - 3 z + 1\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 8 x^{2} + 8 x y + 8 x z - 6 x + 2 y^{2} + 4 y z - 3 y + 2 z^{2} - 3 z + 1\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with vertex 0 of the reference element.

\(\displaystyle l_{2}:\boldsymbol{v}\mapsto\boldsymbol{v}(0,0,0)\cdot\left(\begin{array}{c}0\\0\\1\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{2} = \begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 2 x^{2} + 4 x y + 8 x z - 3 x + 2 y^{2} + 8 y z - 3 y + 8 z^{2} - 6 z + 1\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 2 x^{2} + 8 x y + 4 x z - 3 x + 8 y^{2} + 8 y z - 6 y + 2 z^{2} - 3 z + 1\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 8 x^{2} + 8 x y + 8 x z - 6 x + 2 y^{2} + 4 y z - 3 y + 2 z^{2} - 3 z + 1\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with vertex 0 of the reference element.

\(\displaystyle l_{3}:\boldsymbol{v}\mapsto\boldsymbol{v}(1,0,0)\cdot\left(\begin{array}{c}1\\0\\0\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{3} = \begin{cases} \left(\begin{array}{c}\displaystyle 2 x^{2} - 4 x z - x + 2 z^{2} + z\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 2 x^{2} - 4 x y - x + 2 y^{2} + y\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 8 x^{2} + 8 x y + 8 x z - 10 x + 2 y^{2} + 4 y z - 5 y + 2 z^{2} - 5 z + 3\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with vertex 1 of the reference element.

\(\displaystyle l_{4}:\boldsymbol{v}\mapsto\boldsymbol{v}(1,0,0)\cdot\left(\begin{array}{c}0\\1\\0\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{4} = \begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 2 x^{2} - 4 x z - x + 2 z^{2} + z\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 2 x^{2} - 4 x y - x + 2 y^{2} + y\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 8 x^{2} + 8 x y + 8 x z - 10 x + 2 y^{2} + 4 y z - 5 y + 2 z^{2} - 5 z + 3\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with vertex 1 of the reference element.

\(\displaystyle l_{5}:\boldsymbol{v}\mapsto\boldsymbol{v}(1,0,0)\cdot\left(\begin{array}{c}0\\0\\1\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{5} = \begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 2 x^{2} - 4 x z - x + 2 z^{2} + z\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 2 x^{2} - 4 x y - x + 2 y^{2} + y\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 8 x^{2} + 8 x y + 8 x z - 10 x + 2 y^{2} + 4 y z - 5 y + 2 z^{2} - 5 z + 3\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with vertex 1 of the reference element.

\(\displaystyle l_{6}:\boldsymbol{v}\mapsto\boldsymbol{v}(0,1,0)\cdot\left(\begin{array}{c}1\\0\\0\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{6} = \begin{cases} \left(\begin{array}{c}\displaystyle 2 y^{2} - 4 y z - y + 2 z^{2} + z\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 2 x^{2} - 4 x y + x + 2 y^{2} - y\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 2 x^{2} + 8 x y + 4 x z - 5 x + 8 y^{2} + 8 y z - 10 y + 2 z^{2} - 5 z + 3\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with vertex 2 of the reference element.

\(\displaystyle l_{7}:\boldsymbol{v}\mapsto\boldsymbol{v}(0,1,0)\cdot\left(\begin{array}{c}0\\1\\0\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{7} = \begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 2 y^{2} - 4 y z - y + 2 z^{2} + z\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 2 x^{2} - 4 x y + x + 2 y^{2} - y\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 2 x^{2} + 8 x y + 4 x z - 5 x + 8 y^{2} + 8 y z - 10 y + 2 z^{2} - 5 z + 3\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with vertex 2 of the reference element.

\(\displaystyle l_{8}:\boldsymbol{v}\mapsto\boldsymbol{v}(0,1,0)\cdot\left(\begin{array}{c}0\\0\\1\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{8} = \begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 2 y^{2} - 4 y z - y + 2 z^{2} + z\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 2 x^{2} - 4 x y + x + 2 y^{2} - y\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 2 x^{2} + 8 x y + 4 x z - 5 x + 8 y^{2} + 8 y z - 10 y + 2 z^{2} - 5 z + 3\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with vertex 2 of the reference element.

\(\displaystyle l_{9}:\boldsymbol{v}\mapsto\boldsymbol{v}(0,0,1)\cdot\left(\begin{array}{c}1\\0\\0\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{9} = \begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 2 y^{2} - 4 y z + y + 2 z^{2} - z\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 2 x^{2} - 4 x z + x + 2 z^{2} - z\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 2 x^{2} + 4 x y + 8 x z - 5 x + 2 y^{2} + 8 y z - 5 y + 8 z^{2} - 10 z + 3\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with vertex 3 of the reference element.

\(\displaystyle l_{10}:\boldsymbol{v}\mapsto\boldsymbol{v}(0,0,1)\cdot\left(\begin{array}{c}0\\1\\0\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{10} = \begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 2 y^{2} - 4 y z + y + 2 z^{2} - z\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 2 x^{2} - 4 x z + x + 2 z^{2} - z\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 2 x^{2} + 4 x y + 8 x z - 5 x + 2 y^{2} + 8 y z - 5 y + 8 z^{2} - 10 z + 3\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with vertex 3 of the reference element.

\(\displaystyle l_{11}:\boldsymbol{v}\mapsto\boldsymbol{v}(0,0,1)\cdot\left(\begin{array}{c}0\\0\\1\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{11} = \begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 2 y^{2} - 4 y z + y + 2 z^{2} - z\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 2 x^{2} - 4 x z + x + 2 z^{2} - z\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 2 x^{2} + 4 x y + 8 x z - 5 x + 2 y^{2} + 8 y z - 5 y + 8 z^{2} - 10 z + 3\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with vertex 3 of the reference element.

\(\displaystyle l_{12}:\boldsymbol{v}\mapsto\boldsymbol{v}(0,\tfrac{1}{2},\tfrac{1}{2})\cdot\left(\begin{array}{c}1\\0\\0\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{12} = \begin{cases} \left(\begin{array}{c}\displaystyle \tfrac{5 z \left(- 96 x^{2} + 144 x y - 1248 x z + 210 x + 288 y^{2} + 24 y z - 237 y + 952 z^{2} + 3 z + 2\right)}{72}\\\displaystyle \tfrac{5 z \left(- 288 x y + 528 x z - 30 x + 48 y^{2} + 840 y z - 105 y - 872 z^{2} + 39 z + 8\right)}{72}\\\displaystyle \tfrac{5 z \left(240 x z - 30 x - 120 y z + 15 y + 136 z^{2} - 81 z + 8\right)}{72}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{5 y \left(- 96 x^{2} - 1248 x y + 144 x z + 210 x + 952 y^{2} + 24 y z + 3 y + 288 z^{2} - 237 z + 2\right)}{72}\\\displaystyle \tfrac{5 y \left(240 x y - 30 x + 136 y^{2} - 120 y z - 81 y + 15 z + 8\right)}{72}\\\displaystyle \tfrac{5 y \left(528 x y - 288 x z - 30 x - 872 y^{2} + 840 y z + 39 y + 48 z^{2} - 105 z + 8\right)}{72}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle - \tfrac{170 x^{3}}{9} - \tfrac{25 x^{2} y}{3} - \tfrac{25 x^{2} z}{3} + \tfrac{61 x^{2}}{4} - \tfrac{11 x y}{24} - \tfrac{11 x z}{24} + \tfrac{5 x}{36} + 20 y^{2} z + 20 y z^{2} - 16 y z\\\displaystyle \tfrac{5 x \left(- 80 x^{2} + 408 x y - 264 x z - 6 x + 48 y^{2} + 144 y z - 105 y + 15 z + 8\right)}{72}\\\displaystyle \tfrac{5 x \left(- 80 x^{2} - 264 x y + 408 x z - 6 x + 144 y z + 15 y + 48 z^{2} - 105 z + 8\right)}{72}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle - \tfrac{1330 x^{3}}{9} - 375 x^{2} y - 375 x^{2} z + \tfrac{4313 x^{2}}{12} - \tfrac{910 x y^{2}}{3} - 580 x y z + \tfrac{14093 x y}{24} - \tfrac{910 x z^{2}}{3} + \tfrac{14093 x z}{24} - \tfrac{2552 x}{9} - \tfrac{685 y^{3}}{9} - 195 y^{2} z + \tfrac{5387 y^{2}}{24} - 195 y z^{2} + \tfrac{5035 y z}{12} - \tfrac{15859 y}{72} - \tfrac{685 z^{3}}{9} + \tfrac{5387 z^{2}}{24} - \tfrac{15859 z}{72} + \tfrac{863}{12}\\\displaystyle \tfrac{590 x^{3}}{9} + \tfrac{665 x^{2} y}{3} + 135 x^{2} z - \tfrac{1955 x^{2}}{12} + 230 x y^{2} + 290 x y z - \tfrac{8575 x y}{24} + 80 x z^{2} - \tfrac{5095 x z}{24} + \tfrac{4705 x}{36} + \tfrac{665 y^{3}}{9} + 145 y^{2} z - \tfrac{4265 y^{2}}{24} + \tfrac{245 y z^{2}}{3} - \tfrac{2645 y z}{12} + \tfrac{9875 y}{72} + \tfrac{95 z^{3}}{9} - \tfrac{1345 z^{2}}{24} + \tfrac{5675 z}{72} - \tfrac{100}{3}\\\displaystyle \tfrac{590 x^{3}}{9} + 135 x^{2} y + \tfrac{665 x^{2} z}{3} - \tfrac{1955 x^{2}}{12} + 80 x y^{2} + 290 x y z - \tfrac{5095 x y}{24} + 230 x z^{2} - \tfrac{8575 x z}{24} + \tfrac{4705 x}{36} + \tfrac{95 y^{3}}{9} + \tfrac{245 y^{2} z}{3} - \tfrac{1345 y^{2}}{24} + 145 y z^{2} - \tfrac{2645 y z}{12} + \tfrac{5675 y}{72} + \tfrac{665 z^{3}}{9} - \tfrac{4265 z^{2}}{24} + \tfrac{9875 z}{72} - \tfrac{100}{3}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with edge 0 of the reference element.

\(\displaystyle l_{13}:\boldsymbol{v}\mapsto\boldsymbol{v}(0,\tfrac{1}{2},\tfrac{1}{2})\cdot\left(\begin{array}{c}0\\1\\0\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{13} = \begin{cases} \left(\begin{array}{c}\displaystyle \tfrac{z \left(- 480 x^{2} - 1440 x y - 1056 x z + 672 x + 2640 y z - 150 y + 3440 z^{2} - 1686 z + 97\right)}{72}\\\displaystyle \tfrac{z \left(- 1440 x y + 2640 x z - 150 x - 480 y^{2} - 1056 y z + 672 y + 3440 z^{2} - 1686 z + 97\right)}{72}\\\displaystyle \tfrac{z \left(1200 x z - 150 x + 1200 y z - 150 y + 704 z^{2} - 864 z + 97\right)}{72}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{y \left(- 480 x^{2} - 1056 x y - 1440 x z + 672 x + 3440 y^{2} + 2640 y z - 1686 y - 150 z + 97\right)}{72}\\\displaystyle \tfrac{y \left(1200 x y - 150 x + 704 y^{2} + 1200 y z - 864 y - 150 z + 97\right)}{72}\\\displaystyle \tfrac{y \left(2640 x y - 1440 x z - 150 x + 3440 y^{2} - 1056 y z - 1686 y - 480 z^{2} + 672 z + 97\right)}{72}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{x \left(704 x^{2} + 1200 x y + 1200 x z - 864 x - 150 y - 150 z + 97\right)}{72}\\\displaystyle \tfrac{430 x^{3}}{9} - \tfrac{44 x^{2} y}{3} + \tfrac{110 x^{2} z}{3} - \tfrac{233 x^{2}}{12} - \tfrac{20 x y^{2}}{3} - 20 x y z + \tfrac{16 x y}{3} - \tfrac{73 x z}{12} + \tfrac{97 x}{72} + 4 y z\\\displaystyle \tfrac{x \left(3440 x^{2} + 2640 x y - 1056 x z - 1686 x - 1440 y z - 150 y - 480 z^{2} + 672 z + 97\right)}{72}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle - \tfrac{112 x^{3}}{9} - 6 x^{2} y - 6 x^{2} z + \tfrac{56 x^{2}}{3} + \tfrac{56 x y^{2}}{3} + 24 x y z - \tfrac{167 x y}{12} + \tfrac{56 x z^{2}}{3} - \tfrac{167 x z}{12} - \tfrac{289 x}{72} + \tfrac{110 y^{3}}{9} + 30 y^{2} z - \tfrac{311 y^{2}}{12} + 30 y z^{2} - \tfrac{271 y z}{6} + \tfrac{1145 y}{72} + \tfrac{110 z^{3}}{9} - \tfrac{311 z^{2}}{12} + \tfrac{1145 z}{72} - \tfrac{53}{24}\\\displaystyle \tfrac{110 x^{3}}{9} + \tfrac{56 x^{2} y}{3} + 30 x^{2} z - \tfrac{263 x^{2}}{12} - 6 x y^{2} + 24 x y z - \tfrac{23 x y}{12} + 30 x z^{2} - \tfrac{199 x z}{6} + \tfrac{569 x}{72} - \tfrac{112 y^{3}}{9} - 6 y^{2} z + \tfrac{80 y^{2}}{3} + \tfrac{56 y z^{2}}{3} + \tfrac{73 y z}{12} - \tfrac{1153 y}{72} + \tfrac{110 z^{3}}{9} - \tfrac{215 z^{2}}{12} + \tfrac{281 z}{72} + \tfrac{43}{24}\\\displaystyle \tfrac{110 x^{3}}{9} + 30 x^{2} y + \tfrac{56 x^{2} z}{3} - \tfrac{311 x^{2}}{12} + 30 x y^{2} + 24 x y z - \tfrac{271 x y}{6} - 6 x z^{2} - \tfrac{167 x z}{12} + \tfrac{1145 x}{72} + \tfrac{110 y^{3}}{9} + \tfrac{56 y^{2} z}{3} - \tfrac{311 y^{2}}{12} - 6 y z^{2} - \tfrac{167 y z}{12} + \tfrac{1145 y}{72} - \tfrac{112 z^{3}}{9} + \tfrac{56 z^{2}}{3} - \tfrac{289 z}{72} - \tfrac{53}{24}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with edge 0 of the reference element.

\(\displaystyle l_{14}:\boldsymbol{v}\mapsto\boldsymbol{v}(0,\tfrac{1}{2},\tfrac{1}{2})\cdot\left(\begin{array}{c}0\\0\\1\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{14} = \begin{cases} \left(\begin{array}{c}\displaystyle \tfrac{z \left(- 480 x^{2} - 1440 x y - 1056 x z + 672 x + 2640 y z - 150 y + 3440 z^{2} - 1686 z + 97\right)}{72}\\\displaystyle \tfrac{z \left(- 1440 x y + 2640 x z - 150 x - 480 y^{2} - 1056 y z + 672 y + 3440 z^{2} - 1686 z + 97\right)}{72}\\\displaystyle \tfrac{z \left(1200 x z - 150 x + 1200 y z - 150 y + 704 z^{2} - 864 z + 97\right)}{72}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{y \left(- 480 x^{2} - 1056 x y - 1440 x z + 672 x + 3440 y^{2} + 2640 y z - 1686 y - 150 z + 97\right)}{72}\\\displaystyle \tfrac{y \left(1200 x y - 150 x + 704 y^{2} + 1200 y z - 864 y - 150 z + 97\right)}{72}\\\displaystyle \tfrac{y \left(2640 x y - 1440 x z - 150 x + 3440 y^{2} - 1056 y z - 1686 y - 480 z^{2} + 672 z + 97\right)}{72}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{x \left(704 x^{2} + 1200 x y + 1200 x z - 864 x - 150 y - 150 z + 97\right)}{72}\\\displaystyle \tfrac{x \left(3440 x^{2} - 1056 x y + 2640 x z - 1686 x - 480 y^{2} - 1440 y z + 672 y - 150 z + 97\right)}{72}\\\displaystyle \tfrac{430 x^{3}}{9} + \tfrac{110 x^{2} y}{3} - \tfrac{44 x^{2} z}{3} - \tfrac{233 x^{2}}{12} - 20 x y z - \tfrac{73 x y}{12} - \tfrac{20 x z^{2}}{3} + \tfrac{16 x z}{3} + \tfrac{97 x}{72} + 4 y z\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle - \tfrac{112 x^{3}}{9} - 6 x^{2} y - 6 x^{2} z + \tfrac{56 x^{2}}{3} + \tfrac{56 x y^{2}}{3} + 24 x y z - \tfrac{167 x y}{12} + \tfrac{56 x z^{2}}{3} - \tfrac{167 x z}{12} - \tfrac{289 x}{72} + \tfrac{110 y^{3}}{9} + 30 y^{2} z - \tfrac{311 y^{2}}{12} + 30 y z^{2} - \tfrac{271 y z}{6} + \tfrac{1145 y}{72} + \tfrac{110 z^{3}}{9} - \tfrac{311 z^{2}}{12} + \tfrac{1145 z}{72} - \tfrac{53}{24}\\\displaystyle \tfrac{110 x^{3}}{9} + \tfrac{56 x^{2} y}{3} + 30 x^{2} z - \tfrac{311 x^{2}}{12} - 6 x y^{2} + 24 x y z - \tfrac{167 x y}{12} + 30 x z^{2} - \tfrac{271 x z}{6} + \tfrac{1145 x}{72} - \tfrac{112 y^{3}}{9} - 6 y^{2} z + \tfrac{56 y^{2}}{3} + \tfrac{56 y z^{2}}{3} - \tfrac{167 y z}{12} - \tfrac{289 y}{72} + \tfrac{110 z^{3}}{9} - \tfrac{311 z^{2}}{12} + \tfrac{1145 z}{72} - \tfrac{53}{24}\\\displaystyle \tfrac{110 x^{3}}{9} + 30 x^{2} y + \tfrac{56 x^{2} z}{3} - \tfrac{263 x^{2}}{12} + 30 x y^{2} + 24 x y z - \tfrac{199 x y}{6} - 6 x z^{2} - \tfrac{23 x z}{12} + \tfrac{569 x}{72} + \tfrac{110 y^{3}}{9} + \tfrac{56 y^{2} z}{3} - \tfrac{215 y^{2}}{12} - 6 y z^{2} + \tfrac{73 y z}{12} + \tfrac{281 y}{72} - \tfrac{112 z^{3}}{9} + \tfrac{80 z^{2}}{3} - \tfrac{1153 z}{72} + \tfrac{43}{24}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with edge 0 of the reference element.

\(\displaystyle l_{15}:\boldsymbol{v}\mapsto\boldsymbol{v}(\tfrac{1}{2},0,\tfrac{1}{2})\cdot\left(\begin{array}{c}1\\0\\0\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{15} = \begin{cases} \left(\begin{array}{c}\displaystyle \tfrac{z \left(- 480 x^{2} - 1440 x y - 1056 x z + 672 x + 2640 y z - 150 y + 3440 z^{2} - 1686 z + 97\right)}{72}\\\displaystyle \tfrac{z \left(- 1440 x y + 2640 x z - 150 x - 480 y^{2} - 1056 y z + 672 y + 3440 z^{2} - 1686 z + 97\right)}{72}\\\displaystyle \tfrac{z \left(1200 x z - 150 x + 1200 y z - 150 y + 704 z^{2} - 864 z + 97\right)}{72}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle - \tfrac{20 x^{2} y}{3} - \tfrac{44 x y^{2}}{3} - 20 x y z + \tfrac{16 x y}{3} + 4 x z + \tfrac{430 y^{3}}{9} + \tfrac{110 y^{2} z}{3} - \tfrac{233 y^{2}}{12} - \tfrac{73 y z}{12} + \tfrac{97 y}{72}\\\displaystyle \tfrac{y \left(1200 x y - 150 x + 704 y^{2} + 1200 y z - 864 y - 150 z + 97\right)}{72}\\\displaystyle \tfrac{y \left(2640 x y - 1440 x z - 150 x + 3440 y^{2} - 1056 y z - 1686 y - 480 z^{2} + 672 z + 97\right)}{72}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{x \left(704 x^{2} + 1200 x y + 1200 x z - 864 x - 150 y - 150 z + 97\right)}{72}\\\displaystyle \tfrac{x \left(3440 x^{2} - 1056 x y + 2640 x z - 1686 x - 480 y^{2} - 1440 y z + 672 y - 150 z + 97\right)}{72}\\\displaystyle \tfrac{x \left(3440 x^{2} + 2640 x y - 1056 x z - 1686 x - 1440 y z - 150 y - 480 z^{2} + 672 z + 97\right)}{72}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle - \tfrac{112 x^{3}}{9} - 6 x^{2} y - 6 x^{2} z + \tfrac{80 x^{2}}{3} + \tfrac{56 x y^{2}}{3} + 24 x y z - \tfrac{23 x y}{12} + \tfrac{56 x z^{2}}{3} + \tfrac{73 x z}{12} - \tfrac{1153 x}{72} + \tfrac{110 y^{3}}{9} + 30 y^{2} z - \tfrac{263 y^{2}}{12} + 30 y z^{2} - \tfrac{199 y z}{6} + \tfrac{569 y}{72} + \tfrac{110 z^{3}}{9} - \tfrac{215 z^{2}}{12} + \tfrac{281 z}{72} + \tfrac{43}{24}\\\displaystyle \tfrac{110 x^{3}}{9} + \tfrac{56 x^{2} y}{3} + 30 x^{2} z - \tfrac{311 x^{2}}{12} - 6 x y^{2} + 24 x y z - \tfrac{167 x y}{12} + 30 x z^{2} - \tfrac{271 x z}{6} + \tfrac{1145 x}{72} - \tfrac{112 y^{3}}{9} - 6 y^{2} z + \tfrac{56 y^{2}}{3} + \tfrac{56 y z^{2}}{3} - \tfrac{167 y z}{12} - \tfrac{289 y}{72} + \tfrac{110 z^{3}}{9} - \tfrac{311 z^{2}}{12} + \tfrac{1145 z}{72} - \tfrac{53}{24}\\\displaystyle \tfrac{110 x^{3}}{9} + 30 x^{2} y + \tfrac{56 x^{2} z}{3} - \tfrac{311 x^{2}}{12} + 30 x y^{2} + 24 x y z - \tfrac{271 x y}{6} - 6 x z^{2} - \tfrac{167 x z}{12} + \tfrac{1145 x}{72} + \tfrac{110 y^{3}}{9} + \tfrac{56 y^{2} z}{3} - \tfrac{311 y^{2}}{12} - 6 y z^{2} - \tfrac{167 y z}{12} + \tfrac{1145 y}{72} - \tfrac{112 z^{3}}{9} + \tfrac{56 z^{2}}{3} - \tfrac{289 z}{72} - \tfrac{53}{24}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with edge 1 of the reference element.

\(\displaystyle l_{16}:\boldsymbol{v}\mapsto\boldsymbol{v}(\tfrac{1}{2},0,\tfrac{1}{2})\cdot\left(\begin{array}{c}0\\1\\0\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{16} = \begin{cases} \left(\begin{array}{c}\displaystyle \tfrac{5 z \left(48 x^{2} - 288 x y + 840 x z - 105 x + 528 y z - 30 y - 872 z^{2} + 39 z + 8\right)}{72}\\\displaystyle \tfrac{5 z \left(288 x^{2} + 144 x y + 24 x z - 237 x - 96 y^{2} - 1248 y z + 210 y + 952 z^{2} + 3 z + 2\right)}{72}\\\displaystyle \tfrac{5 z \left(- 120 x z + 15 x + 240 y z - 30 y + 136 z^{2} - 81 z + 8\right)}{72}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{5 y \left(48 x^{2} + 408 x y + 144 x z - 105 x - 80 y^{2} - 264 y z - 6 y + 15 z + 8\right)}{72}\\\displaystyle 20 x^{2} z - \tfrac{25 x y^{2}}{3} - \tfrac{11 x y}{24} + 20 x z^{2} - 16 x z - \tfrac{170 y^{3}}{9} - \tfrac{25 y^{2} z}{3} + \tfrac{61 y^{2}}{4} - \tfrac{11 y z}{24} + \tfrac{5 y}{36}\\\displaystyle \tfrac{5 y \left(- 264 x y + 144 x z + 15 x - 80 y^{2} + 408 y z - 6 y + 48 z^{2} - 105 z + 8\right)}{72}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{5 x \left(136 x^{2} + 240 x y - 120 x z - 81 x - 30 y + 15 z + 8\right)}{72}\\\displaystyle \tfrac{5 x \left(952 x^{2} - 1248 x y + 24 x z + 3 x - 96 y^{2} + 144 y z + 210 y + 288 z^{2} - 237 z + 2\right)}{72}\\\displaystyle \tfrac{5 x \left(- 872 x^{2} + 528 x y + 840 x z + 39 x - 288 y z - 30 y + 48 z^{2} - 105 z + 8\right)}{72}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{665 x^{3}}{9} + 230 x^{2} y + 145 x^{2} z - \tfrac{4265 x^{2}}{24} + \tfrac{665 x y^{2}}{3} + 290 x y z - \tfrac{8575 x y}{24} + \tfrac{245 x z^{2}}{3} - \tfrac{2645 x z}{12} + \tfrac{9875 x}{72} + \tfrac{590 y^{3}}{9} + 135 y^{2} z - \tfrac{1955 y^{2}}{12} + 80 y z^{2} - \tfrac{5095 y z}{24} + \tfrac{4705 y}{36} + \tfrac{95 z^{3}}{9} - \tfrac{1345 z^{2}}{24} + \tfrac{5675 z}{72} - \tfrac{100}{3}\\\displaystyle - \tfrac{685 x^{3}}{9} - \tfrac{910 x^{2} y}{3} - 195 x^{2} z + \tfrac{5387 x^{2}}{24} - 375 x y^{2} - 580 x y z + \tfrac{14093 x y}{24} - 195 x z^{2} + \tfrac{5035 x z}{12} - \tfrac{15859 x}{72} - \tfrac{1330 y^{3}}{9} - 375 y^{2} z + \tfrac{4313 y^{2}}{12} - \tfrac{910 y z^{2}}{3} + \tfrac{14093 y z}{24} - \tfrac{2552 y}{9} - \tfrac{685 z^{3}}{9} + \tfrac{5387 z^{2}}{24} - \tfrac{15859 z}{72} + \tfrac{863}{12}\\\displaystyle \tfrac{95 x^{3}}{9} + 80 x^{2} y + \tfrac{245 x^{2} z}{3} - \tfrac{1345 x^{2}}{24} + 135 x y^{2} + 290 x y z - \tfrac{5095 x y}{24} + 145 x z^{2} - \tfrac{2645 x z}{12} + \tfrac{5675 x}{72} + \tfrac{590 y^{3}}{9} + \tfrac{665 y^{2} z}{3} - \tfrac{1955 y^{2}}{12} + 230 y z^{2} - \tfrac{8575 y z}{24} + \tfrac{4705 y}{36} + \tfrac{665 z^{3}}{9} - \tfrac{4265 z^{2}}{24} + \tfrac{9875 z}{72} - \tfrac{100}{3}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with edge 1 of the reference element.

\(\displaystyle l_{17}:\boldsymbol{v}\mapsto\boldsymbol{v}(\tfrac{1}{2},0,\tfrac{1}{2})\cdot\left(\begin{array}{c}0\\0\\1\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{17} = \begin{cases} \left(\begin{array}{c}\displaystyle \tfrac{z \left(- 480 x^{2} - 1440 x y - 1056 x z + 672 x + 2640 y z - 150 y + 3440 z^{2} - 1686 z + 97\right)}{72}\\\displaystyle \tfrac{z \left(- 1440 x y + 2640 x z - 150 x - 480 y^{2} - 1056 y z + 672 y + 3440 z^{2} - 1686 z + 97\right)}{72}\\\displaystyle \tfrac{z \left(1200 x z - 150 x + 1200 y z - 150 y + 704 z^{2} - 864 z + 97\right)}{72}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{y \left(- 480 x^{2} - 1056 x y - 1440 x z + 672 x + 3440 y^{2} + 2640 y z - 1686 y - 150 z + 97\right)}{72}\\\displaystyle \tfrac{y \left(1200 x y - 150 x + 704 y^{2} + 1200 y z - 864 y - 150 z + 97\right)}{72}\\\displaystyle \tfrac{110 x y^{2}}{3} - 20 x y z - \tfrac{73 x y}{12} + 4 x z + \tfrac{430 y^{3}}{9} - \tfrac{44 y^{2} z}{3} - \tfrac{233 y^{2}}{12} - \tfrac{20 y z^{2}}{3} + \tfrac{16 y z}{3} + \tfrac{97 y}{72}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{x \left(704 x^{2} + 1200 x y + 1200 x z - 864 x - 150 y - 150 z + 97\right)}{72}\\\displaystyle \tfrac{x \left(3440 x^{2} - 1056 x y + 2640 x z - 1686 x - 480 y^{2} - 1440 y z + 672 y - 150 z + 97\right)}{72}\\\displaystyle \tfrac{x \left(3440 x^{2} + 2640 x y - 1056 x z - 1686 x - 1440 y z - 150 y - 480 z^{2} + 672 z + 97\right)}{72}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle - \tfrac{112 x^{3}}{9} - 6 x^{2} y - 6 x^{2} z + \tfrac{56 x^{2}}{3} + \tfrac{56 x y^{2}}{3} + 24 x y z - \tfrac{167 x y}{12} + \tfrac{56 x z^{2}}{3} - \tfrac{167 x z}{12} - \tfrac{289 x}{72} + \tfrac{110 y^{3}}{9} + 30 y^{2} z - \tfrac{311 y^{2}}{12} + 30 y z^{2} - \tfrac{271 y z}{6} + \tfrac{1145 y}{72} + \tfrac{110 z^{3}}{9} - \tfrac{311 z^{2}}{12} + \tfrac{1145 z}{72} - \tfrac{53}{24}\\\displaystyle \tfrac{110 x^{3}}{9} + \tfrac{56 x^{2} y}{3} + 30 x^{2} z - \tfrac{311 x^{2}}{12} - 6 x y^{2} + 24 x y z - \tfrac{167 x y}{12} + 30 x z^{2} - \tfrac{271 x z}{6} + \tfrac{1145 x}{72} - \tfrac{112 y^{3}}{9} - 6 y^{2} z + \tfrac{56 y^{2}}{3} + \tfrac{56 y z^{2}}{3} - \tfrac{167 y z}{12} - \tfrac{289 y}{72} + \tfrac{110 z^{3}}{9} - \tfrac{311 z^{2}}{12} + \tfrac{1145 z}{72} - \tfrac{53}{24}\\\displaystyle \tfrac{110 x^{3}}{9} + 30 x^{2} y + \tfrac{56 x^{2} z}{3} - \tfrac{215 x^{2}}{12} + 30 x y^{2} + 24 x y z - \tfrac{199 x y}{6} - 6 x z^{2} + \tfrac{73 x z}{12} + \tfrac{281 x}{72} + \tfrac{110 y^{3}}{9} + \tfrac{56 y^{2} z}{3} - \tfrac{263 y^{2}}{12} - 6 y z^{2} - \tfrac{23 y z}{12} + \tfrac{569 y}{72} - \tfrac{112 z^{3}}{9} + \tfrac{80 z^{2}}{3} - \tfrac{1153 z}{72} + \tfrac{43}{24}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with edge 1 of the reference element.

\(\displaystyle l_{18}:\boldsymbol{v}\mapsto\boldsymbol{v}(\tfrac{1}{2},\tfrac{1}{2},0)\cdot\left(\begin{array}{c}1\\0\\0\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{18} = \begin{cases} \left(\begin{array}{c}\displaystyle - \tfrac{20 x^{2} z}{3} - 20 x y z + 4 x y - \tfrac{44 x z^{2}}{3} + \tfrac{16 x z}{3} + \tfrac{110 y z^{2}}{3} - \tfrac{73 y z}{12} + \tfrac{430 z^{3}}{9} - \tfrac{233 z^{2}}{12} + \tfrac{97 z}{72}\\\displaystyle \tfrac{z \left(- 1440 x y + 2640 x z - 150 x - 480 y^{2} - 1056 y z + 672 y + 3440 z^{2} - 1686 z + 97\right)}{72}\\\displaystyle \tfrac{z \left(1200 x z - 150 x + 1200 y z - 150 y + 704 z^{2} - 864 z + 97\right)}{72}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{y \left(- 480 x^{2} - 1056 x y - 1440 x z + 672 x + 3440 y^{2} + 2640 y z - 1686 y - 150 z + 97\right)}{72}\\\displaystyle \tfrac{y \left(1200 x y - 150 x + 704 y^{2} + 1200 y z - 864 y - 150 z + 97\right)}{72}\\\displaystyle \tfrac{y \left(2640 x y - 1440 x z - 150 x + 3440 y^{2} - 1056 y z - 1686 y - 480 z^{2} + 672 z + 97\right)}{72}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{x \left(704 x^{2} + 1200 x y + 1200 x z - 864 x - 150 y - 150 z + 97\right)}{72}\\\displaystyle \tfrac{x \left(3440 x^{2} - 1056 x y + 2640 x z - 1686 x - 480 y^{2} - 1440 y z + 672 y - 150 z + 97\right)}{72}\\\displaystyle \tfrac{x \left(3440 x^{2} + 2640 x y - 1056 x z - 1686 x - 1440 y z - 150 y - 480 z^{2} + 672 z + 97\right)}{72}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle - \tfrac{112 x^{3}}{9} - 6 x^{2} y - 6 x^{2} z + \tfrac{80 x^{2}}{3} + \tfrac{56 x y^{2}}{3} + 24 x y z + \tfrac{73 x y}{12} + \tfrac{56 x z^{2}}{3} - \tfrac{23 x z}{12} - \tfrac{1153 x}{72} + \tfrac{110 y^{3}}{9} + 30 y^{2} z - \tfrac{215 y^{2}}{12} + 30 y z^{2} - \tfrac{199 y z}{6} + \tfrac{281 y}{72} + \tfrac{110 z^{3}}{9} - \tfrac{263 z^{2}}{12} + \tfrac{569 z}{72} + \tfrac{43}{24}\\\displaystyle \tfrac{110 x^{3}}{9} + \tfrac{56 x^{2} y}{3} + 30 x^{2} z - \tfrac{311 x^{2}}{12} - 6 x y^{2} + 24 x y z - \tfrac{167 x y}{12} + 30 x z^{2} - \tfrac{271 x z}{6} + \tfrac{1145 x}{72} - \tfrac{112 y^{3}}{9} - 6 y^{2} z + \tfrac{56 y^{2}}{3} + \tfrac{56 y z^{2}}{3} - \tfrac{167 y z}{12} - \tfrac{289 y}{72} + \tfrac{110 z^{3}}{9} - \tfrac{311 z^{2}}{12} + \tfrac{1145 z}{72} - \tfrac{53}{24}\\\displaystyle \tfrac{110 x^{3}}{9} + 30 x^{2} y + \tfrac{56 x^{2} z}{3} - \tfrac{311 x^{2}}{12} + 30 x y^{2} + 24 x y z - \tfrac{271 x y}{6} - 6 x z^{2} - \tfrac{167 x z}{12} + \tfrac{1145 x}{72} + \tfrac{110 y^{3}}{9} + \tfrac{56 y^{2} z}{3} - \tfrac{311 y^{2}}{12} - 6 y z^{2} - \tfrac{167 y z}{12} + \tfrac{1145 y}{72} - \tfrac{112 z^{3}}{9} + \tfrac{56 z^{2}}{3} - \tfrac{289 z}{72} - \tfrac{53}{24}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with edge 2 of the reference element.

\(\displaystyle l_{19}:\boldsymbol{v}\mapsto\boldsymbol{v}(\tfrac{1}{2},\tfrac{1}{2},0)\cdot\left(\begin{array}{c}0\\1\\0\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{19} = \begin{cases} \left(\begin{array}{c}\displaystyle \tfrac{z \left(- 480 x^{2} - 1440 x y - 1056 x z + 672 x + 2640 y z - 150 y + 3440 z^{2} - 1686 z + 97\right)}{72}\\\displaystyle - 20 x y z + 4 x y + \tfrac{110 x z^{2}}{3} - \tfrac{73 x z}{12} - \tfrac{20 y^{2} z}{3} - \tfrac{44 y z^{2}}{3} + \tfrac{16 y z}{3} + \tfrac{430 z^{3}}{9} - \tfrac{233 z^{2}}{12} + \tfrac{97 z}{72}\\\displaystyle \tfrac{z \left(1200 x z - 150 x + 1200 y z - 150 y + 704 z^{2} - 864 z + 97\right)}{72}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{y \left(- 480 x^{2} - 1056 x y - 1440 x z + 672 x + 3440 y^{2} + 2640 y z - 1686 y - 150 z + 97\right)}{72}\\\displaystyle \tfrac{y \left(1200 x y - 150 x + 704 y^{2} + 1200 y z - 864 y - 150 z + 97\right)}{72}\\\displaystyle \tfrac{y \left(2640 x y - 1440 x z - 150 x + 3440 y^{2} - 1056 y z - 1686 y - 480 z^{2} + 672 z + 97\right)}{72}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{x \left(704 x^{2} + 1200 x y + 1200 x z - 864 x - 150 y - 150 z + 97\right)}{72}\\\displaystyle \tfrac{x \left(3440 x^{2} - 1056 x y + 2640 x z - 1686 x - 480 y^{2} - 1440 y z + 672 y - 150 z + 97\right)}{72}\\\displaystyle \tfrac{x \left(3440 x^{2} + 2640 x y - 1056 x z - 1686 x - 1440 y z - 150 y - 480 z^{2} + 672 z + 97\right)}{72}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle - \tfrac{112 x^{3}}{9} - 6 x^{2} y - 6 x^{2} z + \tfrac{56 x^{2}}{3} + \tfrac{56 x y^{2}}{3} + 24 x y z - \tfrac{167 x y}{12} + \tfrac{56 x z^{2}}{3} - \tfrac{167 x z}{12} - \tfrac{289 x}{72} + \tfrac{110 y^{3}}{9} + 30 y^{2} z - \tfrac{311 y^{2}}{12} + 30 y z^{2} - \tfrac{271 y z}{6} + \tfrac{1145 y}{72} + \tfrac{110 z^{3}}{9} - \tfrac{311 z^{2}}{12} + \tfrac{1145 z}{72} - \tfrac{53}{24}\\\displaystyle \tfrac{110 x^{3}}{9} + \tfrac{56 x^{2} y}{3} + 30 x^{2} z - \tfrac{215 x^{2}}{12} - 6 x y^{2} + 24 x y z + \tfrac{73 x y}{12} + 30 x z^{2} - \tfrac{199 x z}{6} + \tfrac{281 x}{72} - \tfrac{112 y^{3}}{9} - 6 y^{2} z + \tfrac{80 y^{2}}{3} + \tfrac{56 y z^{2}}{3} - \tfrac{23 y z}{12} - \tfrac{1153 y}{72} + \tfrac{110 z^{3}}{9} - \tfrac{263 z^{2}}{12} + \tfrac{569 z}{72} + \tfrac{43}{24}\\\displaystyle \tfrac{110 x^{3}}{9} + 30 x^{2} y + \tfrac{56 x^{2} z}{3} - \tfrac{311 x^{2}}{12} + 30 x y^{2} + 24 x y z - \tfrac{271 x y}{6} - 6 x z^{2} - \tfrac{167 x z}{12} + \tfrac{1145 x}{72} + \tfrac{110 y^{3}}{9} + \tfrac{56 y^{2} z}{3} - \tfrac{311 y^{2}}{12} - 6 y z^{2} - \tfrac{167 y z}{12} + \tfrac{1145 y}{72} - \tfrac{112 z^{3}}{9} + \tfrac{56 z^{2}}{3} - \tfrac{289 z}{72} - \tfrac{53}{24}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with edge 2 of the reference element.

\(\displaystyle l_{20}:\boldsymbol{v}\mapsto\boldsymbol{v}(\tfrac{1}{2},\tfrac{1}{2},0)\cdot\left(\begin{array}{c}0\\0\\1\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{20} = \begin{cases} \left(\begin{array}{c}\displaystyle \tfrac{5 z \left(48 x^{2} + 144 x y + 408 x z - 105 x - 264 y z + 15 y - 80 z^{2} - 6 z + 8\right)}{72}\\\displaystyle \tfrac{5 z \left(144 x y - 264 x z + 15 x + 48 y^{2} + 408 y z - 105 y - 80 z^{2} - 6 z + 8\right)}{72}\\\displaystyle 20 x^{2} y + 20 x y^{2} - 16 x y - \tfrac{25 x z^{2}}{3} - \tfrac{11 x z}{24} - \tfrac{25 y z^{2}}{3} - \tfrac{11 y z}{24} - \tfrac{170 z^{3}}{9} + \tfrac{61 z^{2}}{4} + \tfrac{5 z}{36}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{5 y \left(48 x^{2} + 840 x y - 288 x z - 105 x - 872 y^{2} + 528 y z + 39 y - 30 z + 8\right)}{72}\\\displaystyle \tfrac{5 y \left(- 120 x y + 15 x + 136 y^{2} + 240 y z - 81 y - 30 z + 8\right)}{72}\\\displaystyle \tfrac{5 y \left(288 x^{2} + 24 x y + 144 x z - 237 x + 952 y^{2} - 1248 y z + 3 y - 96 z^{2} + 210 z + 2\right)}{72}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{5 x \left(136 x^{2} - 120 x y + 240 x z - 81 x + 15 y - 30 z + 8\right)}{72}\\\displaystyle \tfrac{5 x \left(- 872 x^{2} + 840 x y + 528 x z + 39 x + 48 y^{2} - 288 y z - 105 y - 30 z + 8\right)}{72}\\\displaystyle \tfrac{5 x \left(952 x^{2} + 24 x y - 1248 x z + 3 x + 288 y^{2} + 144 y z - 237 y - 96 z^{2} + 210 z + 2\right)}{72}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{665 x^{3}}{9} + 145 x^{2} y + 230 x^{2} z - \tfrac{4265 x^{2}}{24} + \tfrac{245 x y^{2}}{3} + 290 x y z - \tfrac{2645 x y}{12} + \tfrac{665 x z^{2}}{3} - \tfrac{8575 x z}{24} + \tfrac{9875 x}{72} + \tfrac{95 y^{3}}{9} + 80 y^{2} z - \tfrac{1345 y^{2}}{24} + 135 y z^{2} - \tfrac{5095 y z}{24} + \tfrac{5675 y}{72} + \tfrac{590 z^{3}}{9} - \tfrac{1955 z^{2}}{12} + \tfrac{4705 z}{36} - \tfrac{100}{3}\\\displaystyle \tfrac{95 x^{3}}{9} + \tfrac{245 x^{2} y}{3} + 80 x^{2} z - \tfrac{1345 x^{2}}{24} + 145 x y^{2} + 290 x y z - \tfrac{2645 x y}{12} + 135 x z^{2} - \tfrac{5095 x z}{24} + \tfrac{5675 x}{72} + \tfrac{665 y^{3}}{9} + 230 y^{2} z - \tfrac{4265 y^{2}}{24} + \tfrac{665 y z^{2}}{3} - \tfrac{8575 y z}{24} + \tfrac{9875 y}{72} + \tfrac{590 z^{3}}{9} - \tfrac{1955 z^{2}}{12} + \tfrac{4705 z}{36} - \tfrac{100}{3}\\\displaystyle - \tfrac{685 x^{3}}{9} - 195 x^{2} y - \tfrac{910 x^{2} z}{3} + \tfrac{5387 x^{2}}{24} - 195 x y^{2} - 580 x y z + \tfrac{5035 x y}{12} - 375 x z^{2} + \tfrac{14093 x z}{24} - \tfrac{15859 x}{72} - \tfrac{685 y^{3}}{9} - \tfrac{910 y^{2} z}{3} + \tfrac{5387 y^{2}}{24} - 375 y z^{2} + \tfrac{14093 y z}{24} - \tfrac{15859 y}{72} - \tfrac{1330 z^{3}}{9} + \tfrac{4313 z^{2}}{12} - \tfrac{2552 z}{9} + \tfrac{863}{12}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with edge 2 of the reference element.

\(\displaystyle l_{21}:\boldsymbol{v}\mapsto\boldsymbol{v}(0,0,\tfrac{1}{2})\cdot\left(\begin{array}{c}1\\0\\0\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{21} = \begin{cases} \left(\begin{array}{c}\displaystyle \tfrac{z \left(720 x y - 1728 x z + 126 x + 480 y^{2} - 840 y z - 345 y + 440 z^{2} + 567 z - 29\right)}{24}\\\displaystyle \tfrac{z \left(240 y^{2} + 1752 y z - 399 y - 2600 z^{2} + 627 z - 19\right)}{24}\\\displaystyle \tfrac{z \left(- 600 y z + 75 y - 8 z^{2} + 153 z - 19\right)}{24}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle - 72 x y^{2} + 30 x y z + \tfrac{37 x y}{4} - 4 x z + \tfrac{55 y^{3}}{3} - 35 y^{2} z + \tfrac{253 y^{2}}{8} + 20 y z^{2} - \tfrac{147 y z}{8} - \tfrac{125 y}{24} - 4 z^{2} + 4 z\\\displaystyle \tfrac{y \left(- 8 y^{2} - 600 y z + 153 y + 75 z - 19\right)}{24}\\\displaystyle \tfrac{y \left(- 2600 y^{2} + 1752 y z + 627 y + 240 z^{2} - 399 z - 19\right)}{24}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle - \tfrac{86 x^{3}}{3} - 25 x^{2} y - 25 x^{2} z + \tfrac{125 x^{2}}{4} + \tfrac{77 x y}{8} + \tfrac{13 x z}{8} - \tfrac{125 x}{24} + 20 y^{2} z + 20 y z^{2} - 24 y z - 4 z^{2} + 4 z\\\displaystyle \tfrac{x \left(- 1280 x^{2} + 1032 x y - 1320 x z + 552 x + 240 y^{2} + 720 y z - 399 y + 75 z - 19\right)}{24}\\\displaystyle \tfrac{x \left(- 1280 x^{2} - 1320 x y + 1032 x z + 552 x + 720 y z + 75 y + 240 z^{2} - 399 z - 19\right)}{24}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle - \tfrac{406 x^{3}}{3} - 369 x^{2} y - 369 x^{2} z + \tfrac{1347 x^{2}}{4} - 322 x y^{2} - 604 x y z + \tfrac{4713 x y}{8} - 322 x z^{2} + \tfrac{4713 x z}{8} - \tfrac{6517 x}{24} - \tfrac{265 y^{3}}{3} - 225 y^{2} z + \tfrac{1939 y^{2}}{8} - 225 y z^{2} + \tfrac{1779 y z}{4} - \tfrac{1345 y}{6} - \tfrac{265 z^{3}}{3} + \tfrac{1939 z^{2}}{8} - \tfrac{1345 z}{6} + \tfrac{561}{8}\\\displaystyle \tfrac{160 x^{3}}{3} + 203 x^{2} y + 105 x^{2} z - 137 x^{2} + 236 x y^{2} + 266 x y z - \tfrac{2747 x y}{8} + 50 x z^{2} - \tfrac{1337 x z}{8} + \tfrac{2755 x}{24} + \tfrac{259 y^{3}}{3} + 151 y^{2} z - \tfrac{1571 y^{2}}{8} + 63 y z^{2} - \tfrac{413 y z}{2} + \tfrac{847 y}{6} - \tfrac{5 z^{3}}{3} - \tfrac{241 z^{2}}{8} + \tfrac{755 z}{12} - \tfrac{249}{8}\\\displaystyle \tfrac{160 x^{3}}{3} + 105 x^{2} y + 203 x^{2} z - 137 x^{2} + 50 x y^{2} + 266 x y z - \tfrac{1337 x y}{8} + 236 x z^{2} - \tfrac{2747 x z}{8} + \tfrac{2755 x}{24} - \tfrac{5 y^{3}}{3} + 63 y^{2} z - \tfrac{241 y^{2}}{8} + 151 y z^{2} - \tfrac{413 y z}{2} + \tfrac{755 y}{12} + \tfrac{259 z^{3}}{3} - \tfrac{1571 z^{2}}{8} + \tfrac{847 z}{6} - \tfrac{249}{8}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with edge 3 of the reference element.

\(\displaystyle l_{22}:\boldsymbol{v}\mapsto\boldsymbol{v}(0,0,\tfrac{1}{2})\cdot\left(\begin{array}{c}0\\1\\0\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{22} = \begin{cases} \left(\begin{array}{c}\displaystyle \tfrac{z \left(240 x^{2} + 1752 x z - 399 x - 2600 z^{2} + 627 z - 19\right)}{24}\\\displaystyle \tfrac{z \left(480 x^{2} + 720 x y - 840 x z - 345 x - 1728 y z + 126 y + 440 z^{2} + 567 z - 29\right)}{24}\\\displaystyle \tfrac{z \left(- 600 x z + 75 x - 8 z^{2} + 153 z - 19\right)}{24}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{y \left(240 x^{2} + 1032 x y + 720 x z - 399 x - 1280 y^{2} - 1320 y z + 552 y + 75 z - 19\right)}{24}\\\displaystyle 20 x^{2} z - 25 x y^{2} + \tfrac{77 x y}{8} + 20 x z^{2} - 24 x z - \tfrac{86 y^{3}}{3} - 25 y^{2} z + \tfrac{125 y^{2}}{4} + \tfrac{13 y z}{8} - \tfrac{125 y}{24} - 4 z^{2} + 4 z\\\displaystyle \tfrac{y \left(- 1320 x y + 720 x z + 75 x - 1280 y^{2} + 1032 y z + 552 y + 240 z^{2} - 399 z - 19\right)}{24}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{x \left(- 8 x^{2} - 600 x z + 153 x + 75 z - 19\right)}{24}\\\displaystyle \tfrac{55 x^{3}}{3} - 72 x^{2} y - 35 x^{2} z + \tfrac{253 x^{2}}{8} + 30 x y z + \tfrac{37 x y}{4} + 20 x z^{2} - \tfrac{147 x z}{8} - \tfrac{125 x}{24} - 4 y z - 4 z^{2} + 4 z\\\displaystyle \tfrac{x \left(- 2600 x^{2} + 1752 x z + 627 x + 240 z^{2} - 399 z - 19\right)}{24}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{259 x^{3}}{3} + 236 x^{2} y + 151 x^{2} z - \tfrac{1571 x^{2}}{8} + 203 x y^{2} + 266 x y z - \tfrac{2747 x y}{8} + 63 x z^{2} - \tfrac{413 x z}{2} + \tfrac{847 x}{6} + \tfrac{160 y^{3}}{3} + 105 y^{2} z - 137 y^{2} + 50 y z^{2} - \tfrac{1337 y z}{8} + \tfrac{2755 y}{24} - \tfrac{5 z^{3}}{3} - \tfrac{241 z^{2}}{8} + \tfrac{755 z}{12} - \tfrac{249}{8}\\\displaystyle - \tfrac{265 x^{3}}{3} - 322 x^{2} y - 225 x^{2} z + \tfrac{1939 x^{2}}{8} - 369 x y^{2} - 604 x y z + \tfrac{4713 x y}{8} - 225 x z^{2} + \tfrac{1779 x z}{4} - \tfrac{1345 x}{6} - \tfrac{406 y^{3}}{3} - 369 y^{2} z + \tfrac{1347 y^{2}}{4} - 322 y z^{2} + \tfrac{4713 y z}{8} - \tfrac{6517 y}{24} - \tfrac{265 z^{3}}{3} + \tfrac{1939 z^{2}}{8} - \tfrac{1345 z}{6} + \tfrac{561}{8}\\\displaystyle - \tfrac{5 x^{3}}{3} + 50 x^{2} y + 63 x^{2} z - \tfrac{241 x^{2}}{8} + 105 x y^{2} + 266 x y z - \tfrac{1337 x y}{8} + 151 x z^{2} - \tfrac{413 x z}{2} + \tfrac{755 x}{12} + \tfrac{160 y^{3}}{3} + 203 y^{2} z - 137 y^{2} + 236 y z^{2} - \tfrac{2747 y z}{8} + \tfrac{2755 y}{24} + \tfrac{259 z^{3}}{3} - \tfrac{1571 z^{2}}{8} + \tfrac{847 z}{6} - \tfrac{249}{8}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with edge 3 of the reference element.

\(\displaystyle l_{23}:\boldsymbol{v}\mapsto\boldsymbol{v}(0,0,\tfrac{1}{2})\cdot\left(\begin{array}{c}0\\0\\1\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{23} = \begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 4 x y - 4 x z + 8 y^{2} - 4 y z - 4 y - 4 z^{2} + 4 z\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 8 x^{2} + 4 x y - 4 x z - 4 x - 4 y z - 4 z^{2} + 4 z\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with edge 3 of the reference element.

\(\displaystyle l_{24}:\boldsymbol{v}\mapsto\boldsymbol{v}(0,\tfrac{1}{2},0)\cdot\left(\begin{array}{c}1\\0\\0\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{24} = \begin{cases} \left(\begin{array}{c}\displaystyle 30 x y z - 4 x y - 72 x z^{2} + \tfrac{37 x z}{4} + 20 y^{2} z - 4 y^{2} - 35 y z^{2} - \tfrac{147 y z}{8} + 4 y + \tfrac{55 z^{3}}{3} + \tfrac{253 z^{2}}{8} - \tfrac{125 z}{24}\\\displaystyle \tfrac{z \left(240 y^{2} + 1752 y z - 399 y - 2600 z^{2} + 627 z - 19\right)}{24}\\\displaystyle \tfrac{z \left(- 600 y z + 75 y - 8 z^{2} + 153 z - 19\right)}{24}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{y \left(- 1728 x y + 720 x z + 126 x + 440 y^{2} - 840 y z + 567 y + 480 z^{2} - 345 z - 29\right)}{24}\\\displaystyle \tfrac{y \left(- 8 y^{2} - 600 y z + 153 y + 75 z - 19\right)}{24}\\\displaystyle \tfrac{y \left(- 2600 y^{2} + 1752 y z + 627 y + 240 z^{2} - 399 z - 19\right)}{24}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle - \tfrac{86 x^{3}}{3} - 25 x^{2} y - 25 x^{2} z + \tfrac{125 x^{2}}{4} + \tfrac{13 x y}{8} + \tfrac{77 x z}{8} - \tfrac{125 x}{24} + 20 y^{2} z - 4 y^{2} + 20 y z^{2} - 24 y z + 4 y\\\displaystyle \tfrac{x \left(- 1280 x^{2} + 1032 x y - 1320 x z + 552 x + 240 y^{2} + 720 y z - 399 y + 75 z - 19\right)}{24}\\\displaystyle \tfrac{x \left(- 1280 x^{2} - 1320 x y + 1032 x z + 552 x + 720 y z + 75 y + 240 z^{2} - 399 z - 19\right)}{24}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle - \tfrac{406 x^{3}}{3} - 369 x^{2} y - 369 x^{2} z + \tfrac{1347 x^{2}}{4} - 322 x y^{2} - 604 x y z + \tfrac{4713 x y}{8} - 322 x z^{2} + \tfrac{4713 x z}{8} - \tfrac{6517 x}{24} - \tfrac{265 y^{3}}{3} - 225 y^{2} z + \tfrac{1939 y^{2}}{8} - 225 y z^{2} + \tfrac{1779 y z}{4} - \tfrac{1345 y}{6} - \tfrac{265 z^{3}}{3} + \tfrac{1939 z^{2}}{8} - \tfrac{1345 z}{6} + \tfrac{561}{8}\\\displaystyle \tfrac{160 x^{3}}{3} + 203 x^{2} y + 105 x^{2} z - 137 x^{2} + 236 x y^{2} + 266 x y z - \tfrac{2747 x y}{8} + 50 x z^{2} - \tfrac{1337 x z}{8} + \tfrac{2755 x}{24} + \tfrac{259 y^{3}}{3} + 151 y^{2} z - \tfrac{1571 y^{2}}{8} + 63 y z^{2} - \tfrac{413 y z}{2} + \tfrac{847 y}{6} - \tfrac{5 z^{3}}{3} - \tfrac{241 z^{2}}{8} + \tfrac{755 z}{12} - \tfrac{249}{8}\\\displaystyle \tfrac{160 x^{3}}{3} + 105 x^{2} y + 203 x^{2} z - 137 x^{2} + 50 x y^{2} + 266 x y z - \tfrac{1337 x y}{8} + 236 x z^{2} - \tfrac{2747 x z}{8} + \tfrac{2755 x}{24} - \tfrac{5 y^{3}}{3} + 63 y^{2} z - \tfrac{241 y^{2}}{8} + 151 y z^{2} - \tfrac{413 y z}{2} + \tfrac{755 y}{12} + \tfrac{259 z^{3}}{3} - \tfrac{1571 z^{2}}{8} + \tfrac{847 z}{6} - \tfrac{249}{8}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with edge 4 of the reference element.

\(\displaystyle l_{25}:\boldsymbol{v}\mapsto\boldsymbol{v}(0,\tfrac{1}{2},0)\cdot\left(\begin{array}{c}0\\1\\0\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{25} = \begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle - 4 x y + 4 x z - 4 y^{2} - 4 y z + 4 y + 8 z^{2} - 4 z\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 8 x^{2} - 4 x y + 4 x z - 4 x - 4 y^{2} - 4 y z + 4 y\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with edge 4 of the reference element.

\(\displaystyle l_{26}:\boldsymbol{v}\mapsto\boldsymbol{v}(0,\tfrac{1}{2},0)\cdot\left(\begin{array}{c}0\\0\\1\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{26} = \begin{cases} \left(\begin{array}{c}\displaystyle \tfrac{z \left(240 x^{2} + 720 x y + 1032 x z - 399 x - 1320 y z + 75 y - 1280 z^{2} + 552 z - 19\right)}{24}\\\displaystyle \tfrac{z \left(720 x y - 1320 x z + 75 x + 240 y^{2} + 1032 y z - 399 y - 1280 z^{2} + 552 z - 19\right)}{24}\\\displaystyle 20 x^{2} y + 20 x y^{2} - 24 x y - 25 x z^{2} + \tfrac{77 x z}{8} - 4 y^{2} - 25 y z^{2} + \tfrac{13 y z}{8} + 4 y - \tfrac{86 z^{3}}{3} + \tfrac{125 z^{2}}{4} - \tfrac{125 z}{24}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{y \left(240 x^{2} + 1752 x y - 399 x - 2600 y^{2} + 627 y - 19\right)}{24}\\\displaystyle \tfrac{y \left(- 600 x y + 75 x - 8 y^{2} + 153 y - 19\right)}{24}\\\displaystyle \tfrac{y \left(480 x^{2} - 840 x y + 720 x z - 345 x + 440 y^{2} - 1728 y z + 567 y + 126 z - 29\right)}{24}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{x \left(- 8 x^{2} - 600 x y + 153 x + 75 y - 19\right)}{24}\\\displaystyle \tfrac{x \left(- 2600 x^{2} + 1752 x y + 627 x + 240 y^{2} - 399 y - 19\right)}{24}\\\displaystyle \tfrac{55 x^{3}}{3} - 35 x^{2} y - 72 x^{2} z + \tfrac{253 x^{2}}{8} + 20 x y^{2} + 30 x y z - \tfrac{147 x y}{8} + \tfrac{37 x z}{4} - \tfrac{125 x}{24} - 4 y^{2} - 4 y z + 4 y\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{259 x^{3}}{3} + 151 x^{2} y + 236 x^{2} z - \tfrac{1571 x^{2}}{8} + 63 x y^{2} + 266 x y z - \tfrac{413 x y}{2} + 203 x z^{2} - \tfrac{2747 x z}{8} + \tfrac{847 x}{6} - \tfrac{5 y^{3}}{3} + 50 y^{2} z - \tfrac{241 y^{2}}{8} + 105 y z^{2} - \tfrac{1337 y z}{8} + \tfrac{755 y}{12} + \tfrac{160 z^{3}}{3} - 137 z^{2} + \tfrac{2755 z}{24} - \tfrac{249}{8}\\\displaystyle - \tfrac{5 x^{3}}{3} + 63 x^{2} y + 50 x^{2} z - \tfrac{241 x^{2}}{8} + 151 x y^{2} + 266 x y z - \tfrac{413 x y}{2} + 105 x z^{2} - \tfrac{1337 x z}{8} + \tfrac{755 x}{12} + \tfrac{259 y^{3}}{3} + 236 y^{2} z - \tfrac{1571 y^{2}}{8} + 203 y z^{2} - \tfrac{2747 y z}{8} + \tfrac{847 y}{6} + \tfrac{160 z^{3}}{3} - 137 z^{2} + \tfrac{2755 z}{24} - \tfrac{249}{8}\\\displaystyle - \tfrac{265 x^{3}}{3} - 225 x^{2} y - 322 x^{2} z + \tfrac{1939 x^{2}}{8} - 225 x y^{2} - 604 x y z + \tfrac{1779 x y}{4} - 369 x z^{2} + \tfrac{4713 x z}{8} - \tfrac{1345 x}{6} - \tfrac{265 y^{3}}{3} - 322 y^{2} z + \tfrac{1939 y^{2}}{8} - 369 y z^{2} + \tfrac{4713 y z}{8} - \tfrac{1345 y}{6} - \tfrac{406 z^{3}}{3} + \tfrac{1347 z^{2}}{4} - \tfrac{6517 z}{24} + \tfrac{561}{8}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with edge 4 of the reference element.

\(\displaystyle l_{27}:\boldsymbol{v}\mapsto\boldsymbol{v}(\tfrac{1}{2},0,0)\cdot\left(\begin{array}{c}1\\0\\0\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{27} = \begin{cases} \left(\begin{array}{c}\displaystyle - 4 x^{2} - 4 x y - 4 x z + 4 x + 4 y z + 8 z^{2} - 4 z\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle - 4 x^{2} - 4 x y - 4 x z + 4 x + 8 y^{2} + 4 y z - 4 y\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with edge 5 of the reference element.

\(\displaystyle l_{28}:\boldsymbol{v}\mapsto\boldsymbol{v}(\tfrac{1}{2},0,0)\cdot\left(\begin{array}{c}0\\1\\0\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{28} = \begin{cases} \left(\begin{array}{c}\displaystyle \tfrac{z \left(240 x^{2} + 1752 x z - 399 x - 2600 z^{2} + 627 z - 19\right)}{24}\\\displaystyle 20 x^{2} z - 4 x^{2} + 30 x y z - 4 x y - 35 x z^{2} - \tfrac{147 x z}{8} + 4 x - 72 y z^{2} + \tfrac{37 y z}{4} + \tfrac{55 z^{3}}{3} + \tfrac{253 z^{2}}{8} - \tfrac{125 z}{24}\\\displaystyle \tfrac{z \left(- 600 x z + 75 x - 8 z^{2} + 153 z - 19\right)}{24}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{y \left(240 x^{2} + 1032 x y + 720 x z - 399 x - 1280 y^{2} - 1320 y z + 552 y + 75 z - 19\right)}{24}\\\displaystyle 20 x^{2} z - 4 x^{2} - 25 x y^{2} + \tfrac{13 x y}{8} + 20 x z^{2} - 24 x z + 4 x - \tfrac{86 y^{3}}{3} - 25 y^{2} z + \tfrac{125 y^{2}}{4} + \tfrac{77 y z}{8} - \tfrac{125 y}{24}\\\displaystyle \tfrac{y \left(- 1320 x y + 720 x z + 75 x - 1280 y^{2} + 1032 y z + 552 y + 240 z^{2} - 399 z - 19\right)}{24}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{x \left(- 8 x^{2} - 600 x z + 153 x + 75 z - 19\right)}{24}\\\displaystyle \tfrac{x \left(440 x^{2} - 1728 x y - 840 x z + 567 x + 720 y z + 126 y + 480 z^{2} - 345 z - 29\right)}{24}\\\displaystyle \tfrac{x \left(- 2600 x^{2} + 1752 x z + 627 x + 240 z^{2} - 399 z - 19\right)}{24}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{259 x^{3}}{3} + 236 x^{2} y + 151 x^{2} z - \tfrac{1571 x^{2}}{8} + 203 x y^{2} + 266 x y z - \tfrac{2747 x y}{8} + 63 x z^{2} - \tfrac{413 x z}{2} + \tfrac{847 x}{6} + \tfrac{160 y^{3}}{3} + 105 y^{2} z - 137 y^{2} + 50 y z^{2} - \tfrac{1337 y z}{8} + \tfrac{2755 y}{24} - \tfrac{5 z^{3}}{3} - \tfrac{241 z^{2}}{8} + \tfrac{755 z}{12} - \tfrac{249}{8}\\\displaystyle - \tfrac{265 x^{3}}{3} - 322 x^{2} y - 225 x^{2} z + \tfrac{1939 x^{2}}{8} - 369 x y^{2} - 604 x y z + \tfrac{4713 x y}{8} - 225 x z^{2} + \tfrac{1779 x z}{4} - \tfrac{1345 x}{6} - \tfrac{406 y^{3}}{3} - 369 y^{2} z + \tfrac{1347 y^{2}}{4} - 322 y z^{2} + \tfrac{4713 y z}{8} - \tfrac{6517 y}{24} - \tfrac{265 z^{3}}{3} + \tfrac{1939 z^{2}}{8} - \tfrac{1345 z}{6} + \tfrac{561}{8}\\\displaystyle - \tfrac{5 x^{3}}{3} + 50 x^{2} y + 63 x^{2} z - \tfrac{241 x^{2}}{8} + 105 x y^{2} + 266 x y z - \tfrac{1337 x y}{8} + 151 x z^{2} - \tfrac{413 x z}{2} + \tfrac{755 x}{12} + \tfrac{160 y^{3}}{3} + 203 y^{2} z - 137 y^{2} + 236 y z^{2} - \tfrac{2747 y z}{8} + \tfrac{2755 y}{24} + \tfrac{259 z^{3}}{3} - \tfrac{1571 z^{2}}{8} + \tfrac{847 z}{6} - \tfrac{249}{8}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with edge 5 of the reference element.

\(\displaystyle l_{29}:\boldsymbol{v}\mapsto\boldsymbol{v}(\tfrac{1}{2},0,0)\cdot\left(\begin{array}{c}0\\0\\1\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{29} = \begin{cases} \left(\begin{array}{c}\displaystyle \tfrac{z \left(240 x^{2} + 720 x y + 1032 x z - 399 x - 1320 y z + 75 y - 1280 z^{2} + 552 z - 19\right)}{24}\\\displaystyle \tfrac{z \left(720 x y - 1320 x z + 75 x + 240 y^{2} + 1032 y z - 399 y - 1280 z^{2} + 552 z - 19\right)}{24}\\\displaystyle 20 x^{2} y - 4 x^{2} + 20 x y^{2} - 24 x y - 25 x z^{2} + \tfrac{13 x z}{8} + 4 x - 25 y z^{2} + \tfrac{77 y z}{8} - \tfrac{86 z^{3}}{3} + \tfrac{125 z^{2}}{4} - \tfrac{125 z}{24}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{y \left(240 x^{2} + 1752 x y - 399 x - 2600 y^{2} + 627 y - 19\right)}{24}\\\displaystyle \tfrac{y \left(- 600 x y + 75 x - 8 y^{2} + 153 y - 19\right)}{24}\\\displaystyle 20 x^{2} y - 4 x^{2} - 35 x y^{2} + 30 x y z - \tfrac{147 x y}{8} - 4 x z + 4 x + \tfrac{55 y^{3}}{3} - 72 y^{2} z + \tfrac{253 y^{2}}{8} + \tfrac{37 y z}{4} - \tfrac{125 y}{24}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{x \left(- 8 x^{2} - 600 x y + 153 x + 75 y - 19\right)}{24}\\\displaystyle \tfrac{x \left(- 2600 x^{2} + 1752 x y + 627 x + 240 y^{2} - 399 y - 19\right)}{24}\\\displaystyle \tfrac{x \left(440 x^{2} - 840 x y - 1728 x z + 567 x + 480 y^{2} + 720 y z - 345 y + 126 z - 29\right)}{24}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{259 x^{3}}{3} + 151 x^{2} y + 236 x^{2} z - \tfrac{1571 x^{2}}{8} + 63 x y^{2} + 266 x y z - \tfrac{413 x y}{2} + 203 x z^{2} - \tfrac{2747 x z}{8} + \tfrac{847 x}{6} - \tfrac{5 y^{3}}{3} + 50 y^{2} z - \tfrac{241 y^{2}}{8} + 105 y z^{2} - \tfrac{1337 y z}{8} + \tfrac{755 y}{12} + \tfrac{160 z^{3}}{3} - 137 z^{2} + \tfrac{2755 z}{24} - \tfrac{249}{8}\\\displaystyle - \tfrac{5 x^{3}}{3} + 63 x^{2} y + 50 x^{2} z - \tfrac{241 x^{2}}{8} + 151 x y^{2} + 266 x y z - \tfrac{413 x y}{2} + 105 x z^{2} - \tfrac{1337 x z}{8} + \tfrac{755 x}{12} + \tfrac{259 y^{3}}{3} + 236 y^{2} z - \tfrac{1571 y^{2}}{8} + 203 y z^{2} - \tfrac{2747 y z}{8} + \tfrac{847 y}{6} + \tfrac{160 z^{3}}{3} - 137 z^{2} + \tfrac{2755 z}{24} - \tfrac{249}{8}\\\displaystyle - \tfrac{265 x^{3}}{3} - 225 x^{2} y - 322 x^{2} z + \tfrac{1939 x^{2}}{8} - 225 x y^{2} - 604 x y z + \tfrac{1779 x y}{4} - 369 x z^{2} + \tfrac{4713 x z}{8} - \tfrac{1345 x}{6} - \tfrac{265 y^{3}}{3} - 322 y^{2} z + \tfrac{1939 y^{2}}{8} - 369 y z^{2} + \tfrac{4713 y z}{8} - \tfrac{1345 y}{6} - \tfrac{406 z^{3}}{3} + \tfrac{1347 z^{2}}{4} - \tfrac{6517 z}{24} + \tfrac{561}{8}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with edge 5 of the reference element.

\(\displaystyle l_{30}:\boldsymbol{v}\mapsto\boldsymbol{v}(\tfrac{1}{8},\tfrac{1}{8},\tfrac{1}{8})\cdot\left(\begin{array}{c}1\\0\\0\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{30} = \begin{cases} \left(\begin{array}{c}\displaystyle 16 z \left(- x - y - 2 z + 1\right)\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 16 y \left(- x - 2 y - z + 1\right)\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 16 x \left(- 2 x - y - z + 1\right)\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with volume 0 of the reference element.

\(\displaystyle l_{31}:\boldsymbol{v}\mapsto\boldsymbol{v}(\tfrac{1}{8},\tfrac{1}{8},\tfrac{1}{8})\cdot\left(\begin{array}{c}0\\1\\0\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{31} = \begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 16 z \left(- x - y - 2 z + 1\right)\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 16 y \left(- x - 2 y - z + 1\right)\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 16 x \left(- 2 x - y - z + 1\right)\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with volume 0 of the reference element.

\(\displaystyle l_{32}:\boldsymbol{v}\mapsto\boldsymbol{v}(\tfrac{1}{8},\tfrac{1}{8},\tfrac{1}{8})\cdot\left(\begin{array}{c}0\\0\\1\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{32} = \begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 16 z \left(- x - y - 2 z + 1\right)\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 16 y \left(- x - 2 y - z + 1\right)\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 16 x \left(- 2 x - y - z + 1\right)\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with volume 0 of the reference element.

\(\displaystyle l_{33}:\boldsymbol{v}\mapsto\boldsymbol{v}(\tfrac{5}{8},\tfrac{1}{8},\tfrac{1}{8})\cdot\left(\begin{array}{c}1\\0\\0\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{33} = \begin{cases} \left(\begin{array}{c}\displaystyle 16 z \left(x - z\right)\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 16 y \left(x - y\right)\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle - 32 x^{2} - 48 x y - 48 x z + 48 x - 16 y^{2} - 32 y z + 32 y - 16 z^{2} + 32 z - 16\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with volume 0 of the reference element.

\(\displaystyle l_{34}:\boldsymbol{v}\mapsto\boldsymbol{v}(\tfrac{5}{8},\tfrac{1}{8},\tfrac{1}{8})\cdot\left(\begin{array}{c}0\\1\\0\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{34} = \begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 16 z \left(x - z\right)\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 16 y \left(x - y\right)\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle - 32 x^{2} - 48 x y - 48 x z + 48 x - 16 y^{2} - 32 y z + 32 y - 16 z^{2} + 32 z - 16\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with volume 0 of the reference element.

\(\displaystyle l_{35}:\boldsymbol{v}\mapsto\boldsymbol{v}(\tfrac{5}{8},\tfrac{1}{8},\tfrac{1}{8})\cdot\left(\begin{array}{c}0\\0\\1\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{35} = \begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 16 z \left(x - z\right)\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 16 y \left(x - y\right)\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle - 32 x^{2} - 48 x y - 48 x z + 48 x - 16 y^{2} - 32 y z + 32 y - 16 z^{2} + 32 z - 16\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with volume 0 of the reference element.

\(\displaystyle l_{36}:\boldsymbol{v}\mapsto\boldsymbol{v}(\tfrac{1}{8},\tfrac{5}{8},\tfrac{1}{8})\cdot\left(\begin{array}{c}1\\0\\0\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{36} = \begin{cases} \left(\begin{array}{c}\displaystyle 16 z \left(y - z\right)\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 16 x \left(- x + y\right)\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle - 16 x^{2} - 48 x y - 32 x z + 32 x - 32 y^{2} - 48 y z + 48 y - 16 z^{2} + 32 z - 16\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with volume 0 of the reference element.

\(\displaystyle l_{37}:\boldsymbol{v}\mapsto\boldsymbol{v}(\tfrac{1}{8},\tfrac{5}{8},\tfrac{1}{8})\cdot\left(\begin{array}{c}0\\1\\0\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{37} = \begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 16 z \left(y - z\right)\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 16 x \left(- x + y\right)\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle - 16 x^{2} - 48 x y - 32 x z + 32 x - 32 y^{2} - 48 y z + 48 y - 16 z^{2} + 32 z - 16\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with volume 0 of the reference element.

\(\displaystyle l_{38}:\boldsymbol{v}\mapsto\boldsymbol{v}(\tfrac{1}{8},\tfrac{5}{8},\tfrac{1}{8})\cdot\left(\begin{array}{c}0\\0\\1\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{38} = \begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 16 z \left(y - z\right)\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 16 x \left(- x + y\right)\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle - 16 x^{2} - 48 x y - 32 x z + 32 x - 32 y^{2} - 48 y z + 48 y - 16 z^{2} + 32 z - 16\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with volume 0 of the reference element.

\(\displaystyle l_{39}:\boldsymbol{v}\mapsto\boldsymbol{v}(\tfrac{1}{8},\tfrac{1}{8},\tfrac{5}{8})\cdot\left(\begin{array}{c}1\\0\\0\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{39} = \begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 16 y \left(- y + z\right)\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 16 x \left(- x + z\right)\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle - 16 x^{2} - 32 x y - 48 x z + 32 x - 16 y^{2} - 48 y z + 32 y - 32 z^{2} + 48 z - 16\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with volume 0 of the reference element.

\(\displaystyle l_{40}:\boldsymbol{v}\mapsto\boldsymbol{v}(\tfrac{1}{8},\tfrac{1}{8},\tfrac{5}{8})\cdot\left(\begin{array}{c}0\\1\\0\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{40} = \begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 16 y \left(- y + z\right)\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 16 x \left(- x + z\right)\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle - 16 x^{2} - 32 x y - 48 x z + 32 x - 16 y^{2} - 48 y z + 32 y - 32 z^{2} + 48 z - 16\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with volume 0 of the reference element.

\(\displaystyle l_{41}:\boldsymbol{v}\mapsto\boldsymbol{v}(\tfrac{1}{8},\tfrac{1}{8},\tfrac{5}{8})\cdot\left(\begin{array}{c}0\\0\\1\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{41} = \begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 16 y \left(- y + z\right)\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 16 x \left(- x + z\right)\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle - 16 x^{2} - 32 x y - 48 x z + 32 x - 16 y^{2} - 48 y z + 32 y - 32 z^{2} + 48 z - 16\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with volume 0 of the reference element.

\(\displaystyle l_{42}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{0}}\boldsymbol{v}\cdot(1)\hat{\boldsymbol{n}}_{0}\)
where \(f_{0}\) is the 0th face;
and \(\hat{\boldsymbol{n}}_{0}\) is the normal to facet 0.

\(\displaystyle \boldsymbol{\phi}_{42} = \begin{cases} \left(\begin{array}{c}\displaystyle \tfrac{z \left(480 x^{2} + 1440 x y + 1056 x z - 672 x - 2640 y z + 150 y - 3440 z^{2} + 1686 z - 97\right)}{12}\\\displaystyle \tfrac{z \left(1440 x y - 2640 x z + 150 x + 480 y^{2} + 1056 y z - 672 y - 3440 z^{2} + 1686 z - 97\right)}{12}\\\displaystyle \tfrac{z \left(- 1200 x z + 150 x - 1200 y z + 150 y - 704 z^{2} + 864 z - 97\right)}{12}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{y \left(480 x^{2} + 1056 x y + 1440 x z - 672 x - 3440 y^{2} - 2640 y z + 1686 y + 150 z - 97\right)}{12}\\\displaystyle \tfrac{y \left(- 1200 x y + 150 x - 704 y^{2} - 1200 y z + 864 y + 150 z - 97\right)}{12}\\\displaystyle \tfrac{y \left(- 2640 x y + 1440 x z + 150 x - 3440 y^{2} + 1056 y z + 1686 y + 480 z^{2} - 672 z - 97\right)}{12}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{x \left(- 704 x^{2} - 1200 x y - 1200 x z + 864 x + 150 y + 150 z - 97\right)}{12}\\\displaystyle \tfrac{x \left(- 3440 x^{2} + 1056 x y - 2640 x z + 1686 x + 480 y^{2} + 1440 y z - 672 y + 150 z - 97\right)}{12}\\\displaystyle \tfrac{x \left(- 3440 x^{2} - 2640 x y + 1056 x z + 1686 x + 1440 y z + 150 y + 480 z^{2} - 672 z - 97\right)}{12}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{224 x^{3}}{3} + 36 x^{2} y + 36 x^{2} z - 112 x^{2} - 112 x y^{2} - 144 x y z + \tfrac{167 x y}{2} - 112 x z^{2} + \tfrac{167 x z}{2} + \tfrac{289 x}{12} - \tfrac{220 y^{3}}{3} - 180 y^{2} z + \tfrac{311 y^{2}}{2} - 180 y z^{2} + 271 y z - \tfrac{1145 y}{12} - \tfrac{220 z^{3}}{3} + \tfrac{311 z^{2}}{2} - \tfrac{1145 z}{12} + \tfrac{53}{4}\\\displaystyle - \tfrac{220 x^{3}}{3} - 112 x^{2} y - 180 x^{2} z + \tfrac{311 x^{2}}{2} + 36 x y^{2} - 144 x y z + \tfrac{167 x y}{2} - 180 x z^{2} + 271 x z - \tfrac{1145 x}{12} + \tfrac{224 y^{3}}{3} + 36 y^{2} z - 112 y^{2} - 112 y z^{2} + \tfrac{167 y z}{2} + \tfrac{289 y}{12} - \tfrac{220 z^{3}}{3} + \tfrac{311 z^{2}}{2} - \tfrac{1145 z}{12} + \tfrac{53}{4}\\\displaystyle - \tfrac{220 x^{3}}{3} - 180 x^{2} y - 112 x^{2} z + \tfrac{311 x^{2}}{2} - 180 x y^{2} - 144 x y z + 271 x y + 36 x z^{2} + \tfrac{167 x z}{2} - \tfrac{1145 x}{12} - \tfrac{220 y^{3}}{3} - 112 y^{2} z + \tfrac{311 y^{2}}{2} + 36 y z^{2} + \tfrac{167 y z}{2} - \tfrac{1145 y}{12} + \tfrac{224 z^{3}}{3} - 112 z^{2} + \tfrac{289 z}{12} + \tfrac{53}{4}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with face 0 of the reference element.

\(\displaystyle l_{43}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{1}}\boldsymbol{v}\cdot(1)\hat{\boldsymbol{n}}_{1}\)
where \(f_{1}\) is the 1st face;
and \(\hat{\boldsymbol{n}}_{1}\) is the normal to facet 1.

\(\displaystyle \boldsymbol{\phi}_{43} = \begin{cases} \left(\begin{array}{c}\displaystyle \tfrac{z \left(- 720 x y + 1728 x z - 126 x - 480 y^{2} + 840 y z + 345 y - 440 z^{2} - 567 z + 29\right)}{4}\\\displaystyle \tfrac{z \left(- 240 y^{2} - 1752 y z + 399 y + 2600 z^{2} - 627 z + 19\right)}{4}\\\displaystyle \tfrac{z \left(600 y z - 75 y + 8 z^{2} - 153 z + 19\right)}{4}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{y \left(1728 x y - 720 x z - 126 x - 440 y^{2} + 840 y z - 567 y - 480 z^{2} + 345 z + 29\right)}{4}\\\displaystyle \tfrac{y \left(8 y^{2} + 600 y z - 153 y - 75 z + 19\right)}{4}\\\displaystyle \tfrac{y \left(2600 y^{2} - 1752 y z - 627 y - 240 z^{2} + 399 z + 19\right)}{4}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 172 x^{3} + 150 x^{2} y + 150 x^{2} z - \tfrac{279 x^{2}}{2} - \tfrac{135 x y}{4} - \tfrac{135 x z}{4} + \tfrac{29 x}{4} - 120 y^{2} z - 120 y z^{2} + 120 y z\\\displaystyle \tfrac{x \left(1280 x^{2} - 1032 x y + 1320 x z - 552 x - 240 y^{2} - 720 y z + 399 y - 75 z + 19\right)}{4}\\\displaystyle \tfrac{x \left(1280 x^{2} + 1320 x y - 1032 x z - 552 x - 720 y z - 75 y - 240 z^{2} + 399 z + 19\right)}{4}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 812 x^{3} + 2214 x^{2} y + 2214 x^{2} z - \tfrac{4041 x^{2}}{2} + 1932 x y^{2} + 3624 x y z - \tfrac{14139 x y}{4} + 1932 x z^{2} - \tfrac{14139 x z}{4} + \tfrac{6517 x}{4} + 530 y^{3} + 1350 y^{2} z - \tfrac{5817 y^{2}}{4} + 1350 y z^{2} - \tfrac{5337 y z}{2} + 1345 y + 530 z^{3} - \tfrac{5817 z^{2}}{4} + 1345 z - \tfrac{1683}{4}\\\displaystyle - 320 x^{3} - 1218 x^{2} y - 630 x^{2} z + 822 x^{2} - 1416 x y^{2} - 1596 x y z + \tfrac{8241 x y}{4} - 300 x z^{2} + \tfrac{4011 x z}{4} - \tfrac{2755 x}{4} - 518 y^{3} - 906 y^{2} z + \tfrac{4713 y^{2}}{4} - 378 y z^{2} + 1239 y z - 847 y + 10 z^{3} + \tfrac{723 z^{2}}{4} - \tfrac{755 z}{2} + \tfrac{747}{4}\\\displaystyle - 320 x^{3} - 630 x^{2} y - 1218 x^{2} z + 822 x^{2} - 300 x y^{2} - 1596 x y z + \tfrac{4011 x y}{4} - 1416 x z^{2} + \tfrac{8241 x z}{4} - \tfrac{2755 x}{4} + 10 y^{3} - 378 y^{2} z + \tfrac{723 y^{2}}{4} - 906 y z^{2} + 1239 y z - \tfrac{755 y}{2} - 518 z^{3} + \tfrac{4713 z^{2}}{4} - 847 z + \tfrac{747}{4}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with face 1 of the reference element.

\(\displaystyle l_{44}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{2}}\boldsymbol{v}\cdot(1)\hat{\boldsymbol{n}}_{2}\)
where \(f_{2}\) is the 2nd face;
and \(\hat{\boldsymbol{n}}_{2}\) is the normal to facet 2.

\(\displaystyle \boldsymbol{\phi}_{44} = \begin{cases} \left(\begin{array}{c}\displaystyle \tfrac{z \left(240 x^{2} + 1752 x z - 399 x - 2600 z^{2} + 627 z - 19\right)}{4}\\\displaystyle \tfrac{z \left(480 x^{2} + 720 x y - 840 x z - 345 x - 1728 y z + 126 y + 440 z^{2} + 567 z - 29\right)}{4}\\\displaystyle \tfrac{z \left(- 600 x z + 75 x - 8 z^{2} + 153 z - 19\right)}{4}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{y \left(240 x^{2} + 1032 x y + 720 x z - 399 x - 1280 y^{2} - 1320 y z + 552 y + 75 z - 19\right)}{4}\\\displaystyle 120 x^{2} z - 150 x y^{2} + \tfrac{135 x y}{4} + 120 x z^{2} - 120 x z - 172 y^{3} - 150 y^{2} z + \tfrac{279 y^{2}}{2} + \tfrac{135 y z}{4} - \tfrac{29 y}{4}\\\displaystyle \tfrac{y \left(- 1320 x y + 720 x z + 75 x - 1280 y^{2} + 1032 y z + 552 y + 240 z^{2} - 399 z - 19\right)}{4}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{x \left(- 8 x^{2} - 600 x z + 153 x + 75 z - 19\right)}{4}\\\displaystyle \tfrac{x \left(440 x^{2} - 1728 x y - 840 x z + 567 x + 720 y z + 126 y + 480 z^{2} - 345 z - 29\right)}{4}\\\displaystyle \tfrac{x \left(- 2600 x^{2} + 1752 x z + 627 x + 240 z^{2} - 399 z - 19\right)}{4}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 518 x^{3} + 1416 x^{2} y + 906 x^{2} z - \tfrac{4713 x^{2}}{4} + 1218 x y^{2} + 1596 x y z - \tfrac{8241 x y}{4} + 378 x z^{2} - 1239 x z + 847 x + 320 y^{3} + 630 y^{2} z - 822 y^{2} + 300 y z^{2} - \tfrac{4011 y z}{4} + \tfrac{2755 y}{4} - 10 z^{3} - \tfrac{723 z^{2}}{4} + \tfrac{755 z}{2} - \tfrac{747}{4}\\\displaystyle - 530 x^{3} - 1932 x^{2} y - 1350 x^{2} z + \tfrac{5817 x^{2}}{4} - 2214 x y^{2} - 3624 x y z + \tfrac{14139 x y}{4} - 1350 x z^{2} + \tfrac{5337 x z}{2} - 1345 x - 812 y^{3} - 2214 y^{2} z + \tfrac{4041 y^{2}}{2} - 1932 y z^{2} + \tfrac{14139 y z}{4} - \tfrac{6517 y}{4} - 530 z^{3} + \tfrac{5817 z^{2}}{4} - 1345 z + \tfrac{1683}{4}\\\displaystyle - 10 x^{3} + 300 x^{2} y + 378 x^{2} z - \tfrac{723 x^{2}}{4} + 630 x y^{2} + 1596 x y z - \tfrac{4011 x y}{4} + 906 x z^{2} - 1239 x z + \tfrac{755 x}{2} + 320 y^{3} + 1218 y^{2} z - 822 y^{2} + 1416 y z^{2} - \tfrac{8241 y z}{4} + \tfrac{2755 y}{4} + 518 z^{3} - \tfrac{4713 z^{2}}{4} + 847 z - \tfrac{747}{4}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with face 2 of the reference element.

\(\displaystyle l_{45}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{3}}\boldsymbol{v}\cdot(1)\hat{\boldsymbol{n}}_{3}\)
where \(f_{3}\) is the 3rd face;
and \(\hat{\boldsymbol{n}}_{3}\) is the normal to facet 3.

\(\displaystyle \boldsymbol{\phi}_{45} = \begin{cases} \left(\begin{array}{c}\displaystyle \tfrac{z \left(- 240 x^{2} - 720 x y - 1032 x z + 399 x + 1320 y z - 75 y + 1280 z^{2} - 552 z + 19\right)}{4}\\\displaystyle \tfrac{z \left(- 720 x y + 1320 x z - 75 x - 240 y^{2} - 1032 y z + 399 y + 1280 z^{2} - 552 z + 19\right)}{4}\\\displaystyle - 120 x^{2} y - 120 x y^{2} + 120 x y + 150 x z^{2} - \tfrac{135 x z}{4} + 150 y z^{2} - \tfrac{135 y z}{4} + 172 z^{3} - \tfrac{279 z^{2}}{2} + \tfrac{29 z}{4}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{y \left(- 240 x^{2} - 1752 x y + 399 x + 2600 y^{2} - 627 y + 19\right)}{4}\\\displaystyle \tfrac{y \left(600 x y - 75 x + 8 y^{2} - 153 y + 19\right)}{4}\\\displaystyle \tfrac{y \left(- 480 x^{2} + 840 x y - 720 x z + 345 x - 440 y^{2} + 1728 y z - 567 y - 126 z + 29\right)}{4}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle \tfrac{x \left(8 x^{2} + 600 x y - 153 x - 75 y + 19\right)}{4}\\\displaystyle \tfrac{x \left(2600 x^{2} - 1752 x y - 627 x - 240 y^{2} + 399 y + 19\right)}{4}\\\displaystyle \tfrac{x \left(- 440 x^{2} + 840 x y + 1728 x z - 567 x - 480 y^{2} - 720 y z + 345 y - 126 z + 29\right)}{4}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle - 518 x^{3} - 906 x^{2} y - 1416 x^{2} z + \tfrac{4713 x^{2}}{4} - 378 x y^{2} - 1596 x y z + 1239 x y - 1218 x z^{2} + \tfrac{8241 x z}{4} - 847 x + 10 y^{3} - 300 y^{2} z + \tfrac{723 y^{2}}{4} - 630 y z^{2} + \tfrac{4011 y z}{4} - \tfrac{755 y}{2} - 320 z^{3} + 822 z^{2} - \tfrac{2755 z}{4} + \tfrac{747}{4}\\\displaystyle 10 x^{3} - 378 x^{2} y - 300 x^{2} z + \tfrac{723 x^{2}}{4} - 906 x y^{2} - 1596 x y z + 1239 x y - 630 x z^{2} + \tfrac{4011 x z}{4} - \tfrac{755 x}{2} - 518 y^{3} - 1416 y^{2} z + \tfrac{4713 y^{2}}{4} - 1218 y z^{2} + \tfrac{8241 y z}{4} - 847 y - 320 z^{3} + 822 z^{2} - \tfrac{2755 z}{4} + \tfrac{747}{4}\\\displaystyle 530 x^{3} + 1350 x^{2} y + 1932 x^{2} z - \tfrac{5817 x^{2}}{4} + 1350 x y^{2} + 3624 x y z - \tfrac{5337 x y}{2} + 2214 x z^{2} - \tfrac{14139 x z}{4} + 1345 x + 530 y^{3} + 1932 y^{2} z - \tfrac{5817 y^{2}}{4} + 2214 y z^{2} - \tfrac{14139 y z}{4} + 1345 y + 812 z^{3} - \tfrac{4041 z^{2}}{2} + \tfrac{6517 z}{4} - \tfrac{1683}{4}\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with face 3 of the reference element.

\(\displaystyle l_{46}:\boldsymbol{v}\mapsto\boldsymbol{v}(\tfrac{1}{4},\tfrac{1}{4},\tfrac{1}{4})\cdot\left(\begin{array}{c}1\\0\\0\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{46} = \begin{cases} \left(\begin{array}{c}\displaystyle 4 z \left(8 z - 1\right)\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 4 y \left(8 y - 1\right)\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 4 x \left(8 x - 1\right)\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 32 x^{2} + 64 x y + 64 x z - 60 x + 32 y^{2} + 64 y z - 60 y + 32 z^{2} - 60 z + 28\\\displaystyle 0\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with volume 0 of the reference element.

\(\displaystyle l_{47}:\boldsymbol{v}\mapsto\boldsymbol{v}(\tfrac{1}{4},\tfrac{1}{4},\tfrac{1}{4})\cdot\left(\begin{array}{c}0\\1\\0\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{47} = \begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 4 z \left(8 z - 1\right)\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 4 y \left(8 y - 1\right)\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 4 x \left(8 x - 1\right)\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 32 x^{2} + 64 x y + 64 x z - 60 x + 32 y^{2} + 64 y z - 60 y + 32 z^{2} - 60 z + 28\\\displaystyle 0\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with volume 0 of the reference element.

\(\displaystyle l_{48}:\boldsymbol{v}\mapsto\boldsymbol{v}(\tfrac{1}{4},\tfrac{1}{4},\tfrac{1}{4})\cdot\left(\begin{array}{c}0\\0\\1\end{array}\right)\)

\(\displaystyle \boldsymbol{\phi}_{48} = \begin{cases} \left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 4 z \left(8 z - 1\right)\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 1, 0), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 4 y \left(8 y - 1\right)\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (1, 0, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 4 x \left(8 x - 1\right)\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((0, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\\\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 32 x^{2} + 64 x y + 64 x z - 60 x + 32 y^{2} + 64 y z - 60 y + 32 z^{2} - 60 z + 28\end{array}\right)&\text{in }\operatorname{Tetrahedron}(((1, 0, 0), (0, 1, 0), (0, 0, 1), (1/4, 1/4, 1/4)))\end{cases}\)

This DOF is associated with volume 0 of the reference element.