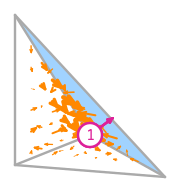
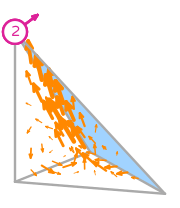
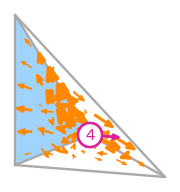
an encyclopedia of finite element definitions
Degree 3 Mardal–Tai–Winther on a tetrahedron
◀ Back to Mardal–Tai–Winther definition page
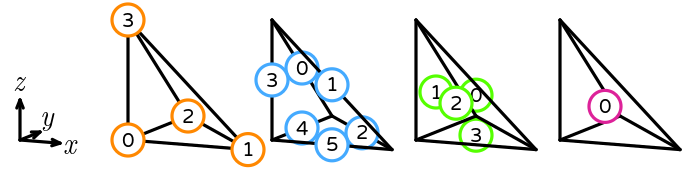
- \(R\) is the reference tetrahedron. The following numbering of the subentities of the reference is used:
- \(\mathcal{V}\) is spanned by: \(\left(\begin{array}{c}\displaystyle 1\\\displaystyle 0\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle 1\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle 1\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle x\\\displaystyle 0\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle x\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle x\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle y\\\displaystyle 0\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle y\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle y\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle z\\\displaystyle 0\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle z\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle 0\\\displaystyle z\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle x y \left(- x - y - 2 z + 1\right)\\\displaystyle x z \left(x + 2 y + z - 1\right)\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle x y \left(x + y + 2 z - 1\right)\\\displaystyle 0\\\displaystyle y z \left(- 2 x - y - z + 1\right)\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle x z \left(- x - 2 y - z + 1\right)\\\displaystyle y z \left(2 x + y + z - 1\right)\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle x^{2} y \left(- x - y - 2 z + 1\right)\\\displaystyle x^{2} z \left(x + 2 y + z - 1\right)\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle x^{2} y \left(x + y + 2 z - 1\right)\\\displaystyle 0\\\displaystyle x y z \left(- 3 x - 2 y - 2 z + 2\right)\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle x^{2} z \left(- x - 2 y - z + 1\right)\\\displaystyle x y z \left(3 x + 2 y + 2 z - 2\right)\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle x y^{2} \left(- x - y - 2 z + 1\right)\\\displaystyle x y z \left(2 x + 3 y + 2 z - 2\right)\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle x y^{2} \left(x + y + 2 z - 1\right)\\\displaystyle 0\\\displaystyle y^{2} z \left(- 2 x - y - z + 1\right)\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle x y z \left(- 2 x - 3 y - 2 z + 2\right)\\\displaystyle y^{2} z \left(2 x + y + z - 1\right)\\\displaystyle 0\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle 0\\\displaystyle x y z \left(- 2 x - 2 y - 3 z + 2\right)\\\displaystyle x z^{2} \left(x + 2 y + z - 1\right)\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle x y z \left(2 x + 2 y + 3 z - 2\right)\\\displaystyle 0\\\displaystyle y z^{2} \left(- 2 x - y - z + 1\right)\end{array}\right)\), \(\left(\begin{array}{c}\displaystyle x z^{2} \left(- x - 2 y - z + 1\right)\\\displaystyle y z^{2} \left(2 x + y + z - 1\right)\\\displaystyle 0\end{array}\right)\)
- \(\mathcal{L}=\{l_0,...,l_{23}\}\)
- Functionals and basis functions:
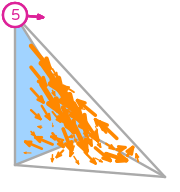
\(\displaystyle l_{0}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{0}}\boldsymbol{v}\cdot(- s_{0} - s_{1} + 1)\hat{\boldsymbol{n}}_{0}\)
where \(f_{0}\) is the 0th face;
\(\hat{\boldsymbol{n}}_{0}\) is the normal to facet 0;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{0}\).
\(\displaystyle \boldsymbol{\phi}_{0} = \left(\begin{array}{c}\displaystyle 6 x \left(- 140 x^{2} y - 140 x^{2} z - 390 x y^{2} - 1600 x y z + 330 x y - 390 x z^{2} + 330 x z - 250 y^{3} - 1800 y^{2} z + 440 y^{2} - 1800 y z^{2} + 1800 y z - 190 y - 250 z^{3} + 440 z^{2} - 190 z + 3\right)\\\displaystyle 6 y \left(- 60 x^{2} y + 540 x^{2} z - 60 x y^{2} + 800 x y z + 60 x y + 960 x z^{2} - 780 x z + 260 y^{2} z + 510 y z^{2} - 450 y z + 250 z^{3} - 440 z^{2} + 190 z - 1\right)\\\displaystyle 6 z \left(540 x^{2} y - 60 x^{2} z + 960 x y^{2} + 800 x y z - 780 x y - 60 x z^{2} + 60 x z + 250 y^{3} + 510 y^{2} z - 440 y^{2} + 260 y z^{2} - 450 y z + 190 y - 1\right)\end{array}\right)\)
This DOF is associated with face 0 of the reference element.
where \(f_{0}\) is the 0th face;
\(\hat{\boldsymbol{n}}_{0}\) is the normal to facet 0;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{0}\).
\(\displaystyle \boldsymbol{\phi}_{0} = \left(\begin{array}{c}\displaystyle 6 x \left(- 140 x^{2} y - 140 x^{2} z - 390 x y^{2} - 1600 x y z + 330 x y - 390 x z^{2} + 330 x z - 250 y^{3} - 1800 y^{2} z + 440 y^{2} - 1800 y z^{2} + 1800 y z - 190 y - 250 z^{3} + 440 z^{2} - 190 z + 3\right)\\\displaystyle 6 y \left(- 60 x^{2} y + 540 x^{2} z - 60 x y^{2} + 800 x y z + 60 x y + 960 x z^{2} - 780 x z + 260 y^{2} z + 510 y z^{2} - 450 y z + 250 z^{3} - 440 z^{2} + 190 z - 1\right)\\\displaystyle 6 z \left(540 x^{2} y - 60 x^{2} z + 960 x y^{2} + 800 x y z - 780 x y - 60 x z^{2} + 60 x z + 250 y^{3} + 510 y^{2} z - 440 y^{2} + 260 y z^{2} - 450 y z + 190 y - 1\right)\end{array}\right)\)
This DOF is associated with face 0 of the reference element.
\(\displaystyle l_{1}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{0}}\boldsymbol{v}\cdot(s_{0})\hat{\boldsymbol{n}}_{0}\)
where \(f_{0}\) is the 0th face;
\(\hat{\boldsymbol{n}}_{0}\) is the normal to facet 0;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{0}\).
\(\displaystyle \boldsymbol{\phi}_{1} = \left(\begin{array}{c}\displaystyle 3 x \left(- 430 x^{2} y + 830 x^{2} z - 585 x y^{2} + 1600 x y z + 585 x y + 1485 x z^{2} - 1365 x z - 155 y^{3} + 1080 y^{2} z + 310 y^{2} + 1920 y z^{2} - 1560 y z - 155 y + 655 z^{3} - 1190 z^{2} + 535 z - 2\right)\\\displaystyle 3 y \left(- 345 x^{3} - 315 x^{2} y - 3600 x^{2} z + 570 x^{2} + 30 x y^{2} - 3200 x y z + 195 x y - 3600 x z^{2} + 3600 x z - 225 x - 590 y^{2} z - 1245 y z^{2} + 1125 y z - 655 z^{3} + 1190 z^{2} - 535 z + 6\right)\\\displaystyle 3 z \left(345 x^{3} + 1920 x^{2} y + 555 x^{2} z - 570 x^{2} + 1080 x y^{2} + 1600 x y z - 1560 x y + 210 x z^{2} - 435 x z + 225 x + 155 y^{3} + 345 y^{2} z - 310 y^{2} + 190 y z^{2} - 345 y z + 155 y - 2\right)\end{array}\right)\)
This DOF is associated with face 0 of the reference element.
where \(f_{0}\) is the 0th face;
\(\hat{\boldsymbol{n}}_{0}\) is the normal to facet 0;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{0}\).
\(\displaystyle \boldsymbol{\phi}_{1} = \left(\begin{array}{c}\displaystyle 3 x \left(- 430 x^{2} y + 830 x^{2} z - 585 x y^{2} + 1600 x y z + 585 x y + 1485 x z^{2} - 1365 x z - 155 y^{3} + 1080 y^{2} z + 310 y^{2} + 1920 y z^{2} - 1560 y z - 155 y + 655 z^{3} - 1190 z^{2} + 535 z - 2\right)\\\displaystyle 3 y \left(- 345 x^{3} - 315 x^{2} y - 3600 x^{2} z + 570 x^{2} + 30 x y^{2} - 3200 x y z + 195 x y - 3600 x z^{2} + 3600 x z - 225 x - 590 y^{2} z - 1245 y z^{2} + 1125 y z - 655 z^{3} + 1190 z^{2} - 535 z + 6\right)\\\displaystyle 3 z \left(345 x^{3} + 1920 x^{2} y + 555 x^{2} z - 570 x^{2} + 1080 x y^{2} + 1600 x y z - 1560 x y + 210 x z^{2} - 435 x z + 225 x + 155 y^{3} + 345 y^{2} z - 310 y^{2} + 190 y z^{2} - 345 y z + 155 y - 2\right)\end{array}\right)\)
This DOF is associated with face 0 of the reference element.
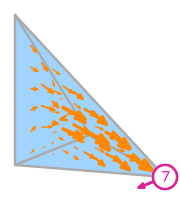
\(\displaystyle l_{2}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{0}}\boldsymbol{v}\cdot(s_{1})\hat{\boldsymbol{n}}_{0}\)
where \(f_{0}\) is the 0th face;
\(\hat{\boldsymbol{n}}_{0}\) is the normal to facet 0;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{0}\).
\(\displaystyle \boldsymbol{\phi}_{2} = \left(\begin{array}{c}\displaystyle 3 x \left(830 x^{2} y - 430 x^{2} z + 1485 x y^{2} + 1600 x y z - 1365 x y - 585 x z^{2} + 585 x z + 655 y^{3} + 1920 y^{2} z - 1190 y^{2} + 1080 y z^{2} - 1560 y z + 535 y - 155 z^{3} + 310 z^{2} - 155 z - 2\right)\\\displaystyle 3 y \left(345 x^{3} + 555 x^{2} y + 1920 x^{2} z - 570 x^{2} + 210 x y^{2} + 1600 x y z - 435 x y + 1080 x z^{2} - 1560 x z + 225 x + 190 y^{2} z + 345 y z^{2} - 345 y z + 155 z^{3} - 310 z^{2} + 155 z - 2\right)\\\displaystyle 3 z \left(- 345 x^{3} - 3600 x^{2} y - 315 x^{2} z + 570 x^{2} - 3600 x y^{2} - 3200 x y z + 3600 x y + 30 x z^{2} + 195 x z - 225 x - 655 y^{3} - 1245 y^{2} z + 1190 y^{2} - 590 y z^{2} + 1125 y z - 535 y + 6\right)\end{array}\right)\)
This DOF is associated with face 0 of the reference element.
where \(f_{0}\) is the 0th face;
\(\hat{\boldsymbol{n}}_{0}\) is the normal to facet 0;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{0}\).
\(\displaystyle \boldsymbol{\phi}_{2} = \left(\begin{array}{c}\displaystyle 3 x \left(830 x^{2} y - 430 x^{2} z + 1485 x y^{2} + 1600 x y z - 1365 x y - 585 x z^{2} + 585 x z + 655 y^{3} + 1920 y^{2} z - 1190 y^{2} + 1080 y z^{2} - 1560 y z + 535 y - 155 z^{3} + 310 z^{2} - 155 z - 2\right)\\\displaystyle 3 y \left(345 x^{3} + 555 x^{2} y + 1920 x^{2} z - 570 x^{2} + 210 x y^{2} + 1600 x y z - 435 x y + 1080 x z^{2} - 1560 x z + 225 x + 190 y^{2} z + 345 y z^{2} - 345 y z + 155 z^{3} - 310 z^{2} + 155 z - 2\right)\\\displaystyle 3 z \left(- 345 x^{3} - 3600 x^{2} y - 315 x^{2} z + 570 x^{2} - 3600 x y^{2} - 3200 x y z + 3600 x y + 30 x z^{2} + 195 x z - 225 x - 655 y^{3} - 1245 y^{2} z + 1190 y^{2} - 590 y z^{2} + 1125 y z - 535 y + 6\right)\end{array}\right)\)
This DOF is associated with face 0 of the reference element.
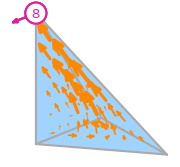
\(\displaystyle l_{3}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{1}}\boldsymbol{v}\cdot(- s_{0} - s_{1} + 1)\hat{\boldsymbol{n}}_{1}\)
where \(f_{1}\) is the 1st face;
\(\hat{\boldsymbol{n}}_{1}\) is the normal to facet 1;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{1}\).
\(\displaystyle \boldsymbol{\phi}_{3} = \left(\begin{array}{c}\displaystyle 720 x^{3} y + 720 x^{3} z - 360 x^{2} y^{2} + 1440 x^{2} y z - 360 x^{2} y - 360 x^{2} z^{2} - 360 x^{2} z - 1080 x y^{3} - 3960 x y^{2} z + 1440 x y^{2} - 3960 x y z^{2} + 2880 x y z - 360 x y - 1080 x z^{3} + 1440 x z^{2} - 360 x z - 18 x - 24 y - 24 z + 18\\\displaystyle 6 y \left(60 x^{2} y - 480 x^{2} z + 60 x y^{2} - 120 x y z - 60 x y - 60 x z^{2} + 240 x z + 60 y^{2} z + 240 y z^{2} - 120 y z + 180 z^{3} - 240 z^{2} + 60 z + 1\right)\\\displaystyle 6 z \left(- 480 x^{2} y + 60 x^{2} z - 60 x y^{2} - 120 x y z + 240 x y + 60 x z^{2} - 60 x z + 180 y^{3} + 240 y^{2} z - 240 y^{2} + 60 y z^{2} - 120 y z + 60 y + 1\right)\end{array}\right)\)
This DOF is associated with face 1 of the reference element.
where \(f_{1}\) is the 1st face;
\(\hat{\boldsymbol{n}}_{1}\) is the normal to facet 1;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{1}\).
\(\displaystyle \boldsymbol{\phi}_{3} = \left(\begin{array}{c}\displaystyle 720 x^{3} y + 720 x^{3} z - 360 x^{2} y^{2} + 1440 x^{2} y z - 360 x^{2} y - 360 x^{2} z^{2} - 360 x^{2} z - 1080 x y^{3} - 3960 x y^{2} z + 1440 x y^{2} - 3960 x y z^{2} + 2880 x y z - 360 x y - 1080 x z^{3} + 1440 x z^{2} - 360 x z - 18 x - 24 y - 24 z + 18\\\displaystyle 6 y \left(60 x^{2} y - 480 x^{2} z + 60 x y^{2} - 120 x y z - 60 x y - 60 x z^{2} + 240 x z + 60 y^{2} z + 240 y z^{2} - 120 y z + 180 z^{3} - 240 z^{2} + 60 z + 1\right)\\\displaystyle 6 z \left(- 480 x^{2} y + 60 x^{2} z - 60 x y^{2} - 120 x y z + 240 x y + 60 x z^{2} - 60 x z + 180 y^{3} + 240 y^{2} z - 240 y^{2} + 60 y z^{2} - 120 y z + 60 y + 1\right)\end{array}\right)\)
This DOF is associated with face 1 of the reference element.
\(\displaystyle l_{4}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{1}}\boldsymbol{v}\cdot(s_{0})\hat{\boldsymbol{n}}_{1}\)
where \(f_{1}\) is the 1st face;
\(\hat{\boldsymbol{n}}_{1}\) is the normal to facet 1;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{1}\).
\(\displaystyle \boldsymbol{\phi}_{4} = \left(\begin{array}{c}\displaystyle 3330 x^{3} y - 4770 x^{3} z + 5715 x^{2} y^{2} - 4320 x^{2} y z - 4995 x^{2} y - 6255 x^{2} z^{2} + 6615 x^{2} z + 2385 x y^{3} + 360 x y^{2} z - 4050 x y^{2} - 2160 x y z^{2} + 1800 x y z + 1665 x y - 1485 x z^{3} + 3330 x z^{2} - 1845 x z + 6 x + 24 y - 6\\\displaystyle 9 y \left(- 185 x^{3} - 435 x^{2} y + 880 x^{2} z + 290 x^{2} - 250 x y^{2} + 880 x y z + 355 x y + 880 x z^{2} - 920 x z - 105 x + 330 y^{2} z + 495 y z^{2} - 535 y z + 165 z^{3} - 370 z^{2} + 205 z - 2\right)\\\displaystyle 3 z \left(555 x^{3} - 720 x^{2} y + 1065 x^{2} z - 870 x^{2} - 1560 x y^{2} - 1200 x y z + 1200 x y + 510 x z^{2} - 825 x z + 315 x - 795 y^{3} - 1545 y^{2} z + 1350 y^{2} - 750 y z^{2} + 1305 y z - 555 y + 2\right)\end{array}\right)\)
This DOF is associated with face 1 of the reference element.
where \(f_{1}\) is the 1st face;
\(\hat{\boldsymbol{n}}_{1}\) is the normal to facet 1;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{1}\).
\(\displaystyle \boldsymbol{\phi}_{4} = \left(\begin{array}{c}\displaystyle 3330 x^{3} y - 4770 x^{3} z + 5715 x^{2} y^{2} - 4320 x^{2} y z - 4995 x^{2} y - 6255 x^{2} z^{2} + 6615 x^{2} z + 2385 x y^{3} + 360 x y^{2} z - 4050 x y^{2} - 2160 x y z^{2} + 1800 x y z + 1665 x y - 1485 x z^{3} + 3330 x z^{2} - 1845 x z + 6 x + 24 y - 6\\\displaystyle 9 y \left(- 185 x^{3} - 435 x^{2} y + 880 x^{2} z + 290 x^{2} - 250 x y^{2} + 880 x y z + 355 x y + 880 x z^{2} - 920 x z - 105 x + 330 y^{2} z + 495 y z^{2} - 535 y z + 165 z^{3} - 370 z^{2} + 205 z - 2\right)\\\displaystyle 3 z \left(555 x^{3} - 720 x^{2} y + 1065 x^{2} z - 870 x^{2} - 1560 x y^{2} - 1200 x y z + 1200 x y + 510 x z^{2} - 825 x z + 315 x - 795 y^{3} - 1545 y^{2} z + 1350 y^{2} - 750 y z^{2} + 1305 y z - 555 y + 2\right)\end{array}\right)\)
This DOF is associated with face 1 of the reference element.
\(\displaystyle l_{5}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{1}}\boldsymbol{v}\cdot(s_{1})\hat{\boldsymbol{n}}_{1}\)
where \(f_{1}\) is the 1st face;
\(\hat{\boldsymbol{n}}_{1}\) is the normal to facet 1;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{1}\).
\(\displaystyle \boldsymbol{\phi}_{5} = \left(\begin{array}{c}\displaystyle - 4770 x^{3} y + 3330 x^{3} z - 6255 x^{2} y^{2} - 4320 x^{2} y z + 6615 x^{2} y + 5715 x^{2} z^{2} - 4995 x^{2} z - 1485 x y^{3} - 2160 x y^{2} z + 3330 x y^{2} + 360 x y z^{2} + 1800 x y z - 1845 x y + 2385 x z^{3} - 4050 x z^{2} + 1665 x z + 6 x + 24 z - 6\\\displaystyle 3 y \left(555 x^{3} + 1065 x^{2} y - 720 x^{2} z - 870 x^{2} + 510 x y^{2} - 1200 x y z - 825 x y - 1560 x z^{2} + 1200 x z + 315 x - 750 y^{2} z - 1545 y z^{2} + 1305 y z - 795 z^{3} + 1350 z^{2} - 555 z + 2\right)\\\displaystyle 9 z \left(- 185 x^{3} + 880 x^{2} y - 435 x^{2} z + 290 x^{2} + 880 x y^{2} + 880 x y z - 920 x y - 250 x z^{2} + 355 x z - 105 x + 165 y^{3} + 495 y^{2} z - 370 y^{2} + 330 y z^{2} - 535 y z + 205 y - 2\right)\end{array}\right)\)
This DOF is associated with face 1 of the reference element.
where \(f_{1}\) is the 1st face;
\(\hat{\boldsymbol{n}}_{1}\) is the normal to facet 1;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{1}\).
\(\displaystyle \boldsymbol{\phi}_{5} = \left(\begin{array}{c}\displaystyle - 4770 x^{3} y + 3330 x^{3} z - 6255 x^{2} y^{2} - 4320 x^{2} y z + 6615 x^{2} y + 5715 x^{2} z^{2} - 4995 x^{2} z - 1485 x y^{3} - 2160 x y^{2} z + 3330 x y^{2} + 360 x y z^{2} + 1800 x y z - 1845 x y + 2385 x z^{3} - 4050 x z^{2} + 1665 x z + 6 x + 24 z - 6\\\displaystyle 3 y \left(555 x^{3} + 1065 x^{2} y - 720 x^{2} z - 870 x^{2} + 510 x y^{2} - 1200 x y z - 825 x y - 1560 x z^{2} + 1200 x z + 315 x - 750 y^{2} z - 1545 y z^{2} + 1305 y z - 795 z^{3} + 1350 z^{2} - 555 z + 2\right)\\\displaystyle 9 z \left(- 185 x^{3} + 880 x^{2} y - 435 x^{2} z + 290 x^{2} + 880 x y^{2} + 880 x y z - 920 x y - 250 x z^{2} + 355 x z - 105 x + 165 y^{3} + 495 y^{2} z - 370 y^{2} + 330 y z^{2} - 535 y z + 205 y - 2\right)\end{array}\right)\)
This DOF is associated with face 1 of the reference element.
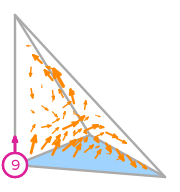
\(\displaystyle l_{6}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{2}}\boldsymbol{v}\cdot(- s_{0} - s_{1} + 1)\hat{\boldsymbol{n}}_{2}\)
where \(f_{2}\) is the 2nd face;
\(\hat{\boldsymbol{n}}_{2}\) is the normal to facet 2;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{2}\).
\(\displaystyle \boldsymbol{\phi}_{6} = \left(\begin{array}{c}\displaystyle 6 x \left(60 x^{2} y - 180 x^{2} z + 120 x y^{2} + 120 x y z - 120 x y - 420 x z^{2} + 300 x z + 60 y^{3} + 480 y^{2} z - 120 y^{2} + 60 y z^{2} - 240 y z + 60 y - 240 z^{3} + 360 z^{2} - 120 z - 1\right)\\\displaystyle 720 x^{3} y - 720 x^{2} y^{2} + 3960 x^{2} y z - 720 x^{2} y - 1440 x y^{3} - 1440 x y^{2} z + 1440 x y^{2} + 3960 x y z^{2} - 2880 x y z + 24 x + 1440 y^{2} z^{2} - 720 y^{2} z + 1440 y z^{3} - 2160 y z^{2} + 720 y z + 18 y + 24 z - 18\\\displaystyle 6 z \left(- 120 x^{3} + 60 x^{2} y - 60 x^{2} z + 120 x^{2} + 480 x y^{2} + 120 x y z - 240 x y + 60 x z^{2} - 60 x z - 60 y^{3} - 240 y^{2} z + 120 y^{2} - 180 y z^{2} + 240 y z - 60 y - 1\right)\end{array}\right)\)
This DOF is associated with face 2 of the reference element.
where \(f_{2}\) is the 2nd face;
\(\hat{\boldsymbol{n}}_{2}\) is the normal to facet 2;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{2}\).
\(\displaystyle \boldsymbol{\phi}_{6} = \left(\begin{array}{c}\displaystyle 6 x \left(60 x^{2} y - 180 x^{2} z + 120 x y^{2} + 120 x y z - 120 x y - 420 x z^{2} + 300 x z + 60 y^{3} + 480 y^{2} z - 120 y^{2} + 60 y z^{2} - 240 y z + 60 y - 240 z^{3} + 360 z^{2} - 120 z - 1\right)\\\displaystyle 720 x^{3} y - 720 x^{2} y^{2} + 3960 x^{2} y z - 720 x^{2} y - 1440 x y^{3} - 1440 x y^{2} z + 1440 x y^{2} + 3960 x y z^{2} - 2880 x y z + 24 x + 1440 y^{2} z^{2} - 720 y^{2} z + 1440 y z^{3} - 2160 y z^{2} + 720 y z + 18 y + 24 z - 18\\\displaystyle 6 z \left(- 120 x^{3} + 60 x^{2} y - 60 x^{2} z + 120 x^{2} + 480 x y^{2} + 120 x y z - 240 x y + 60 x z^{2} - 60 x z - 60 y^{3} - 240 y^{2} z + 120 y^{2} - 180 y z^{2} + 240 y z - 60 y - 1\right)\end{array}\right)\)
This DOF is associated with face 2 of the reference element.
\(\displaystyle l_{7}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{2}}\boldsymbol{v}\cdot(s_{0})\hat{\boldsymbol{n}}_{2}\)
where \(f_{2}\) is the 2nd face;
\(\hat{\boldsymbol{n}}_{2}\) is the normal to facet 2;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{2}\).
\(\displaystyle \boldsymbol{\phi}_{7} = \left(\begin{array}{c}\displaystyle 9 x \left(10 x^{2} y - 90 x^{2} z + 75 x y^{2} - 880 x y z + 5 x y - 135 x z^{2} + 175 x z + 65 y^{3} - 880 y^{2} z - 50 y^{2} - 880 y z^{2} + 920 y z - 15 y - 45 z^{3} + 130 z^{2} - 85 z + 2\right)\\\displaystyle - 1305 x^{3} y - 2475 x^{2} y^{2} - 360 x^{2} y z + 1890 x^{2} y - 1170 x y^{3} + 4320 x y^{2} z + 1755 x y^{2} + 2160 x y z^{2} - 1800 x y z - 585 x y - 24 x + 2610 y^{3} z + 3015 y^{2} z^{2} - 3375 y^{2} z + 405 y z^{3} - 1170 y z^{2} + 765 y z - 6 y + 6\\\displaystyle 3 z \left(435 x^{3} + 1560 x^{2} y + 465 x^{2} z - 630 x^{2} + 720 x y^{2} + 1200 x y z - 1200 x y + 30 x z^{2} - 225 x z + 195 x - 195 y^{3} + 15 y^{2} z + 150 y^{2} + 210 y z^{2} - 255 y z + 45 y - 2\right)\end{array}\right)\)
This DOF is associated with face 2 of the reference element.
where \(f_{2}\) is the 2nd face;
\(\hat{\boldsymbol{n}}_{2}\) is the normal to facet 2;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{2}\).
\(\displaystyle \boldsymbol{\phi}_{7} = \left(\begin{array}{c}\displaystyle 9 x \left(10 x^{2} y - 90 x^{2} z + 75 x y^{2} - 880 x y z + 5 x y - 135 x z^{2} + 175 x z + 65 y^{3} - 880 y^{2} z - 50 y^{2} - 880 y z^{2} + 920 y z - 15 y - 45 z^{3} + 130 z^{2} - 85 z + 2\right)\\\displaystyle - 1305 x^{3} y - 2475 x^{2} y^{2} - 360 x^{2} y z + 1890 x^{2} y - 1170 x y^{3} + 4320 x y^{2} z + 1755 x y^{2} + 2160 x y z^{2} - 1800 x y z - 585 x y - 24 x + 2610 y^{3} z + 3015 y^{2} z^{2} - 3375 y^{2} z + 405 y z^{3} - 1170 y z^{2} + 765 y z - 6 y + 6\\\displaystyle 3 z \left(435 x^{3} + 1560 x^{2} y + 465 x^{2} z - 630 x^{2} + 720 x y^{2} + 1200 x y z - 1200 x y + 30 x z^{2} - 225 x z + 195 x - 195 y^{3} + 15 y^{2} z + 150 y^{2} + 210 y z^{2} - 255 y z + 45 y - 2\right)\end{array}\right)\)
This DOF is associated with face 2 of the reference element.
\(\displaystyle l_{8}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{2}}\boldsymbol{v}\cdot(s_{1})\hat{\boldsymbol{n}}_{2}\)
where \(f_{2}\) is the 2nd face;
\(\hat{\boldsymbol{n}}_{2}\) is the normal to facet 2;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{2}\).
\(\displaystyle \boldsymbol{\phi}_{8} = \left(\begin{array}{c}\displaystyle 6 x \left(- 30 x^{2} y + 150 x^{2} z - 195 x y^{2} + 600 x y z + 75 x y + 435 x z^{2} - 315 x z - 165 y^{3} + 360 y^{2} z + 210 y^{2} + 780 y z^{2} - 600 y z - 45 y + 285 z^{3} - 450 z^{2} + 165 z - 1\right)\\\displaystyle 810 x^{3} y + 4230 x^{2} y^{2} + 2160 x^{2} y z - 1980 x^{2} y + 3420 x y^{3} + 4320 x y^{2} z - 4590 x y^{2} - 360 x y z^{2} - 1800 x y z + 1170 x y - 1980 y^{3} z - 3690 y^{2} z^{2} + 2970 y^{2} z - 1710 y z^{3} + 2700 y z^{2} - 990 y z - 6 y - 24 z + 6\\\displaystyle 18 z \left(- 45 x^{3} - 440 x^{2} y - 135 x^{2} z + 110 x^{2} - 440 x y^{2} - 440 x y z + 460 x y - 90 x z^{2} + 155 x z - 65 x + 55 y^{3} + 105 y^{2} z - 70 y^{2} + 50 y z^{2} - 65 y z + 15 y + 1\right)\end{array}\right)\)
This DOF is associated with face 2 of the reference element.
where \(f_{2}\) is the 2nd face;
\(\hat{\boldsymbol{n}}_{2}\) is the normal to facet 2;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{2}\).
\(\displaystyle \boldsymbol{\phi}_{8} = \left(\begin{array}{c}\displaystyle 6 x \left(- 30 x^{2} y + 150 x^{2} z - 195 x y^{2} + 600 x y z + 75 x y + 435 x z^{2} - 315 x z - 165 y^{3} + 360 y^{2} z + 210 y^{2} + 780 y z^{2} - 600 y z - 45 y + 285 z^{3} - 450 z^{2} + 165 z - 1\right)\\\displaystyle 810 x^{3} y + 4230 x^{2} y^{2} + 2160 x^{2} y z - 1980 x^{2} y + 3420 x y^{3} + 4320 x y^{2} z - 4590 x y^{2} - 360 x y z^{2} - 1800 x y z + 1170 x y - 1980 y^{3} z - 3690 y^{2} z^{2} + 2970 y^{2} z - 1710 y z^{3} + 2700 y z^{2} - 990 y z - 6 y - 24 z + 6\\\displaystyle 18 z \left(- 45 x^{3} - 440 x^{2} y - 135 x^{2} z + 110 x^{2} - 440 x y^{2} - 440 x y z + 460 x y - 90 x z^{2} + 155 x z - 65 x + 55 y^{3} + 105 y^{2} z - 70 y^{2} + 50 y z^{2} - 65 y z + 15 y + 1\right)\end{array}\right)\)
This DOF is associated with face 2 of the reference element.
\(\displaystyle l_{9}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{3}}\boldsymbol{v}\cdot(- s_{0} - s_{1} + 1)\hat{\boldsymbol{n}}_{3}\)
where \(f_{3}\) is the 3rd face;
\(\hat{\boldsymbol{n}}_{3}\) is the normal to facet 3;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{3}\).
\(\displaystyle \boldsymbol{\phi}_{9} = \left(\begin{array}{c}\displaystyle 6 x \left(180 x^{2} y - 60 x^{2} z + 420 x y^{2} - 120 x y z - 300 x y - 120 x z^{2} + 120 x z + 240 y^{3} - 60 y^{2} z - 360 y^{2} - 480 y z^{2} + 240 y z + 120 y - 60 z^{3} + 120 z^{2} - 60 z + 1\right)\\\displaystyle 6 y \left(120 x^{3} + 60 x^{2} y - 60 x^{2} z - 120 x^{2} - 60 x y^{2} - 120 x y z + 60 x y - 480 x z^{2} + 240 x z + 180 y^{2} z + 240 y z^{2} - 240 y z + 60 z^{3} - 120 z^{2} + 60 z + 1\right)\\\displaystyle - 720 x^{3} z - 3960 x^{2} y z + 720 x^{2} z^{2} + 720 x^{2} z - 3960 x y^{2} z + 1440 x y z^{2} + 2880 x y z + 1440 x z^{3} - 1440 x z^{2} - 24 x - 1440 y^{3} z - 1440 y^{2} z^{2} + 2160 y^{2} z + 720 y z^{2} - 720 y z - 24 y - 18 z + 18\end{array}\right)\)
This DOF is associated with face 3 of the reference element.
where \(f_{3}\) is the 3rd face;
\(\hat{\boldsymbol{n}}_{3}\) is the normal to facet 3;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{3}\).
\(\displaystyle \boldsymbol{\phi}_{9} = \left(\begin{array}{c}\displaystyle 6 x \left(180 x^{2} y - 60 x^{2} z + 420 x y^{2} - 120 x y z - 300 x y - 120 x z^{2} + 120 x z + 240 y^{3} - 60 y^{2} z - 360 y^{2} - 480 y z^{2} + 240 y z + 120 y - 60 z^{3} + 120 z^{2} - 60 z + 1\right)\\\displaystyle 6 y \left(120 x^{3} + 60 x^{2} y - 60 x^{2} z - 120 x^{2} - 60 x y^{2} - 120 x y z + 60 x y - 480 x z^{2} + 240 x z + 180 y^{2} z + 240 y z^{2} - 240 y z + 60 z^{3} - 120 z^{2} + 60 z + 1\right)\\\displaystyle - 720 x^{3} z - 3960 x^{2} y z + 720 x^{2} z^{2} + 720 x^{2} z - 3960 x y^{2} z + 1440 x y z^{2} + 2880 x y z + 1440 x z^{3} - 1440 x z^{2} - 24 x - 1440 y^{3} z - 1440 y^{2} z^{2} + 2160 y^{2} z + 720 y z^{2} - 720 y z - 24 y - 18 z + 18\end{array}\right)\)
This DOF is associated with face 3 of the reference element.
\(\displaystyle l_{10}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{3}}\boldsymbol{v}\cdot(s_{0})\hat{\boldsymbol{n}}_{3}\)
where \(f_{3}\) is the 3rd face;
\(\hat{\boldsymbol{n}}_{3}\) is the normal to facet 3;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{3}\).
\(\displaystyle \boldsymbol{\phi}_{10} = \left(\begin{array}{c}\displaystyle 9 x \left(90 x^{2} y - 10 x^{2} z + 135 x y^{2} + 880 x y z - 175 x y - 75 x z^{2} - 5 x z + 45 y^{3} + 880 y^{2} z - 130 y^{2} + 880 y z^{2} - 920 y z + 85 y - 65 z^{3} + 50 z^{2} + 15 z - 2\right)\\\displaystyle 3 y \left(- 435 x^{3} - 465 x^{2} y - 1560 x^{2} z + 630 x^{2} - 30 x y^{2} - 1200 x y z + 225 x y - 720 x z^{2} + 1200 x z - 195 x - 210 y^{2} z - 15 y z^{2} + 255 y z + 195 z^{3} - 150 z^{2} - 45 z + 2\right)\\\displaystyle 1305 x^{3} z + 360 x^{2} y z + 2475 x^{2} z^{2} - 1890 x^{2} z - 2160 x y^{2} z - 4320 x y z^{2} + 1800 x y z + 1170 x z^{3} - 1755 x z^{2} + 585 x z + 24 x - 405 y^{3} z - 3015 y^{2} z^{2} + 1170 y^{2} z - 2610 y z^{3} + 3375 y z^{2} - 765 y z + 6 z - 6\end{array}\right)\)
This DOF is associated with face 3 of the reference element.
where \(f_{3}\) is the 3rd face;
\(\hat{\boldsymbol{n}}_{3}\) is the normal to facet 3;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{3}\).
\(\displaystyle \boldsymbol{\phi}_{10} = \left(\begin{array}{c}\displaystyle 9 x \left(90 x^{2} y - 10 x^{2} z + 135 x y^{2} + 880 x y z - 175 x y - 75 x z^{2} - 5 x z + 45 y^{3} + 880 y^{2} z - 130 y^{2} + 880 y z^{2} - 920 y z + 85 y - 65 z^{3} + 50 z^{2} + 15 z - 2\right)\\\displaystyle 3 y \left(- 435 x^{3} - 465 x^{2} y - 1560 x^{2} z + 630 x^{2} - 30 x y^{2} - 1200 x y z + 225 x y - 720 x z^{2} + 1200 x z - 195 x - 210 y^{2} z - 15 y z^{2} + 255 y z + 195 z^{3} - 150 z^{2} - 45 z + 2\right)\\\displaystyle 1305 x^{3} z + 360 x^{2} y z + 2475 x^{2} z^{2} - 1890 x^{2} z - 2160 x y^{2} z - 4320 x y z^{2} + 1800 x y z + 1170 x z^{3} - 1755 x z^{2} + 585 x z + 24 x - 405 y^{3} z - 3015 y^{2} z^{2} + 1170 y^{2} z - 2610 y z^{3} + 3375 y z^{2} - 765 y z + 6 z - 6\end{array}\right)\)
This DOF is associated with face 3 of the reference element.
\(\displaystyle l_{11}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{3}}\boldsymbol{v}\cdot(s_{1})\hat{\boldsymbol{n}}_{3}\)
where \(f_{3}\) is the 3rd face;
\(\hat{\boldsymbol{n}}_{3}\) is the normal to facet 3;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{3}\).
\(\displaystyle \boldsymbol{\phi}_{11} = \left(\begin{array}{c}\displaystyle 6 x \left(- 150 x^{2} y + 30 x^{2} z - 435 x y^{2} - 600 x y z + 315 x y + 195 x z^{2} - 75 x z - 285 y^{3} - 780 y^{2} z + 450 y^{2} - 360 y z^{2} + 600 y z - 165 y + 165 z^{3} - 210 z^{2} + 45 z + 1\right)\\\displaystyle 18 y \left(45 x^{3} + 135 x^{2} y + 440 x^{2} z - 110 x^{2} + 90 x y^{2} + 440 x y z - 155 x y + 440 x z^{2} - 460 x z + 65 x - 50 y^{2} z - 105 y z^{2} + 65 y z - 55 z^{3} + 70 z^{2} - 15 z - 1\right)\\\displaystyle - 810 x^{3} z - 2160 x^{2} y z - 4230 x^{2} z^{2} + 1980 x^{2} z + 360 x y^{2} z - 4320 x y z^{2} + 1800 x y z - 3420 x z^{3} + 4590 x z^{2} - 1170 x z + 1710 y^{3} z + 3690 y^{2} z^{2} - 2700 y^{2} z + 1980 y z^{3} - 2970 y z^{2} + 990 y z + 24 y + 6 z - 6\end{array}\right)\)
This DOF is associated with face 3 of the reference element.
where \(f_{3}\) is the 3rd face;
\(\hat{\boldsymbol{n}}_{3}\) is the normal to facet 3;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{3}\).
\(\displaystyle \boldsymbol{\phi}_{11} = \left(\begin{array}{c}\displaystyle 6 x \left(- 150 x^{2} y + 30 x^{2} z - 435 x y^{2} - 600 x y z + 315 x y + 195 x z^{2} - 75 x z - 285 y^{3} - 780 y^{2} z + 450 y^{2} - 360 y z^{2} + 600 y z - 165 y + 165 z^{3} - 210 z^{2} + 45 z + 1\right)\\\displaystyle 18 y \left(45 x^{3} + 135 x^{2} y + 440 x^{2} z - 110 x^{2} + 90 x y^{2} + 440 x y z - 155 x y + 440 x z^{2} - 460 x z + 65 x - 50 y^{2} z - 105 y z^{2} + 65 y z - 55 z^{3} + 70 z^{2} - 15 z - 1\right)\\\displaystyle - 810 x^{3} z - 2160 x^{2} y z - 4230 x^{2} z^{2} + 1980 x^{2} z + 360 x y^{2} z - 4320 x y z^{2} + 1800 x y z - 3420 x z^{3} + 4590 x z^{2} - 1170 x z + 1710 y^{3} z + 3690 y^{2} z^{2} - 2700 y^{2} z + 1980 y z^{3} - 2970 y z^{2} + 990 y z + 24 y + 6 z - 6\end{array}\right)\)
This DOF is associated with face 3 of the reference element.
\(\displaystyle l_{12}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{0}}\boldsymbol{v}\cdot\left(\begin{array}{c}\frac{\sqrt{3} \left(- s_{0} + s_{1}\right)}{3}\\- \frac{\sqrt{3} s_{1}}{3}\\\frac{\sqrt{3} s_{0}}{3}\end{array}\right)\)
where \(f_{0}\) is the 0th face;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{0}\).
\(\displaystyle \boldsymbol{\phi}_{12} = \left(\begin{array}{c}\displaystyle 180 x \left(2 x^{2} y - 2 x^{2} z + 3 x y^{2} - 3 x y - 3 x z^{2} + 3 x z + y^{3} - 2 y^{2} + y - z^{3} + 2 z^{2} - z\right)\\\displaystyle 180 y \left(- 2 x^{3} - 6 x^{2} y - 6 x^{2} z + 4 x^{2} - 4 x y^{2} - 8 x y z + 6 x y - 6 x z^{2} + 6 x z - 2 x + 2 y^{2} z + 3 y z^{2} - 3 y z + z^{3} - 2 z^{2} + z\right)\\\displaystyle 180 z \left(2 x^{3} + 6 x^{2} y + 6 x^{2} z - 4 x^{2} + 6 x y^{2} + 8 x y z - 6 x y + 4 x z^{2} - 6 x z + 2 x - y^{3} - 3 y^{2} z + 2 y^{2} - 2 y z^{2} + 3 y z - y\right)\end{array}\right)\)
This DOF is associated with face 0 of the reference element.
where \(f_{0}\) is the 0th face;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{0}\).
\(\displaystyle \boldsymbol{\phi}_{12} = \left(\begin{array}{c}\displaystyle 180 x \left(2 x^{2} y - 2 x^{2} z + 3 x y^{2} - 3 x y - 3 x z^{2} + 3 x z + y^{3} - 2 y^{2} + y - z^{3} + 2 z^{2} - z\right)\\\displaystyle 180 y \left(- 2 x^{3} - 6 x^{2} y - 6 x^{2} z + 4 x^{2} - 4 x y^{2} - 8 x y z + 6 x y - 6 x z^{2} + 6 x z - 2 x + 2 y^{2} z + 3 y z^{2} - 3 y z + z^{3} - 2 z^{2} + z\right)\\\displaystyle 180 z \left(2 x^{3} + 6 x^{2} y + 6 x^{2} z - 4 x^{2} + 6 x y^{2} + 8 x y z - 6 x y + 4 x z^{2} - 6 x z + 2 x - y^{3} - 3 y^{2} z + 2 y^{2} - 2 y z^{2} + 3 y z - y\right)\end{array}\right)\)
This DOF is associated with face 0 of the reference element.
\(\displaystyle l_{13}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{0}}\boldsymbol{v}\cdot\left(\begin{array}{c}\frac{\sqrt{3} \left(s_{0} - s_{1} - 1\right)}{3}\\\frac{\sqrt{3} s_{1}}{3}\\\frac{\sqrt{3} \left(1 - s_{0}\right)}{3}\end{array}\right)\)
where \(f_{0}\) is the 0th face;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{0}\).
\(\displaystyle \boldsymbol{\phi}_{13} = \left(\begin{array}{c}\displaystyle 15 x \left(- 34 x^{2} y + 26 x^{2} z - 51 x y^{2} - 32 x y z + 51 x y + 39 x z^{2} - 39 x z - 17 y^{3} - 24 y^{2} z + 34 y^{2} - 24 y z^{2} + 24 y z - 17 y + 13 z^{3} - 26 z^{2} + 13 z\right)\\\displaystyle 15 y \left(9 x^{3} + 27 x^{2} y - 24 x^{2} z - 18 x^{2} + 18 x y^{2} - 32 x y z - 27 x y - 24 x z^{2} + 24 x z + 9 x - 26 y^{2} z - 39 y z^{2} + 39 y z - 13 z^{3} + 26 z^{2} - 13 z\right)\\\displaystyle 15 z \left(- 9 x^{3} + 48 x^{2} y - 27 x^{2} z + 18 x^{2} + 48 x y^{2} + 64 x y z - 48 x y - 18 x z^{2} + 27 x z - 9 x + 17 y^{3} + 51 y^{2} z - 34 y^{2} + 34 y z^{2} - 51 y z + 17 y\right)\end{array}\right)\)
This DOF is associated with face 0 of the reference element.
where \(f_{0}\) is the 0th face;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{0}\).
\(\displaystyle \boldsymbol{\phi}_{13} = \left(\begin{array}{c}\displaystyle 15 x \left(- 34 x^{2} y + 26 x^{2} z - 51 x y^{2} - 32 x y z + 51 x y + 39 x z^{2} - 39 x z - 17 y^{3} - 24 y^{2} z + 34 y^{2} - 24 y z^{2} + 24 y z - 17 y + 13 z^{3} - 26 z^{2} + 13 z\right)\\\displaystyle 15 y \left(9 x^{3} + 27 x^{2} y - 24 x^{2} z - 18 x^{2} + 18 x y^{2} - 32 x y z - 27 x y - 24 x z^{2} + 24 x z + 9 x - 26 y^{2} z - 39 y z^{2} + 39 y z - 13 z^{3} + 26 z^{2} - 13 z\right)\\\displaystyle 15 z \left(- 9 x^{3} + 48 x^{2} y - 27 x^{2} z + 18 x^{2} + 48 x y^{2} + 64 x y z - 48 x y - 18 x z^{2} + 27 x z - 9 x + 17 y^{3} + 51 y^{2} z - 34 y^{2} + 34 y z^{2} - 51 y z + 17 y\right)\end{array}\right)\)
This DOF is associated with face 0 of the reference element.
\(\displaystyle l_{14}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{0}}\boldsymbol{v}\cdot\left(\begin{array}{c}\frac{\sqrt{3} \left(- s_{0} + s_{1} - 1\right)}{3}\\\frac{\sqrt{3} \left(1 - s_{1}\right)}{3}\\\frac{\sqrt{3} s_{0}}{3}\end{array}\right)\)
where \(f_{0}\) is the 0th face;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{0}\).
\(\displaystyle \boldsymbol{\phi}_{14} = \left(\begin{array}{c}\displaystyle 15 x \left(26 x^{2} y - 34 x^{2} z + 39 x y^{2} - 32 x y z - 39 x y - 51 x z^{2} + 51 x z + 13 y^{3} - 24 y^{2} z - 26 y^{2} - 24 y z^{2} + 24 y z + 13 y - 17 z^{3} + 34 z^{2} - 17 z\right)\\\displaystyle 15 y \left(- 9 x^{3} - 27 x^{2} y + 48 x^{2} z + 18 x^{2} - 18 x y^{2} + 64 x y z + 27 x y + 48 x z^{2} - 48 x z - 9 x + 34 y^{2} z + 51 y z^{2} - 51 y z + 17 z^{3} - 34 z^{2} + 17 z\right)\\\displaystyle 15 z \left(9 x^{3} - 24 x^{2} y + 27 x^{2} z - 18 x^{2} - 24 x y^{2} - 32 x y z + 24 x y + 18 x z^{2} - 27 x z + 9 x - 13 y^{3} - 39 y^{2} z + 26 y^{2} - 26 y z^{2} + 39 y z - 13 y\right)\end{array}\right)\)
This DOF is associated with face 0 of the reference element.
where \(f_{0}\) is the 0th face;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{0}\).
\(\displaystyle \boldsymbol{\phi}_{14} = \left(\begin{array}{c}\displaystyle 15 x \left(26 x^{2} y - 34 x^{2} z + 39 x y^{2} - 32 x y z - 39 x y - 51 x z^{2} + 51 x z + 13 y^{3} - 24 y^{2} z - 26 y^{2} - 24 y z^{2} + 24 y z + 13 y - 17 z^{3} + 34 z^{2} - 17 z\right)\\\displaystyle 15 y \left(- 9 x^{3} - 27 x^{2} y + 48 x^{2} z + 18 x^{2} - 18 x y^{2} + 64 x y z + 27 x y + 48 x z^{2} - 48 x z - 9 x + 34 y^{2} z + 51 y z^{2} - 51 y z + 17 z^{3} - 34 z^{2} + 17 z\right)\\\displaystyle 15 z \left(9 x^{3} - 24 x^{2} y + 27 x^{2} z - 18 x^{2} - 24 x y^{2} - 32 x y z + 24 x y + 18 x z^{2} - 27 x z + 9 x - 13 y^{3} - 39 y^{2} z + 26 y^{2} - 26 y z^{2} + 39 y z - 13 y\right)\end{array}\right)\)
This DOF is associated with face 0 of the reference element.
\(\displaystyle l_{15}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{1}}\boldsymbol{v}\cdot\left(\begin{array}{c}0\\- s_{1}\\s_{0}\end{array}\right)\)
where \(f_{1}\) is the 1st face;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{1}\).
\(\displaystyle \boldsymbol{\phi}_{15} = \left(\begin{array}{c}\displaystyle 180 x \left(3 x^{2} y - 3 x^{2} z + 9 x y^{2} - 6 x y - 9 x z^{2} + 6 x z + 6 y^{3} + 7 y^{2} z - 9 y^{2} - 7 y z^{2} + 3 y - 6 z^{3} + 9 z^{2} - 3 z\right)\\\displaystyle 180 y \left(2 x^{3} - 3 x^{2} y + 3 x^{2} z - x^{2} - 5 x y^{2} - 4 x y z + 6 x y + 3 x z^{2} - x + 5 y^{2} z + 11 y z^{2} - 8 y z + 6 z^{3} - 9 z^{2} + 3 z\right)\\\displaystyle 180 z \left(- 2 x^{3} - 3 x^{2} y + 3 x^{2} z + x^{2} - 3 x y^{2} + 4 x y z + 5 x z^{2} - 6 x z + x - 6 y^{3} - 11 y^{2} z + 9 y^{2} - 5 y z^{2} + 8 y z - 3 y\right)\end{array}\right)\)
This DOF is associated with face 1 of the reference element.
where \(f_{1}\) is the 1st face;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{1}\).
\(\displaystyle \boldsymbol{\phi}_{15} = \left(\begin{array}{c}\displaystyle 180 x \left(3 x^{2} y - 3 x^{2} z + 9 x y^{2} - 6 x y - 9 x z^{2} + 6 x z + 6 y^{3} + 7 y^{2} z - 9 y^{2} - 7 y z^{2} + 3 y - 6 z^{3} + 9 z^{2} - 3 z\right)\\\displaystyle 180 y \left(2 x^{3} - 3 x^{2} y + 3 x^{2} z - x^{2} - 5 x y^{2} - 4 x y z + 6 x y + 3 x z^{2} - x + 5 y^{2} z + 11 y z^{2} - 8 y z + 6 z^{3} - 9 z^{2} + 3 z\right)\\\displaystyle 180 z \left(- 2 x^{3} - 3 x^{2} y + 3 x^{2} z + x^{2} - 3 x y^{2} + 4 x y z + 5 x z^{2} - 6 x z + x - 6 y^{3} - 11 y^{2} z + 9 y^{2} - 5 y z^{2} + 8 y z - 3 y\right)\end{array}\right)\)
This DOF is associated with face 1 of the reference element.
\(\displaystyle l_{16}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{1}}\boldsymbol{v}\cdot\left(\begin{array}{c}0\\s_{1}\\1 - s_{0}\end{array}\right)\)
where \(f_{1}\) is the 1st face;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{1}\).
\(\displaystyle \boldsymbol{\phi}_{16} = \left(\begin{array}{c}\displaystyle 45 x \left(- 26 x^{2} y + 18 x^{2} z - 51 x y^{2} - 16 x y z + 43 x y + 39 x z^{2} - 31 x z - 25 y^{3} - 32 y^{2} z + 42 y^{2} + 24 y z^{2} + 8 y z - 17 y + 21 z^{3} - 34 z^{2} + 13 z\right)\\\displaystyle 45 y \left(- 3 x^{3} + 15 x^{2} y - 24 x^{2} z - 2 x^{2} + 18 x y^{2} - 23 x y - 24 x z^{2} + 16 x z + 5 x - 18 y^{2} z - 39 y z^{2} + 31 y z - 21 z^{3} + 34 z^{2} - 13 z\right)\\\displaystyle 45 z \left(3 x^{3} + 48 x^{2} y - 15 x^{2} z + 2 x^{2} + 48 x y^{2} + 16 x y z - 40 x y - 18 x z^{2} + 23 x z - 5 x + 25 y^{3} + 43 y^{2} z - 42 y^{2} + 18 y z^{2} - 35 y z + 17 y\right)\end{array}\right)\)
This DOF is associated with face 1 of the reference element.
where \(f_{1}\) is the 1st face;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{1}\).
\(\displaystyle \boldsymbol{\phi}_{16} = \left(\begin{array}{c}\displaystyle 45 x \left(- 26 x^{2} y + 18 x^{2} z - 51 x y^{2} - 16 x y z + 43 x y + 39 x z^{2} - 31 x z - 25 y^{3} - 32 y^{2} z + 42 y^{2} + 24 y z^{2} + 8 y z - 17 y + 21 z^{3} - 34 z^{2} + 13 z\right)\\\displaystyle 45 y \left(- 3 x^{3} + 15 x^{2} y - 24 x^{2} z - 2 x^{2} + 18 x y^{2} - 23 x y - 24 x z^{2} + 16 x z + 5 x - 18 y^{2} z - 39 y z^{2} + 31 y z - 21 z^{3} + 34 z^{2} - 13 z\right)\\\displaystyle 45 z \left(3 x^{3} + 48 x^{2} y - 15 x^{2} z + 2 x^{2} + 48 x y^{2} + 16 x y z - 40 x y - 18 x z^{2} + 23 x z - 5 x + 25 y^{3} + 43 y^{2} z - 42 y^{2} + 18 y z^{2} - 35 y z + 17 y\right)\end{array}\right)\)
This DOF is associated with face 1 of the reference element.
\(\displaystyle l_{17}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{1}}\boldsymbol{v}\cdot\left(\begin{array}{c}0\\1 - s_{1}\\s_{0}\end{array}\right)\)
where \(f_{1}\) is the 1st face;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{1}\).
\(\displaystyle \boldsymbol{\phi}_{17} = \left(\begin{array}{c}\displaystyle 45 x \left(18 x^{2} y - 26 x^{2} z + 39 x y^{2} - 16 x y z - 31 x y - 51 x z^{2} + 43 x z + 21 y^{3} + 24 y^{2} z - 34 y^{2} - 32 y z^{2} + 8 y z + 13 y - 25 z^{3} + 42 z^{2} - 17 z\right)\\\displaystyle 45 y \left(3 x^{3} - 15 x^{2} y + 48 x^{2} z + 2 x^{2} - 18 x y^{2} + 16 x y z + 23 x y + 48 x z^{2} - 40 x z - 5 x + 18 y^{2} z + 43 y z^{2} - 35 y z + 25 z^{3} - 42 z^{2} + 17 z\right)\\\displaystyle 45 z \left(- 3 x^{3} - 24 x^{2} y + 15 x^{2} z - 2 x^{2} - 24 x y^{2} + 16 x y + 18 x z^{2} - 23 x z + 5 x - 21 y^{3} - 39 y^{2} z + 34 y^{2} - 18 y z^{2} + 31 y z - 13 y\right)\end{array}\right)\)
This DOF is associated with face 1 of the reference element.
where \(f_{1}\) is the 1st face;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{1}\).
\(\displaystyle \boldsymbol{\phi}_{17} = \left(\begin{array}{c}\displaystyle 45 x \left(18 x^{2} y - 26 x^{2} z + 39 x y^{2} - 16 x y z - 31 x y - 51 x z^{2} + 43 x z + 21 y^{3} + 24 y^{2} z - 34 y^{2} - 32 y z^{2} + 8 y z + 13 y - 25 z^{3} + 42 z^{2} - 17 z\right)\\\displaystyle 45 y \left(3 x^{3} - 15 x^{2} y + 48 x^{2} z + 2 x^{2} - 18 x y^{2} + 16 x y z + 23 x y + 48 x z^{2} - 40 x z - 5 x + 18 y^{2} z + 43 y z^{2} - 35 y z + 25 z^{3} - 42 z^{2} + 17 z\right)\\\displaystyle 45 z \left(- 3 x^{3} - 24 x^{2} y + 15 x^{2} z - 2 x^{2} - 24 x y^{2} + 16 x y + 18 x z^{2} - 23 x z + 5 x - 21 y^{3} - 39 y^{2} z + 34 y^{2} - 18 y z^{2} + 31 y z - 13 y\right)\end{array}\right)\)
This DOF is associated with face 1 of the reference element.
\(\displaystyle l_{18}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{2}}\boldsymbol{v}\cdot\left(\begin{array}{c}- s_{1}\\0\\s_{0}\end{array}\right)\)
where \(f_{2}\) is the 2nd face;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{2}\).
\(\displaystyle \boldsymbol{\phi}_{18} = \left(\begin{array}{c}\displaystyle 90 x \left(- 4 x^{2} y + 4 x^{2} z + 3 x y^{2} - 8 x y z + 3 x y + 13 x z^{2} - 7 x z + 7 y^{3} + 6 y^{2} z - 8 y^{2} + 6 y z^{2} + y + 9 z^{3} - 12 z^{2} + 3 z\right)\\\displaystyle 90 y \left(9 x^{3} + 9 x^{2} y + 14 x^{2} z - 12 x^{2} - 3 x y - 14 x z^{2} + 3 x - 9 y z^{2} + 3 y z - 9 z^{3} + 12 z^{2} - 3 z\right)\\\displaystyle 90 z \left(- 9 x^{3} - 6 x^{2} y - 13 x^{2} z + 12 x^{2} - 6 x y^{2} + 8 x y z - 4 x z^{2} + 7 x z - 3 x - 7 y^{3} - 3 y^{2} z + 8 y^{2} + 4 y z^{2} - 3 y z - y\right)\end{array}\right)\)
This DOF is associated with face 2 of the reference element.
where \(f_{2}\) is the 2nd face;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{2}\).
\(\displaystyle \boldsymbol{\phi}_{18} = \left(\begin{array}{c}\displaystyle 90 x \left(- 4 x^{2} y + 4 x^{2} z + 3 x y^{2} - 8 x y z + 3 x y + 13 x z^{2} - 7 x z + 7 y^{3} + 6 y^{2} z - 8 y^{2} + 6 y z^{2} + y + 9 z^{3} - 12 z^{2} + 3 z\right)\\\displaystyle 90 y \left(9 x^{3} + 9 x^{2} y + 14 x^{2} z - 12 x^{2} - 3 x y - 14 x z^{2} + 3 x - 9 y z^{2} + 3 y z - 9 z^{3} + 12 z^{2} - 3 z\right)\\\displaystyle 90 z \left(- 9 x^{3} - 6 x^{2} y - 13 x^{2} z + 12 x^{2} - 6 x y^{2} + 8 x y z - 4 x z^{2} + 7 x z - 3 x - 7 y^{3} - 3 y^{2} z + 8 y^{2} + 4 y z^{2} - 3 y z - y\right)\end{array}\right)\)
This DOF is associated with face 2 of the reference element.
\(\displaystyle l_{19}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{2}}\boldsymbol{v}\cdot\left(\begin{array}{c}s_{1}\\0\\1 - s_{0}\end{array}\right)\)
where \(f_{2}\) is the 2nd face;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{2}\).
\(\displaystyle \boldsymbol{\phi}_{19} = \left(\begin{array}{c}\displaystyle 90 x \left(2 x^{2} y - 2 x^{2} z - 3 x y^{2} - x y - 9 x z^{2} + 5 x z - 5 y^{3} - 12 y^{2} z + 6 y^{2} - 12 y z^{2} + 8 y z - y - 7 z^{3} + 10 z^{2} - 3 z\right)\\\displaystyle 90 y \left(- 9 x^{3} - 15 x^{2} y - 16 x^{2} z + 14 x^{2} - 6 x y^{2} - 8 x y z + 11 x y + 12 x z^{2} + 4 x z - 5 x + 2 y^{2} z + 9 y z^{2} - 5 y z + 7 z^{3} - 10 z^{2} + 3 z\right)\\\displaystyle 90 z \left(9 x^{3} + 24 x^{2} y + 11 x^{2} z - 14 x^{2} + 24 x y^{2} + 8 x y z - 20 x y + 2 x z^{2} - 7 x z + 5 x + 5 y^{3} + 3 y^{2} z - 6 y^{2} - 2 y z^{2} + y z + y\right)\end{array}\right)\)
This DOF is associated with face 2 of the reference element.
where \(f_{2}\) is the 2nd face;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{2}\).
\(\displaystyle \boldsymbol{\phi}_{19} = \left(\begin{array}{c}\displaystyle 90 x \left(2 x^{2} y - 2 x^{2} z - 3 x y^{2} - x y - 9 x z^{2} + 5 x z - 5 y^{3} - 12 y^{2} z + 6 y^{2} - 12 y z^{2} + 8 y z - y - 7 z^{3} + 10 z^{2} - 3 z\right)\\\displaystyle 90 y \left(- 9 x^{3} - 15 x^{2} y - 16 x^{2} z + 14 x^{2} - 6 x y^{2} - 8 x y z + 11 x y + 12 x z^{2} + 4 x z - 5 x + 2 y^{2} z + 9 y z^{2} - 5 y z + 7 z^{3} - 10 z^{2} + 3 z\right)\\\displaystyle 90 z \left(9 x^{3} + 24 x^{2} y + 11 x^{2} z - 14 x^{2} + 24 x y^{2} + 8 x y z - 20 x y + 2 x z^{2} - 7 x z + 5 x + 5 y^{3} + 3 y^{2} z - 6 y^{2} - 2 y z^{2} + y z + y\right)\end{array}\right)\)
This DOF is associated with face 2 of the reference element.
\(\displaystyle l_{20}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{2}}\boldsymbol{v}\cdot\left(\begin{array}{c}1 - s_{1}\\0\\s_{0}\end{array}\right)\)
where \(f_{2}\) is the 2nd face;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{2}\).
\(\displaystyle \boldsymbol{\phi}_{20} = \left(\begin{array}{c}\displaystyle 45 x \left(- 2 x^{2} y + 2 x^{2} z + 9 x y^{2} + 16 x y z - x y + 19 x z^{2} - 11 x z + 11 y^{3} + 48 y^{2} z - 14 y^{2} + 48 y z^{2} - 40 y z + 3 y + 17 z^{3} - 26 z^{2} + 9 z\right)\\\displaystyle 45 y \left(13 x^{3} + 15 x^{2} y + 24 x^{2} z - 18 x^{2} + 2 x y^{2} - 16 x y z - 7 x y - 32 x z^{2} + 8 x z + 5 x - 10 y^{2} z - 27 y z^{2} + 19 y z - 17 z^{3} + 26 z^{2} - 9 z\right)\\\displaystyle 45 z \left(- 13 x^{3} - 24 x^{2} y - 15 x^{2} z + 18 x^{2} - 24 x y^{2} + 16 x y - 2 x z^{2} + 7 x z - 5 x - 11 y^{3} - 9 y^{2} z + 14 y^{2} + 2 y z^{2} + y z - 3 y\right)\end{array}\right)\)
This DOF is associated with face 2 of the reference element.
where \(f_{2}\) is the 2nd face;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{2}\).
\(\displaystyle \boldsymbol{\phi}_{20} = \left(\begin{array}{c}\displaystyle 45 x \left(- 2 x^{2} y + 2 x^{2} z + 9 x y^{2} + 16 x y z - x y + 19 x z^{2} - 11 x z + 11 y^{3} + 48 y^{2} z - 14 y^{2} + 48 y z^{2} - 40 y z + 3 y + 17 z^{3} - 26 z^{2} + 9 z\right)\\\displaystyle 45 y \left(13 x^{3} + 15 x^{2} y + 24 x^{2} z - 18 x^{2} + 2 x y^{2} - 16 x y z - 7 x y - 32 x z^{2} + 8 x z + 5 x - 10 y^{2} z - 27 y z^{2} + 19 y z - 17 z^{3} + 26 z^{2} - 9 z\right)\\\displaystyle 45 z \left(- 13 x^{3} - 24 x^{2} y - 15 x^{2} z + 18 x^{2} - 24 x y^{2} + 16 x y - 2 x z^{2} + 7 x z - 5 x - 11 y^{3} - 9 y^{2} z + 14 y^{2} + 2 y z^{2} + y z - 3 y\right)\end{array}\right)\)
This DOF is associated with face 2 of the reference element.
\(\displaystyle l_{21}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{3}}\boldsymbol{v}\cdot\left(\begin{array}{c}- s_{1}\\s_{0}\\0\end{array}\right)\)
where \(f_{3}\) is the 3rd face;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{3}\).
\(\displaystyle \boldsymbol{\phi}_{21} = \left(\begin{array}{c}\displaystyle 90 x \left(4 x^{2} y - 4 x^{2} z + 13 x y^{2} - 8 x y z - 7 x y + 3 x z^{2} + 3 x z + 9 y^{3} + 6 y^{2} z - 12 y^{2} + 6 y z^{2} + 3 y + 7 z^{3} - 8 z^{2} + z\right)\\\displaystyle 90 y \left(- 9 x^{3} - 13 x^{2} y - 6 x^{2} z + 12 x^{2} - 4 x y^{2} + 8 x y z + 7 x y - 6 x z^{2} - 3 x + 4 y^{2} z - 3 y z^{2} - 3 y z - 7 z^{3} + 8 z^{2} - z\right)\\\displaystyle 90 z \left(9 x^{3} + 14 x^{2} y + 9 x^{2} z - 12 x^{2} - 14 x y^{2} - 3 x z + 3 x - 9 y^{3} - 9 y^{2} z + 12 y^{2} + 3 y z - 3 y\right)\end{array}\right)\)
This DOF is associated with face 3 of the reference element.
where \(f_{3}\) is the 3rd face;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{3}\).
\(\displaystyle \boldsymbol{\phi}_{21} = \left(\begin{array}{c}\displaystyle 90 x \left(4 x^{2} y - 4 x^{2} z + 13 x y^{2} - 8 x y z - 7 x y + 3 x z^{2} + 3 x z + 9 y^{3} + 6 y^{2} z - 12 y^{2} + 6 y z^{2} + 3 y + 7 z^{3} - 8 z^{2} + z\right)\\\displaystyle 90 y \left(- 9 x^{3} - 13 x^{2} y - 6 x^{2} z + 12 x^{2} - 4 x y^{2} + 8 x y z + 7 x y - 6 x z^{2} - 3 x + 4 y^{2} z - 3 y z^{2} - 3 y z - 7 z^{3} + 8 z^{2} - z\right)\\\displaystyle 90 z \left(9 x^{3} + 14 x^{2} y + 9 x^{2} z - 12 x^{2} - 14 x y^{2} - 3 x z + 3 x - 9 y^{3} - 9 y^{2} z + 12 y^{2} + 3 y z - 3 y\right)\end{array}\right)\)
This DOF is associated with face 3 of the reference element.
\(\displaystyle l_{22}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{3}}\boldsymbol{v}\cdot\left(\begin{array}{c}s_{1}\\1 - s_{0}\\0\end{array}\right)\)
where \(f_{3}\) is the 3rd face;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{3}\).
\(\displaystyle \boldsymbol{\phi}_{22} = \left(\begin{array}{c}\displaystyle 90 x \left(- 2 x^{2} y + 2 x^{2} z - 9 x y^{2} + 5 x y - 3 x z^{2} - x z - 7 y^{3} - 12 y^{2} z + 10 y^{2} - 12 y z^{2} + 8 y z - 3 y - 5 z^{3} + 6 z^{2} - z\right)\\\displaystyle 90 y \left(9 x^{3} + 11 x^{2} y + 24 x^{2} z - 14 x^{2} + 2 x y^{2} + 8 x y z - 7 x y + 24 x z^{2} - 20 x z + 5 x - 2 y^{2} z + 3 y z^{2} + y z + 5 z^{3} - 6 z^{2} + z\right)\\\displaystyle 90 z \left(- 9 x^{3} - 16 x^{2} y - 15 x^{2} z + 14 x^{2} + 12 x y^{2} - 8 x y z + 4 x y - 6 x z^{2} + 11 x z - 5 x + 7 y^{3} + 9 y^{2} z - 10 y^{2} + 2 y z^{2} - 5 y z + 3 y\right)\end{array}\right)\)
This DOF is associated with face 3 of the reference element.
where \(f_{3}\) is the 3rd face;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{3}\).
\(\displaystyle \boldsymbol{\phi}_{22} = \left(\begin{array}{c}\displaystyle 90 x \left(- 2 x^{2} y + 2 x^{2} z - 9 x y^{2} + 5 x y - 3 x z^{2} - x z - 7 y^{3} - 12 y^{2} z + 10 y^{2} - 12 y z^{2} + 8 y z - 3 y - 5 z^{3} + 6 z^{2} - z\right)\\\displaystyle 90 y \left(9 x^{3} + 11 x^{2} y + 24 x^{2} z - 14 x^{2} + 2 x y^{2} + 8 x y z - 7 x y + 24 x z^{2} - 20 x z + 5 x - 2 y^{2} z + 3 y z^{2} + y z + 5 z^{3} - 6 z^{2} + z\right)\\\displaystyle 90 z \left(- 9 x^{3} - 16 x^{2} y - 15 x^{2} z + 14 x^{2} + 12 x y^{2} - 8 x y z + 4 x y - 6 x z^{2} + 11 x z - 5 x + 7 y^{3} + 9 y^{2} z - 10 y^{2} + 2 y z^{2} - 5 y z + 3 y\right)\end{array}\right)\)
This DOF is associated with face 3 of the reference element.
\(\displaystyle l_{23}:\boldsymbol{v}\mapsto\displaystyle\int_{f_{3}}\boldsymbol{v}\cdot\left(\begin{array}{c}1 - s_{1}\\s_{0}\\0\end{array}\right)\)
where \(f_{3}\) is the 3rd face;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{3}\).
\(\displaystyle \boldsymbol{\phi}_{23} = \left(\begin{array}{c}\displaystyle 45 x \left(2 x^{2} y - 2 x^{2} z + 19 x y^{2} + 16 x y z - 11 x y + 9 x z^{2} - x z + 17 y^{3} + 48 y^{2} z - 26 y^{2} + 48 y z^{2} - 40 y z + 9 y + 11 z^{3} - 14 z^{2} + 3 z\right)\\\displaystyle 45 y \left(- 13 x^{3} - 15 x^{2} y - 24 x^{2} z + 18 x^{2} - 2 x y^{2} + 7 x y - 24 x z^{2} + 16 x z - 5 x + 2 y^{2} z - 9 y z^{2} + y z - 11 z^{3} + 14 z^{2} - 3 z\right)\\\displaystyle 45 z \left(13 x^{3} + 24 x^{2} y + 15 x^{2} z - 18 x^{2} - 32 x y^{2} - 16 x y z + 8 x y + 2 x z^{2} - 7 x z + 5 x - 17 y^{3} - 27 y^{2} z + 26 y^{2} - 10 y z^{2} + 19 y z - 9 y\right)\end{array}\right)\)
This DOF is associated with face 3 of the reference element.
where \(f_{3}\) is the 3rd face;
and \(s_{0},s_{1},s_{2}\) is a parametrisation of \(f_{3}\).
\(\displaystyle \boldsymbol{\phi}_{23} = \left(\begin{array}{c}\displaystyle 45 x \left(2 x^{2} y - 2 x^{2} z + 19 x y^{2} + 16 x y z - 11 x y + 9 x z^{2} - x z + 17 y^{3} + 48 y^{2} z - 26 y^{2} + 48 y z^{2} - 40 y z + 9 y + 11 z^{3} - 14 z^{2} + 3 z\right)\\\displaystyle 45 y \left(- 13 x^{3} - 15 x^{2} y - 24 x^{2} z + 18 x^{2} - 2 x y^{2} + 7 x y - 24 x z^{2} + 16 x z - 5 x + 2 y^{2} z - 9 y z^{2} + y z - 11 z^{3} + 14 z^{2} - 3 z\right)\\\displaystyle 45 z \left(13 x^{3} + 24 x^{2} y + 15 x^{2} z - 18 x^{2} - 32 x y^{2} - 16 x y z + 8 x y + 2 x z^{2} - 7 x z + 5 x - 17 y^{3} - 27 y^{2} z + 26 y^{2} - 10 y z^{2} + 19 y z - 9 y\right)\end{array}\right)\)
This DOF is associated with face 3 of the reference element.