an encyclopedia of finite element definitions
Degree 5 conforming Crouzeix–Raviart on a triangle
◀ Back to conforming Crouzeix–Raviart definition page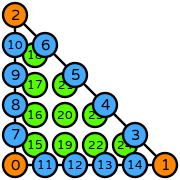
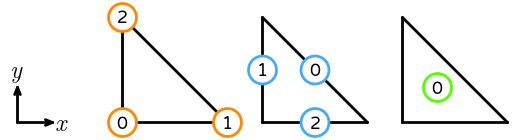
- \(R\) is the reference triangle. The following numbering of the subentities of the reference cell is used:
- \(\mathcal{V}\) is spanned by: \(1\), \(x\), \(x^{2}\), \(x^{3}\), \(x^{4}\), \(x^{5}\), \(y\), \(x y\), \(x^{2} y\), \(x^{3} y\), \(x^{4} y\), \(y^{2}\), \(x y^{2}\), \(x^{2} y^{2}\), \(x^{3} y^{2}\), \(y^{3}\), \(x y^{3}\), \(x^{2} y^{3}\), \(y^{4}\), \(x y^{4}\), \(y^{5}\), \(x y^{4} \left(x + y\right)\), \(x^{2} y^{3} \left(x + y\right)\), \(x^{3} y^{2} \left(x + y\right)\), \(x^{4} y \left(x + y\right)\)
- \(\mathcal{L}=\{l_0,...,l_{24}\}\)
- Functionals and basis functions:
\(\displaystyle l_{0}:v\mapsto v(0,0)\)
\(\displaystyle \phi_{0} = \frac{46875 x^{5} y}{352} - \frac{625 x^{5}}{24} + \frac{41625 x^{4} y^{2}}{88} - \frac{171575 x^{4} y}{352} + \frac{625 x^{4}}{8} + \frac{10875 x^{3} y^{3}}{16} - \frac{1297825 x^{3} y^{2}}{1056} + \frac{695575 x^{3} y}{1056} - \frac{2125 x^{3}}{24} + \frac{41625 x^{2} y^{4}}{88} - \frac{1297825 x^{2} y^{3}}{1056} + \frac{26825 x^{2} y^{2}}{24} - \frac{432671 x^{2} y}{1056} + \frac{375 x^{2}}{8} + \frac{46875 x y^{5}}{352} - \frac{171575 x y^{4}}{352} + \frac{695575 x y^{3}}{1056} - \frac{432671 x y^{2}}{1056} + \frac{10183 x y}{88} - \frac{137 x}{12} - \frac{625 y^{5}}{24} + \frac{625 y^{4}}{8} - \frac{2125 y^{3}}{24} + \frac{375 y^{2}}{8} - \frac{137 y}{12} + 1\)
This DOF is associated with vertex 0 of the reference cell.
\(\displaystyle \phi_{0} = \frac{46875 x^{5} y}{352} - \frac{625 x^{5}}{24} + \frac{41625 x^{4} y^{2}}{88} - \frac{171575 x^{4} y}{352} + \frac{625 x^{4}}{8} + \frac{10875 x^{3} y^{3}}{16} - \frac{1297825 x^{3} y^{2}}{1056} + \frac{695575 x^{3} y}{1056} - \frac{2125 x^{3}}{24} + \frac{41625 x^{2} y^{4}}{88} - \frac{1297825 x^{2} y^{3}}{1056} + \frac{26825 x^{2} y^{2}}{24} - \frac{432671 x^{2} y}{1056} + \frac{375 x^{2}}{8} + \frac{46875 x y^{5}}{352} - \frac{171575 x y^{4}}{352} + \frac{695575 x y^{3}}{1056} - \frac{432671 x y^{2}}{1056} + \frac{10183 x y}{88} - \frac{137 x}{12} - \frac{625 y^{5}}{24} + \frac{625 y^{4}}{8} - \frac{2125 y^{3}}{24} + \frac{375 y^{2}}{8} - \frac{137 y}{12} + 1\)
This DOF is associated with vertex 0 of the reference cell.
\(\displaystyle l_{1}:v\mapsto v(1,0)\)
\(\displaystyle \phi_{1} = \frac{x \left(- 140625 x^{4} y + 27500 x^{4} - 203625 x^{3} y^{2} + 325900 x^{3} y - 55000 x^{3} - 126000 x^{2} y^{3} + 328425 x^{2} y^{2} - 262925 x^{2} y + 38500 x^{2} - 63000 x y^{4} + 160300 x y^{3} - 174950 x y^{2} + 87454 x y - 11000 x + 17150 y^{4} - 34300 y^{3} + 26954 y^{2} - 9804 y + 1056\right)}{1056}\)
This DOF is associated with vertex 1 of the reference cell.
\(\displaystyle \phi_{1} = \frac{x \left(- 140625 x^{4} y + 27500 x^{4} - 203625 x^{3} y^{2} + 325900 x^{3} y - 55000 x^{3} - 126000 x^{2} y^{3} + 328425 x^{2} y^{2} - 262925 x^{2} y + 38500 x^{2} - 63000 x y^{4} + 160300 x y^{3} - 174950 x y^{2} + 87454 x y - 11000 x + 17150 y^{4} - 34300 y^{3} + 26954 y^{2} - 9804 y + 1056\right)}{1056}\)
This DOF is associated with vertex 1 of the reference cell.
\(\displaystyle l_{2}:v\mapsto v(0,1)\)
\(\displaystyle \phi_{2} = \frac{y \left(- 63000 x^{4} y + 17150 x^{4} - 126000 x^{3} y^{2} + 160300 x^{3} y - 34300 x^{3} - 203625 x^{2} y^{3} + 328425 x^{2} y^{2} - 174950 x^{2} y + 26954 x^{2} - 140625 x y^{4} + 325900 x y^{3} - 262925 x y^{2} + 87454 x y - 9804 x + 27500 y^{4} - 55000 y^{3} + 38500 y^{2} - 11000 y + 1056\right)}{1056}\)
This DOF is associated with vertex 2 of the reference cell.
\(\displaystyle \phi_{2} = \frac{y \left(- 63000 x^{4} y + 17150 x^{4} - 126000 x^{3} y^{2} + 160300 x^{3} y - 34300 x^{3} - 203625 x^{2} y^{3} + 328425 x^{2} y^{2} - 174950 x^{2} y + 26954 x^{2} - 140625 x y^{4} + 325900 x y^{3} - 262925 x y^{2} + 87454 x y - 9804 x + 27500 y^{4} - 55000 y^{3} + 38500 y^{2} - 11000 y + 1056\right)}{1056}\)
This DOF is associated with vertex 2 of the reference cell.
\(\displaystyle l_{3}:v\mapsto v(\tfrac{4}{5},\tfrac{1}{5})\)
\(\displaystyle \phi_{3} = \frac{25 x y \left(26250 x^{4} + 18375 x^{3} y - 47950 x^{3} - 3375 x^{2} y^{2} - 16800 x^{2} y + 29250 x^{2} + 1875 x y^{3} - 300 x y^{2} + 5205 x y - 6962 x - 2625 y^{4} + 4300 y^{3} - 2595 y^{2} + 188 y + 468\right)}{1056}\)
This DOF is associated with edge 0 of the reference cell.
\(\displaystyle \phi_{3} = \frac{25 x y \left(26250 x^{4} + 18375 x^{3} y - 47950 x^{3} - 3375 x^{2} y^{2} - 16800 x^{2} y + 29250 x^{2} + 1875 x y^{3} - 300 x y^{2} + 5205 x y - 6962 x - 2625 y^{4} + 4300 y^{3} - 2595 y^{2} + 188 y + 468\right)}{1056}\)
This DOF is associated with edge 0 of the reference cell.
\(\displaystyle l_{4}:v\mapsto v(\tfrac{3}{5},\tfrac{2}{5})\)
\(\displaystyle \phi_{4} = \frac{25 x y \left(- 13125 x^{4} + 34125 x^{3} y + 18200 x^{3} + 32625 x^{2} y^{2} - 49350 x^{2} y - 7475 x^{2} - 7125 x y^{3} - 8100 x y^{2} + 15025 x y + 1292 x + 7500 y^{4} - 12050 y^{3} + 8200 y^{2} - 2998 y + 52\right)}{1056}\)
This DOF is associated with edge 0 of the reference cell.
\(\displaystyle \phi_{4} = \frac{25 x y \left(- 13125 x^{4} + 34125 x^{3} y + 18200 x^{3} + 32625 x^{2} y^{2} - 49350 x^{2} y - 7475 x^{2} - 7125 x y^{3} - 8100 x y^{2} + 15025 x y + 1292 x + 7500 y^{4} - 12050 y^{3} + 8200 y^{2} - 2998 y + 52\right)}{1056}\)
This DOF is associated with edge 0 of the reference cell.
\(\displaystyle l_{5}:v\mapsto v(\tfrac{2}{5},\tfrac{3}{5})\)
\(\displaystyle \phi_{5} = \frac{25 x y \left(7500 x^{4} - 7125 x^{3} y - 12050 x^{3} + 32625 x^{2} y^{2} - 8100 x^{2} y + 8200 x^{2} + 34125 x y^{3} - 49350 x y^{2} + 15025 x y - 2998 x - 13125 y^{4} + 18200 y^{3} - 7475 y^{2} + 1292 y + 52\right)}{1056}\)
This DOF is associated with edge 0 of the reference cell.
\(\displaystyle \phi_{5} = \frac{25 x y \left(7500 x^{4} - 7125 x^{3} y - 12050 x^{3} + 32625 x^{2} y^{2} - 8100 x^{2} y + 8200 x^{2} + 34125 x y^{3} - 49350 x y^{2} + 15025 x y - 2998 x - 13125 y^{4} + 18200 y^{3} - 7475 y^{2} + 1292 y + 52\right)}{1056}\)
This DOF is associated with edge 0 of the reference cell.
\(\displaystyle l_{6}:v\mapsto v(\tfrac{1}{5},\tfrac{4}{5})\)
\(\displaystyle \phi_{6} = \frac{25 x y \left(- 2625 x^{4} + 1875 x^{3} y + 4300 x^{3} - 3375 x^{2} y^{2} - 300 x^{2} y - 2595 x^{2} + 18375 x y^{3} - 16800 x y^{2} + 5205 x y + 188 x + 26250 y^{4} - 47950 y^{3} + 29250 y^{2} - 6962 y + 468\right)}{1056}\)
This DOF is associated with edge 0 of the reference cell.
\(\displaystyle \phi_{6} = \frac{25 x y \left(- 2625 x^{4} + 1875 x^{3} y + 4300 x^{3} - 3375 x^{2} y^{2} - 300 x^{2} y - 2595 x^{2} + 18375 x y^{3} - 16800 x y^{2} + 5205 x y + 188 x + 26250 y^{4} - 47950 y^{3} + 29250 y^{2} - 6962 y + 468\right)}{1056}\)
This DOF is associated with edge 0 of the reference cell.
\(\displaystyle l_{7}:v\mapsto v(0,\tfrac{1}{5})\)
\(\displaystyle \phi_{7} = \frac{25 y \left(- 26250 x^{5} - 112875 x^{4} y + 83300 x^{4} - 185625 x^{3} y^{2} + 276500 x^{3} y - 99950 x^{3} - 140250 x^{2} y^{3} + 319275 x^{2} y^{2} - 234795 x^{2} y + 55588 x^{2} - 41250 x y^{4} + 134200 x y^{3} - 156420 x y^{2} + 77478 x y - 13744 x + 5500 y^{4} - 15400 y^{3} + 15620 y^{2} - 6776 y + 1056\right)}{1056}\)
This DOF is associated with edge 1 of the reference cell.
\(\displaystyle \phi_{7} = \frac{25 y \left(- 26250 x^{5} - 112875 x^{4} y + 83300 x^{4} - 185625 x^{3} y^{2} + 276500 x^{3} y - 99950 x^{3} - 140250 x^{2} y^{3} + 319275 x^{2} y^{2} - 234795 x^{2} y + 55588 x^{2} - 41250 x y^{4} + 134200 x y^{3} - 156420 x y^{2} + 77478 x y - 13744 x + 5500 y^{4} - 15400 y^{3} + 15620 y^{2} - 6776 y + 1056\right)}{1056}\)
This DOF is associated with edge 1 of the reference cell.
\(\displaystyle l_{8}:v\mapsto v(0,\tfrac{2}{5})\)
\(\displaystyle \phi_{8} = \frac{25 y \left(13125 x^{5} + 99750 x^{4} y - 47425 x^{4} + 235125 x^{3} y^{2} - 276850 x^{3} y + 65925 x^{3} + 231000 x^{2} y^{3} - 456225 x^{2} y^{2} + 269500 x^{2} y - 43183 x^{2} + 82500 x y^{4} - 245300 x y^{3} + 251350 x y^{2} - 101868 x y + 12614 x - 11000 y^{4} + 28600 y^{3} - 25960 y^{2} + 9416 y - 1056\right)}{1056}\)
This DOF is associated with edge 1 of the reference cell.
\(\displaystyle \phi_{8} = \frac{25 y \left(13125 x^{5} + 99750 x^{4} y - 47425 x^{4} + 235125 x^{3} y^{2} - 276850 x^{3} y + 65925 x^{3} + 231000 x^{2} y^{3} - 456225 x^{2} y^{2} + 269500 x^{2} y - 43183 x^{2} + 82500 x y^{4} - 245300 x y^{3} + 251350 x y^{2} - 101868 x y + 12614 x - 11000 y^{4} + 28600 y^{3} - 25960 y^{2} + 9416 y - 1056\right)}{1056}\)
This DOF is associated with edge 1 of the reference cell.
\(\displaystyle l_{9}:v\mapsto v(0,\tfrac{3}{5})\)
\(\displaystyle \phi_{9} = \frac{25 y \left(- 7500 x^{5} - 44625 x^{4} y + 25450 x^{4} - 136125 x^{3} y^{2} + 138400 x^{3} y - 35000 x^{3} - 181500 x^{2} y^{3} + 311025 x^{2} y^{2} - 157025 x^{2} y + 24302 x^{2} - 82500 x y^{4} + 222200 x y^{3} - 200750 x y^{2} + 70062 x y - 7956 x + 11000 y^{4} - 26400 y^{3} + 21560 y^{2} - 6864 y + 704\right)}{1056}\)
This DOF is associated with edge 1 of the reference cell.
\(\displaystyle \phi_{9} = \frac{25 y \left(- 7500 x^{5} - 44625 x^{4} y + 25450 x^{4} - 136125 x^{3} y^{2} + 138400 x^{3} y - 35000 x^{3} - 181500 x^{2} y^{3} + 311025 x^{2} y^{2} - 157025 x^{2} y + 24302 x^{2} - 82500 x y^{4} + 222200 x y^{3} - 200750 x y^{2} + 70062 x y - 7956 x + 11000 y^{4} - 26400 y^{3} + 21560 y^{2} - 6864 y + 704\right)}{1056}\)
This DOF is associated with edge 1 of the reference cell.
\(\displaystyle l_{10}:v\mapsto v(0,\tfrac{4}{5})\)
\(\displaystyle \phi_{10} = \frac{25 y \left(2625 x^{5} + 15000 x^{4} y - 8825 x^{4} + 37125 x^{3} y^{2} - 42500 x^{3} y + 11645 x^{3} + 66000 x^{2} y^{3} - 101475 x^{2} y^{2} + 50490 x^{2} y - 8047 x^{2} + 41250 x y^{4} - 99550 x y^{3} + 80520 x y^{2} - 26142 x y + 2866 x - 5500 y^{4} + 12100 y^{3} - 9020 y^{2} + 2684 y - 264\right)}{1056}\)
This DOF is associated with edge 1 of the reference cell.
\(\displaystyle \phi_{10} = \frac{25 y \left(2625 x^{5} + 15000 x^{4} y - 8825 x^{4} + 37125 x^{3} y^{2} - 42500 x^{3} y + 11645 x^{3} + 66000 x^{2} y^{3} - 101475 x^{2} y^{2} + 50490 x^{2} y - 8047 x^{2} + 41250 x y^{4} - 99550 x y^{3} + 80520 x y^{2} - 26142 x y + 2866 x - 5500 y^{4} + 12100 y^{3} - 9020 y^{2} + 2684 y - 264\right)}{1056}\)
This DOF is associated with edge 1 of the reference cell.
\(\displaystyle l_{11}:v\mapsto v(\tfrac{1}{5},0)\)
\(\displaystyle \phi_{11} = \frac{25 x \left(- 41250 x^{4} y + 5500 x^{4} - 140250 x^{3} y^{2} + 134200 x^{3} y - 15400 x^{3} - 185625 x^{2} y^{3} + 319275 x^{2} y^{2} - 156420 x^{2} y + 15620 x^{2} - 112875 x y^{4} + 276500 x y^{3} - 234795 x y^{2} + 77478 x y - 6776 x - 26250 y^{5} + 83300 y^{4} - 99950 y^{3} + 55588 y^{2} - 13744 y + 1056\right)}{1056}\)
This DOF is associated with edge 2 of the reference cell.
\(\displaystyle \phi_{11} = \frac{25 x \left(- 41250 x^{4} y + 5500 x^{4} - 140250 x^{3} y^{2} + 134200 x^{3} y - 15400 x^{3} - 185625 x^{2} y^{3} + 319275 x^{2} y^{2} - 156420 x^{2} y + 15620 x^{2} - 112875 x y^{4} + 276500 x y^{3} - 234795 x y^{2} + 77478 x y - 6776 x - 26250 y^{5} + 83300 y^{4} - 99950 y^{3} + 55588 y^{2} - 13744 y + 1056\right)}{1056}\)
This DOF is associated with edge 2 of the reference cell.
\(\displaystyle l_{12}:v\mapsto v(\tfrac{2}{5},0)\)
\(\displaystyle \phi_{12} = \frac{25 x \left(82500 x^{4} y - 11000 x^{4} + 231000 x^{3} y^{2} - 245300 x^{3} y + 28600 x^{3} + 235125 x^{2} y^{3} - 456225 x^{2} y^{2} + 251350 x^{2} y - 25960 x^{2} + 99750 x y^{4} - 276850 x y^{3} + 269500 x y^{2} - 101868 x y + 9416 x + 13125 y^{5} - 47425 y^{4} + 65925 y^{3} - 43183 y^{2} + 12614 y - 1056\right)}{1056}\)
This DOF is associated with edge 2 of the reference cell.
\(\displaystyle \phi_{12} = \frac{25 x \left(82500 x^{4} y - 11000 x^{4} + 231000 x^{3} y^{2} - 245300 x^{3} y + 28600 x^{3} + 235125 x^{2} y^{3} - 456225 x^{2} y^{2} + 251350 x^{2} y - 25960 x^{2} + 99750 x y^{4} - 276850 x y^{3} + 269500 x y^{2} - 101868 x y + 9416 x + 13125 y^{5} - 47425 y^{4} + 65925 y^{3} - 43183 y^{2} + 12614 y - 1056\right)}{1056}\)
This DOF is associated with edge 2 of the reference cell.
\(\displaystyle l_{13}:v\mapsto v(\tfrac{3}{5},0)\)
\(\displaystyle \phi_{13} = \frac{25 x \left(- 82500 x^{4} y + 11000 x^{4} - 181500 x^{3} y^{2} + 222200 x^{3} y - 26400 x^{3} - 136125 x^{2} y^{3} + 311025 x^{2} y^{2} - 200750 x^{2} y + 21560 x^{2} - 44625 x y^{4} + 138400 x y^{3} - 157025 x y^{2} + 70062 x y - 6864 x - 7500 y^{5} + 25450 y^{4} - 35000 y^{3} + 24302 y^{2} - 7956 y + 704\right)}{1056}\)
This DOF is associated with edge 2 of the reference cell.
\(\displaystyle \phi_{13} = \frac{25 x \left(- 82500 x^{4} y + 11000 x^{4} - 181500 x^{3} y^{2} + 222200 x^{3} y - 26400 x^{3} - 136125 x^{2} y^{3} + 311025 x^{2} y^{2} - 200750 x^{2} y + 21560 x^{2} - 44625 x y^{4} + 138400 x y^{3} - 157025 x y^{2} + 70062 x y - 6864 x - 7500 y^{5} + 25450 y^{4} - 35000 y^{3} + 24302 y^{2} - 7956 y + 704\right)}{1056}\)
This DOF is associated with edge 2 of the reference cell.
\(\displaystyle l_{14}:v\mapsto v(\tfrac{4}{5},0)\)
\(\displaystyle \phi_{14} = \frac{25 x \left(41250 x^{4} y - 5500 x^{4} + 66000 x^{3} y^{2} - 99550 x^{3} y + 12100 x^{3} + 37125 x^{2} y^{3} - 101475 x^{2} y^{2} + 80520 x^{2} y - 9020 x^{2} + 15000 x y^{4} - 42500 x y^{3} + 50490 x y^{2} - 26142 x y + 2684 x + 2625 y^{5} - 8825 y^{4} + 11645 y^{3} - 8047 y^{2} + 2866 y - 264\right)}{1056}\)
This DOF is associated with edge 2 of the reference cell.
\(\displaystyle \phi_{14} = \frac{25 x \left(41250 x^{4} y - 5500 x^{4} + 66000 x^{3} y^{2} - 99550 x^{3} y + 12100 x^{3} + 37125 x^{2} y^{3} - 101475 x^{2} y^{2} + 80520 x^{2} y - 9020 x^{2} + 15000 x y^{4} - 42500 x y^{3} + 50490 x y^{2} - 26142 x y + 2684 x + 2625 y^{5} - 8825 y^{4} + 11645 y^{3} - 8047 y^{2} + 2866 y - 264\right)}{1056}\)
This DOF is associated with edge 2 of the reference cell.
\(\displaystyle l_{15}:v\mapsto v(\tfrac{2}{15},\tfrac{2}{15})\)
\(\displaystyle \phi_{15} = \frac{625 x y \left(675 x^{4} + 2700 x^{3} y - 2025 x^{3} + 4050 x^{2} y^{2} - 6075 x^{2} y + 2223 x^{2} + 2700 x y^{3} - 6075 x y^{2} + 4446 x y - 1055 x + 675 y^{4} - 2025 y^{3} + 2223 y^{2} - 1055 y + 182\right)}{264}\)
This DOF is associated with face 0 of the reference cell.
\(\displaystyle \phi_{15} = \frac{625 x y \left(675 x^{4} + 2700 x^{3} y - 2025 x^{3} + 4050 x^{2} y^{2} - 6075 x^{2} y + 2223 x^{2} + 2700 x y^{3} - 6075 x y^{2} + 4446 x y - 1055 x + 675 y^{4} - 2025 y^{3} + 2223 y^{2} - 1055 y + 182\right)}{264}\)
This DOF is associated with face 0 of the reference cell.
\(\displaystyle l_{16}:v\mapsto v(\tfrac{2}{15},\tfrac{1}{3})\)
\(\displaystyle \phi_{16} = \frac{125 x y \left(- 675 x^{3} y + 90 x^{3} - 2025 x^{2} y^{2} + 1980 x^{2} y - 228 x^{2} - 2025 x y^{3} + 3690 x y^{2} - 1881 x y + 190 x - 675 y^{4} + 1800 y^{3} - 1653 y^{2} + 580 y - 52\right)}{32}\)
This DOF is associated with face 0 of the reference cell.
\(\displaystyle \phi_{16} = \frac{125 x y \left(- 675 x^{3} y + 90 x^{3} - 2025 x^{2} y^{2} + 1980 x^{2} y - 228 x^{2} - 2025 x y^{3} + 3690 x y^{2} - 1881 x y + 190 x - 675 y^{4} + 1800 y^{3} - 1653 y^{2} + 580 y - 52\right)}{32}\)
This DOF is associated with face 0 of the reference cell.
\(\displaystyle l_{17}:v\mapsto v(\tfrac{2}{15},\tfrac{8}{15})\)
\(\displaystyle \phi_{17} = \frac{125 x y \left(675 x^{2} y^{2} - 315 x^{2} y + 30 x^{2} + 1350 x y^{3} - 1890 x y^{2} + 648 x y - 56 x + 675 y^{4} - 1575 y^{3} + 1203 y^{2} - 329 y + 26\right)}{32}\)
This DOF is associated with face 0 of the reference cell.
\(\displaystyle \phi_{17} = \frac{125 x y \left(675 x^{2} y^{2} - 315 x^{2} y + 30 x^{2} + 1350 x y^{3} - 1890 x y^{2} + 648 x y - 56 x + 675 y^{4} - 1575 y^{3} + 1203 y^{2} - 329 y + 26\right)}{32}\)
This DOF is associated with face 0 of the reference cell.
\(\displaystyle l_{18}:v\mapsto v(\tfrac{2}{15},\tfrac{11}{15})\)
\(\displaystyle \phi_{18} = \frac{625 x y \left(- 675 x y^{3} + 675 x y^{2} - 198 x y + 16 x - 675 y^{4} + 1350 y^{3} - 873 y^{2} + 214 y - 16\right)}{264}\)
This DOF is associated with face 0 of the reference cell.
\(\displaystyle \phi_{18} = \frac{625 x y \left(- 675 x y^{3} + 675 x y^{2} - 198 x y + 16 x - 675 y^{4} + 1350 y^{3} - 873 y^{2} + 214 y - 16\right)}{264}\)
This DOF is associated with face 0 of the reference cell.
\(\displaystyle l_{19}:v\mapsto v(\tfrac{1}{3},\tfrac{2}{15})\)
\(\displaystyle \phi_{19} = \frac{125 x y \left(- 675 x^{4} - 2025 x^{3} y + 1800 x^{3} - 2025 x^{2} y^{2} + 3690 x^{2} y - 1653 x^{2} - 675 x y^{3} + 1980 x y^{2} - 1881 x y + 580 x + 90 y^{3} - 228 y^{2} + 190 y - 52\right)}{32}\)
This DOF is associated with face 0 of the reference cell.
\(\displaystyle \phi_{19} = \frac{125 x y \left(- 675 x^{4} - 2025 x^{3} y + 1800 x^{3} - 2025 x^{2} y^{2} + 3690 x^{2} y - 1653 x^{2} - 675 x y^{3} + 1980 x y^{2} - 1881 x y + 580 x + 90 y^{3} - 228 y^{2} + 190 y - 52\right)}{32}\)
This DOF is associated with face 0 of the reference cell.
\(\displaystyle l_{20}:v\mapsto v(\tfrac{1}{3},\tfrac{1}{3})\)
\(\displaystyle \phi_{20} = x y \left(3375 x^{3} y - 450 x^{3} + 6750 x^{2} y^{2} - 7650 x^{2} y + 900 x^{2} + 3375 x y^{3} - 7650 x y^{2} + 4725 x y - 502 x - 450 y^{3} + 900 y^{2} - 502 y + 52\right)\)
This DOF is associated with face 0 of the reference cell.
\(\displaystyle \phi_{20} = x y \left(3375 x^{3} y - 450 x^{3} + 6750 x^{2} y^{2} - 7650 x^{2} y + 900 x^{2} + 3375 x y^{3} - 7650 x y^{2} + 4725 x y - 502 x - 450 y^{3} + 900 y^{2} - 502 y + 52\right)\)
This DOF is associated with face 0 of the reference cell.
\(\displaystyle l_{21}:v\mapsto v(\tfrac{1}{3},\tfrac{8}{15})\)
\(\displaystyle \phi_{21} = \frac{125 x y \left(- 675 x^{2} y^{2} + 315 x^{2} y - 30 x^{2} - 675 x y^{3} + 1080 x y^{2} - 387 x y + 34 x + 90 y^{3} - 132 y^{2} + 46 y - 4\right)}{32}\)
This DOF is associated with face 0 of the reference cell.
\(\displaystyle \phi_{21} = \frac{125 x y \left(- 675 x^{2} y^{2} + 315 x^{2} y - 30 x^{2} - 675 x y^{3} + 1080 x y^{2} - 387 x y + 34 x + 90 y^{3} - 132 y^{2} + 46 y - 4\right)}{32}\)
This DOF is associated with face 0 of the reference cell.
\(\displaystyle l_{22}:v\mapsto v(\tfrac{8}{15},\tfrac{2}{15})\)
\(\displaystyle \phi_{22} = \frac{125 x y \left(675 x^{4} + 1350 x^{3} y - 1575 x^{3} + 675 x^{2} y^{2} - 1890 x^{2} y + 1203 x^{2} - 315 x y^{2} + 648 x y - 329 x + 30 y^{2} - 56 y + 26\right)}{32}\)
This DOF is associated with face 0 of the reference cell.
\(\displaystyle \phi_{22} = \frac{125 x y \left(675 x^{4} + 1350 x^{3} y - 1575 x^{3} + 675 x^{2} y^{2} - 1890 x^{2} y + 1203 x^{2} - 315 x y^{2} + 648 x y - 329 x + 30 y^{2} - 56 y + 26\right)}{32}\)
This DOF is associated with face 0 of the reference cell.
\(\displaystyle l_{23}:v\mapsto v(\tfrac{8}{15},\tfrac{1}{3})\)
\(\displaystyle \phi_{23} = \frac{125 x y \left(- 675 x^{3} y + 90 x^{3} - 675 x^{2} y^{2} + 1080 x^{2} y - 132 x^{2} + 315 x y^{2} - 387 x y + 46 x - 30 y^{2} + 34 y - 4\right)}{32}\)
This DOF is associated with face 0 of the reference cell.
\(\displaystyle \phi_{23} = \frac{125 x y \left(- 675 x^{3} y + 90 x^{3} - 675 x^{2} y^{2} + 1080 x^{2} y - 132 x^{2} + 315 x y^{2} - 387 x y + 46 x - 30 y^{2} + 34 y - 4\right)}{32}\)
This DOF is associated with face 0 of the reference cell.