Serendipity

Click here to read what the information on this page means.

De Rham complex families	\(\left[S_{1,k}^\square\right]_{0}\) or \(\mathcal{S}_{k}\Lambda^{0}(\square_d)\), \(\left[S_{2,k-d}^\square\right]_{0}\) or \(\mathcal{S}^-_{k-d}\Lambda^{0}(\square_d)\)
Abbreviated names	S
Degrees	\(1\leqslant k\) where \(k\) is the Lagrange superdegree
Polynomial subdegree	\(k\)
Polynomial superdegree	\(k + d - 1\)
Lagrange subdegree	\(\max(1,\operatorname{floor}(k/d))\)
Lagrange superdegree	\(k\)
Reference elements	interval, quadrilateral, hexahedron
Polynomial set	\(\mathcal{P}_{k} \oplus \mathcal{X}_{k}\) ↓ Show polynomial set definitions ↓↑ Hide polynomial set definitions ↑ \(\mathcal{P}_k=\operatorname{span}\left\{\prod_{i=1}^dx_i^{p_i}\middle\|\sum_{i=1}^dp_i\leqslant k\right\}\) \(\mathcal{X}_k=\operatorname{span}\left\{\prod_{i=1}^dx_i^{p_i}\middle\|k<\sum_{i=1}^da_i\leqslant k+\#\left\{i\in\{1,\dots,d\}\middle\| a_i=1\right\}\right\}\)
DOFs	On each vertex: point evaluations On each edge: integral moments with an degree \(k-2\) dPc space On each face: integral moments with an degree \(k-4\) dPc space On each volume: integral moments with an degree \(k-6\) dPc space
Number of DOFs	interval: \(k+1\) (A000027) quadrilateral: \(\begin{cases}4&k=1\\k(k+3)/2+3&k>1\end{cases}\) (A340266) hexahedron: \(\begin{cases}12k-4&k=1,2,3\\3k^2-3k+14&k=4,5\\k(k-1)(k+1)/6+k^2+5k+4&k>6\end{cases}\)
Mapping	identity
continuity	Function values are continuous.
Categories	Scalar-valued elements

Implementations

This element is implemented in Basix , Basix.UFL , FIAT , Symfem , and (legacy) UFL.↓ Show implementation detail ↓

↑ Hide implementation detail ↑

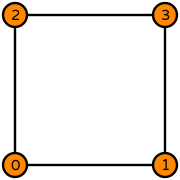
Basix	`basix.ElementFamily.serendipity, lagrange_variant=basix.LagrangeVariant.equispaced, dpc_variant=basix.DPCVariant.simplex_equispaced` ↓ Show Basix examples ↓↑ Hide Basix examples ↑ Before running this example, you must install Basix: pip3 install fenics-basix This element can then be created with the following lines of Python: import basix # Create serendipity degree 1 on a interval element = basix.create_element(basix.ElementFamily.serendipity, basix.CellType.interval, 1, lagrange_variant=basix.LagrangeVariant.equispaced, dpc_variant=basix.DPCVariant.simplex_equispaced) # 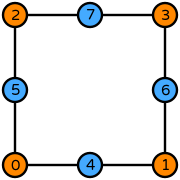Create serendipity degree 2 on a interval element = basix.create_element(basix.ElementFamily.serendipity, basix.CellType.interval, 2, lagrange_variant=basix.LagrangeVariant.equispaced, dpc_variant=basix.DPCVariant.simplex_equispaced) # 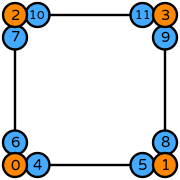Create serendipity degree 3 on a interval element = basix.create_element(basix.ElementFamily.serendipity, basix.CellType.interval, 3, lagrange_variant=basix.LagrangeVariant.equispaced, dpc_variant=basix.DPCVariant.simplex_equispaced) # Create serendipity degree 1 on a quadrilateral element = basix.create_element(basix.ElementFamily.serendipity, basix.CellType.quadrilateral, 1, lagrange_variant=basix.LagrangeVariant.equispaced, dpc_variant=basix.DPCVariant.simplex_equispaced) # Create serendipity degree 2 on a quadrilateral element = basix.create_element(basix.ElementFamily.serendipity, basix.CellType.quadrilateral, 2, lagrange_variant=basix.LagrangeVariant.equispaced, dpc_variant=basix.DPCVariant.simplex_equispaced) # Create serendipity degree 3 on a quadrilateral element = basix.create_element(basix.ElementFamily.serendipity, basix.CellType.quadrilateral, 3, lagrange_variant=basix.LagrangeVariant.equispaced, dpc_variant=basix.DPCVariant.simplex_equispaced) This implementation is correct for all the examples below.
Basix.UFL	`basix.ElementFamily.serendipity, lagrange_variant=basix.LagrangeVariant.equispaced, dpc_variant=basix.DPCVariant.simplex_equispaced` ↓ Show Basix.UFL examples ↓↑ Hide Basix.UFL examples ↑ Before running this example, you must install Basix.UFL: pip3 install fenics-basix fenics-ufl This element can then be created with the following lines of Python: import basix import basix.ufl # Create serendipity degree 1 on a interval element = basix.ufl.element(basix.ElementFamily.serendipity, basix.CellType.interval, 1, lagrange_variant=basix.LagrangeVariant.equispaced, dpc_variant=basix.DPCVariant.simplex_equispaced) # Create serendipity degree 2 on a interval element = basix.ufl.element(basix.ElementFamily.serendipity, basix.CellType.interval, 2, lagrange_variant=basix.LagrangeVariant.equispaced, dpc_variant=basix.DPCVariant.simplex_equispaced) # Create serendipity degree 3 on a interval element = basix.ufl.element(basix.ElementFamily.serendipity, basix.CellType.interval, 3, lagrange_variant=basix.LagrangeVariant.equispaced, dpc_variant=basix.DPCVariant.simplex_equispaced) # Create serendipity degree 1 on a quadrilateral element = basix.ufl.element(basix.ElementFamily.serendipity, basix.CellType.quadrilateral, 1, lagrange_variant=basix.LagrangeVariant.equispaced, dpc_variant=basix.DPCVariant.simplex_equispaced) # Create serendipity degree 2 on a quadrilateral element = basix.ufl.element(basix.ElementFamily.serendipity, basix.CellType.quadrilateral, 2, lagrange_variant=basix.LagrangeVariant.equispaced, dpc_variant=basix.DPCVariant.simplex_equispaced) # Create serendipity degree 3 on a quadrilateral element = basix.ufl.element(basix.ElementFamily.serendipity, basix.CellType.quadrilateral, 3, lagrange_variant=basix.LagrangeVariant.equispaced, dpc_variant=basix.DPCVariant.simplex_equispaced) This implementation is correct for all the examples below.
FIAT	`FIAT.Serendipity` ↓ Show FIAT examples ↓↑ Hide FIAT examples ↑ Before running this example, you must install FIAT: pip3 install git+https://github.com/firedrakeproject/fiat.git This element can then be created with the following lines of Python: import FIAT # Create serendipity degree 1 element = FIAT.Serendipity(FIAT.ufc_cell("interval"), 1) # Create serendipity degree 2 element = FIAT.Serendipity(FIAT.ufc_cell("interval"), 2) # Create serendipity degree 3 element = FIAT.Serendipity(FIAT.ufc_cell("interval"), 3) # Create serendipity degree 1 element = FIAT.Serendipity(FIAT.reference_element.UFCQuadrilateral(), 1) # Create serendipity degree 2 element = FIAT.Serendipity(FIAT.reference_element.UFCQuadrilateral(), 2) # Create serendipity degree 3 element = FIAT.Serendipity(FIAT.reference_element.UFCQuadrilateral(), 3) This implementation is correct for all the examples below.
Symfem	`"serendipity"` ↓ Show Symfem examples ↓↑ Hide Symfem examples ↑ Before running this example, you must install Symfem: pip3 install symfem This element can then be created with the following lines of Python: import symfem # Create serendipity degree 1 on a interval element = symfem.create_element("interval", "serendipity", 1) # Create serendipity degree 2 on a interval element = symfem.create_element("interval", "serendipity", 2) # Create serendipity degree 3 on a interval element = symfem.create_element("interval", "serendipity", 3) # Create serendipity degree 1 on a quadrilateral element = symfem.create_element("quadrilateral", "serendipity", 1) # Create serendipity degree 2 on a quadrilateral element = symfem.create_element("quadrilateral", "serendipity", 2) # Create serendipity degree 3 on a quadrilateral element = symfem.create_element("quadrilateral", "serendipity", 3) This implementation is used to compute the examples below and verify other implementations.
(legacy) UFL	`"S"` ↓ Show (legacy) UFL examples ↓↑ Hide (legacy) UFL examples ↑ Before running this example, you must install (legacy) UFL: pip3 install setuptools pip3 install fenics-ufl-legacy This element can then be created with the following lines of Python: import ufl_legacy # Create serendipity degree 1 on a interval element = ufl_legacy.FiniteElement("S", "interval", 1) # Create serendipity degree 2 on a interval element = ufl_legacy.FiniteElement("S", "interval", 2) # Create serendipity degree 3 on a interval element = ufl_legacy.FiniteElement("S", "interval", 3) # Create serendipity degree 1 on a quadrilateral element = ufl_legacy.FiniteElement("S", "quadrilateral", 1) # Create serendipity degree 2 on a quadrilateral element = ufl_legacy.FiniteElement("S", "quadrilateral", 2) # Create serendipity degree 3 on a quadrilateral element = ufl_legacy.FiniteElement("S", "quadrilateral", 3)

Examples

interval degree 1	(click to view basis functions)
interval degree 2	(click to view basis functions)
interval degree 3	(click to view basis functions)
quadrilateral degree 1	(click to view basis functions)
quadrilateral degree 2	(click to view basis functions)
quadrilateral degree 3	(click to view basis functions)

References

Arnold, Douglas N. and Awanou, Gerard. The serendipity family of finite elements, Foundations of Computational Mathematics 11(3), 337–344, 2011. [DOI: 10.1007/s10208-011-9087-3] [BibTeX]
Arnold, Douglas N. and Logg, Anders. Periodic table of the finite elements, SIAM News 47, 2014. [www.siam.org/publications/siam-news/issues/volume-47-number-09-november-2014/] [BibTeX]
Cockburn, Bernardo and Fu, Guosheng. A systematic construction of finite element commuting exact sequences, SIAM journal on numerical analysis 55, 1650–1688, 2017. [DOI: 10.1137/16M1073352] [BibTeX]

DefElement stats

Element added	02 January 2021
Element last updated	27 September 2024